Reading: Chapter 3, pages 23-26.

Introductions

Course Home Page

Course Overview Course Learning Goals Course Information Course Outline

Course Questionaire

Introduction to Motion- Position, Velocity and Acceleration

For now, let's confine ourselves to motion in a straight line, or one-dimensional motion. Our system is some object in motion. We wish to:

• develop a mental picture of what's happening.
• determine the important descriptive parameters (variables).
• figure out a relationship between those variables.
• test our relationship, fix it up if necessary.
• build up a grand "theory" of motion.

Let's say our object in motion is the instructor's private jet (I wish...), taking him home after a hard day of teaching. How do we describe the instructors position at a given time after leaving UO?

Distances & displacements or, "where's Crow, Oregon?"

• 

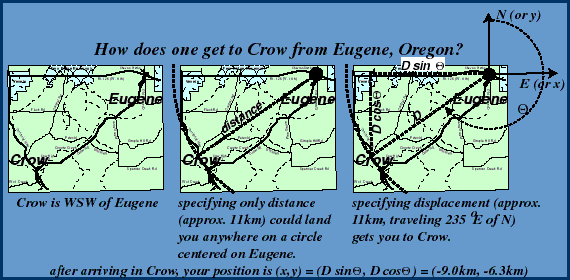
  So our list of important variables now includes displacement and time. We also would like to know about velocity. Let's speculate that:

  Velocity-- change in position (or displacement) over time (which wounds all heels?).

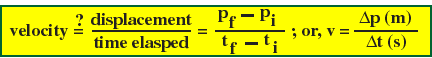
We can test our hypothetical relationship between velocity, position and time by making, matching and interpreting graphs of position vs. time.

• Make a graph using a motion detector and the computer, and ourselves as moving objects (see above).
• How does one move to match the position-time graph given on the computer display?
• What can we say about the motion associated with different segments of our position-time graphs?
• Do our experiments verify the above relationship between velocity, position and time?

Definition: average velocity is the change in x-position, Dx, over the change in time, Dt.

 

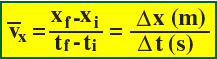
A refinement of the relationship: Speed and velocity-- what's the difference?

• 

   

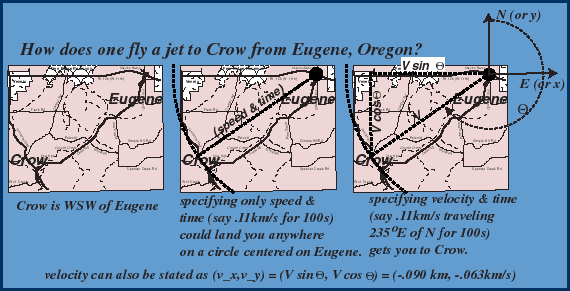
  To carefully specify velocity, we must keep track of the change in position in three dimensions (Dx, Dy & Dz). We can then calculate the three velocities, (v_x, v_y, v_z). This gives a complete description of our velocity. It can alternately be stated as a magnitude of velocity (speed) and direction (see above). Regardless, our definition of velocity related to change in position and time makes sense.

   

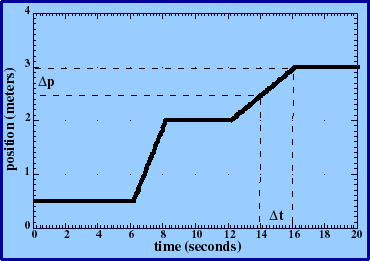
  Test our understanding- how do we read velocity from position vs. time graphs?

• 

  •  

  Reading velocity from velocity vs. time graphs.

  Moving to match velocity vs. time graphs.

  What about acceleration? We define it to be related to velocity vs. time graphs.

• 

  Definition: average acceleration is the change in velocity over the time elapsed.

Previous Lecture Next Lecture Back to Course Page.