World Views of the Universe

Reading: Chapter 26, Cosmology

Cosmological Facts

Expansion of the Universe
Cosmic Microwave Background (CMBR)
Hydrogen/Helium abundances

mass of the Universe
matter/anti-matter asymmetry
large scale structure of the Universe
Cosmological constant
....

The primary observational facts are the first three results. The other results will be discussed when appropriate. Before we move on to the main topics, let us consider something known as Olbers's Paradox

Models of the Universe

Based on Hubble's law and the CMBR, our world view of the Universe is rather firm. On the assumption that Hubble's Law, v = H_o x D, is due to an uniform expansion of the Universe, models have been constructed based on Einstein's General Theory of Relativity (GTR). Einstein himself made models for the Universe (circa 1910-1920's). Unfortunately, he erred because he believed in an homogeneous, isotropic, and static Universe (on philosphical grounds).

In a gravitating Universe, a static solution is not possible because gravity is always attractive ---> it always tries to pull things closer together
To get around this problem, Einstein postulated the existence of a large-scale repulsive force which could counteract gravity
This force is parameterized by what is referred to as The Cosmological Constant (represented by the Greek letter capital Lambda)

When Hubble's result became known (1929), Einstein immediately withdrew his suggestion of a Cosmological Constant as there was no need for a large-scale repulsive force in a non-static Universe. Today, however, the idea of a Cosmological Constant has been revived. This does not mean we believe in steady-state universes though; the basic models for the Universe all consider models which are expanding. We will now consider the properties of the proposed models.

Plan
I. I will first approach the Universe from a theoretical slan
II. I will next look at the observations relevant to theory
III. Finally, I will consider our place in the Universe

I. Modern View of the Universe

Types of Universes

After Einstein invented his General Theory of Relativity , Alexander Friedman developed the first models for the (spacetime) structure of universes based on Einstein's General Theory of Relativity (GTR). (Lemaitre also investigated models for universes using different assumptions.) Using GTR and assuming that the Cosmological Principle was valid for our Universe, Friedman found three general types of universes, flat, closed, and open each representing a different ultimate fate for the Universe.

Wait, recall that the Cosmological Principle says that the Universe is homogeneous and isotropic for any observer in the Universe. The appearance of the Universe can change with time, but all observers must see the same Universe for any given time.
How is this consistent with the observed structure in the Universe (structures almost a billion light years in size!)? Despite the impressive sizes of these features (e.g., the Voids, and the Sloan Great Wall mentioned in Topic 3), these structures are still less than 10 % of the distances to the farthest QSOs and thus fill less than 1 % of the volume of the Universe. The Universe appears fairly bland on scales larger than these observed features and the Cosmological Principle seems to hold for our Universe.

To get a feel for Friedman's models, we demonstrate some properties of Friedman universes using two-dimensional analogies.
Spacetime: Recall that in order to determine the locations of events in our Universe we must specify four things, the spatial location (where) and temporal location (when) of the event ===> we must consider our Universe to have four dimensions, the position and time of an event. These define the spacetime coordinates of an event. Note in our Universe although time is, in a sense, a simple coordinate equivalent to position, time occupies a preferred place in our modeling in that we are not able to move arbitrarily in time as we can through space.
Interestingly, the framework upon which we place these spacetime events for the Universe can have different shapes and that the shape is modified by the presence of mass (recall our discussion of black holes, and singularities). In terms of spacetime, the Friedman models are flat (Euclidean space, critical universe), postive curvature (e.g., a sphere, the closed universe), and negative curvature (e.g., a saddle, open universe) universes. In two-dimensions, positive and negative cruvature spacetimes look like

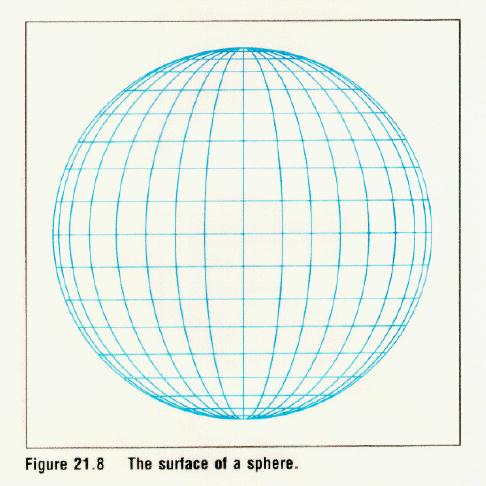
positive curvature

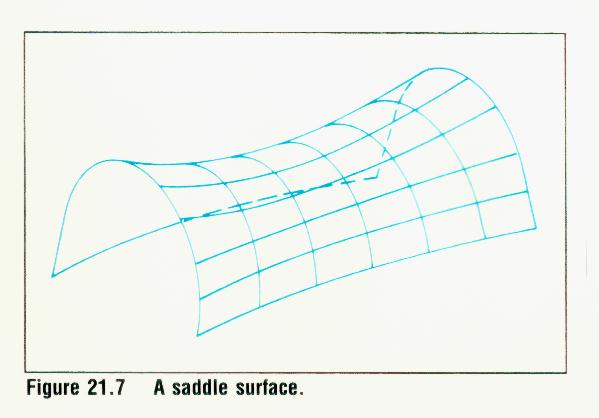
negative curvature

Abstract as these concepts are, these shapes (topologies) have important consequences for how our Universe evolves; the different shapes are indicative of the ultimate fate of the Universe. If we can determine the topology (geometry or shape) of the Universe, we can infer what is going to happen to our Universe in the distant future. In the coming weeks, we will explore how astronomers deduce the shape (topology) of our Universe and thus determine the ultimate fate of our Universe.

Properties of Geometric Objects in Different* Universes*
The geometry (curvature) of each type of universe is different; the spacetime of each universe has different character. A simple measurable difference between the universes can be found from proprties of different geometric shapes and features in each spacetime.
Flat universes ---> expanding universe and will stop expanding after an infinite amount of time; the flat universe is the dividing line between open and closed universes.

Closed universes ---> expanding, but will reach a maximum size and then collapse

Open universes ---> expanding universe which will expand forever and is infinite in spatial extent

Hubble Law and Topology of the Universe
How does the Hubble Law, that is, the relation between the redshift of and distance to far-away galaxies behave and how is it interpreted in the context of Einstein's GTR and the different Friedman models. The different Friedman solutions affect the Hubble Law at large distances primarily.

Hubble's Law In the context of Einstein's view of the universe,
How are Hubble's Law and galactic redshifts interpreted?
The galaxies and other denizens of the Universe are simply carried along by the expansion of the spacetime. In this sense, they may be stationary in the expanding Universe; the apparent recession of distant galaxies with respect to our home galaxy, the Milky Way, occurs because the distances of the galaxies from us, grow with time as the Universe grows in size! Each galaxy sees the other galaxies behave in the same manner. No one galaxy sits at the center of the universe, furthermore, there is no center of the expansion. This difficult conceptual point follows from the notion that the Universe itself is growing in size, it is not expanding into anything and so has no edges and therefore no center. All points in the Universe are simply moving apart with time.

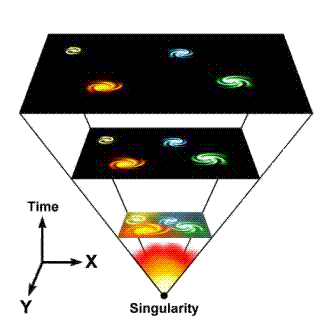Cosmological Redshifts Again, in an epxanding Universe, galaxies and the other denizens of the Universe are carried along by the expansion of the spacetime and, in this sense, are stationary. So, How Do Galactic Redshifts Arise? The apparent recession of distant galaxies with respect to our home galaxy, the Milky Way, occurs because the distances of the galaxies from us, grow with time as the Universe grows in size! In addition, the light in the Universe (see the inset to the right) also stretches as the Universe expands. In this manner, the expansion of the Universe redshifts the light from distant galaxies; the wavelength of the light when produced is smaller than today. Such redshifts caused by the expansion of the Universe are referred to as Cosmological Redshifts

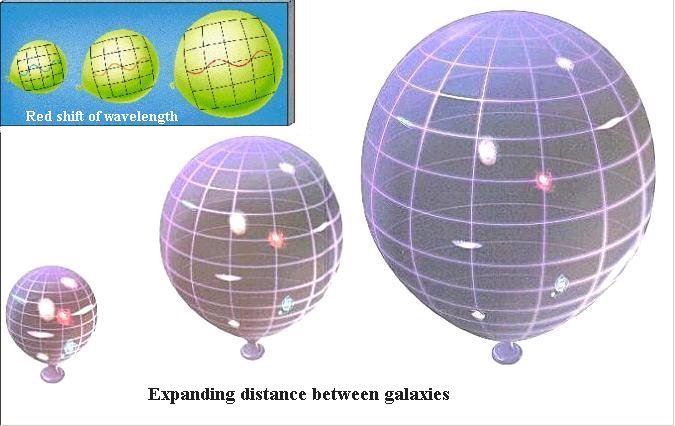Scale Factor, R(t)
Properties of the different spacetime solutions can also be readily visualized using what is referred to as the scale factor for the universe R(t). The scale factor, R(t), tells us how much bigger or smaller the Universe is today than it was yesterday and so on.

Scale Factor ===> Size = R(t) x Size (in the past) where Size (in the past) is usually the size of the universe at some point in the past.
In terms of the scale factor R(t), the evolution of the three solutions for the universe are shown to the right.
Closed universes correspond to the bottom curve. Open universes correspond to the top curve. Flat universes (critical universes) fall right between closed and open universes.

A great deal of effort is now directed toward determining which of the above models is the correct one for our Universe. The methods used to determine the correct universe model fall into two categories:

Dynamical Tests where we check to see if the Universe exceeds its escape speed by measuring the expansion rate of the Universe (the Hubble constant, H_o) and the mass of the Universe
Topological Tests where we try and measure the shape of the Universe
Tests where we match our most promising models to detailed observations of the Universe. In particular, we see which model can best explain the snapshot of the Universe taken at the Epoch of Recombination, that is, through analysis of the Cosmic Microwave Background Radiation (CMBR).

We will spend a fair amount of time on these methods (later). Let me first touch on some aspects of the second method. We consider results of other more definitive tests later.

Topological Tests for the Shape of the Universe
In principle, if we sat down and drew large triangles and measured their interior angles, we could determine the shape of the Universe. As a practical matter this is difficult. The other geometric properties of the Universe are also difficult to measure (as well). Are there other tests we can apply? Yes.

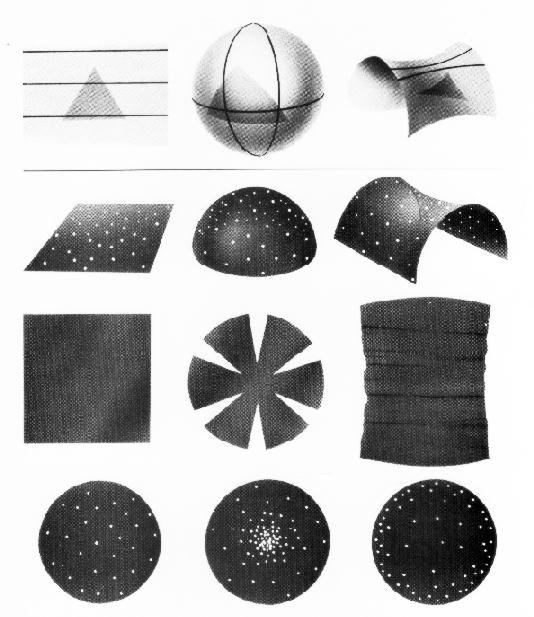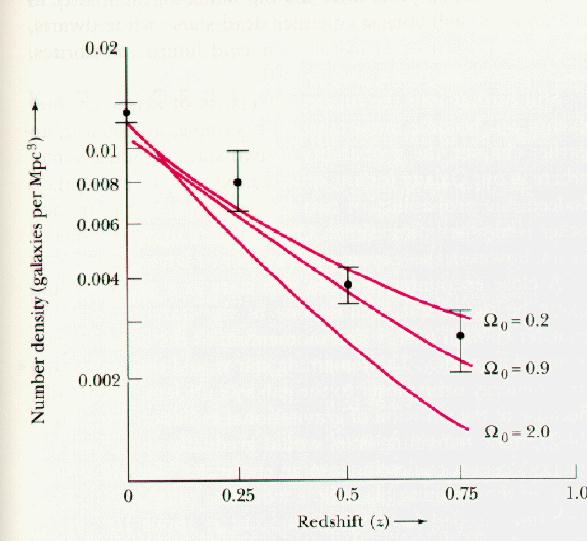
Angles, Areas, & Volumes
Depending upon the geometry of the Universe, the number density of galaxies at large redshift (===>large distance) should depend upon the geometry of the Universe. Why? Well, just as the area of a circle depends upon the geometry of the Universe, the volumes of objects may also depend upon the geometry of the Universe. The difference in the way volumes depend upon distance will affect the way densities depend upon distance. Tests for this effect have been carried out. The results are interesting, but not conclusive.

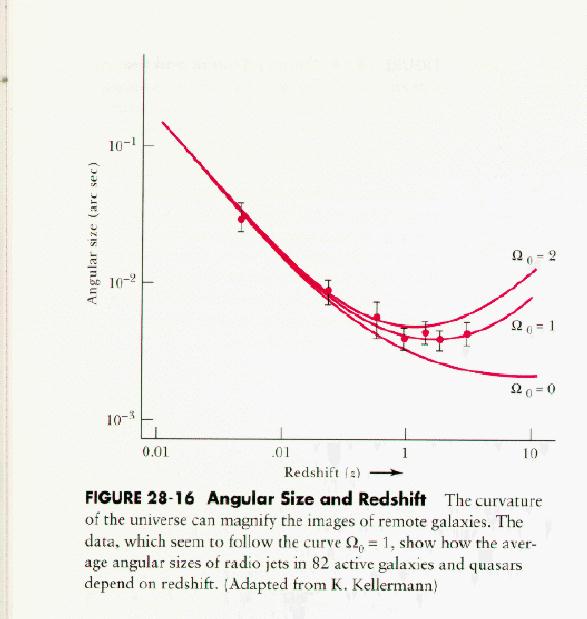
Parallel Lines
Depending upon the geometry of the Universe, the paths of parallel lines can cross or diverge, A consequence of this is that if measures the angular sizes of distant galaxies, they do necessarily have to decrease as 1/distance (as they would in a flat universe). This effect has also also been studied. The results are suggestive but, again, not conclusive.

Return to Home Page