 Nikolaus Copernicus |
Cosmological Principle |
 |
 Nikolaus Copernicus |
Cosmological Principle |
 |
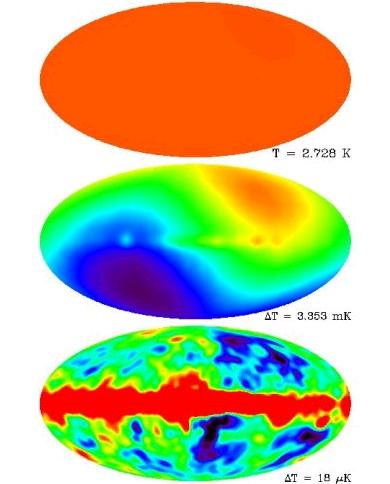
We do not occupy a preferred place in the Universe (Copernican belief). Further, at a given time, the Universe looks homogeneous and isotropic to all observers as is supported by the smoothness of the cosmic microwave background (CMB) radiation.
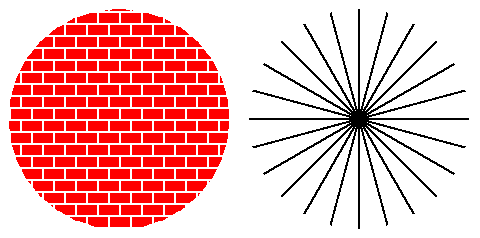 |
Homogeneous means that in an average sense, the Universe looks the same to all observers regardless of location. This is true for the left hand figure in the left panel (the brick wall) but not for the right hand figure in the left panel (the diverging lines from the center of the image).Isotropic means that the Universe appears the same in whatever direction one looks. If one is at the center of the figure on the right in the left hand panel, this is true and so the Universe appears isotropic for an observer at the center of this Universe. The figure on the left in the left hand panel does not appear isotropic for any observer! The suggestion that the Univere is Homogeneous and Isotropic is consistent with the smoothness of the CMB (more on this later).  The CMB is 2.7 Kelvin to within 0.003 Kelvin everywhere |
The Universe is homogeneous and isotropic on the scale of the CMB, but not on smaller scales such as this room, the Solar System, the Galaxy, and so on. We must specify the size of the region over which the Universe should be averaged. The scale over which the Universe should appear homogeneous and isotropic is not well-defined, outside of the requirement that it is large (e.g., see Abell 2218 , Hubble Deep Field Photograph Great Wall),
There is structure in the Universe, but it is small compared to the overall size of the Universe and so, in a sense, the imperfections can be averaged over ===> the Cosmologoical Principle is apparently valid on large scales.
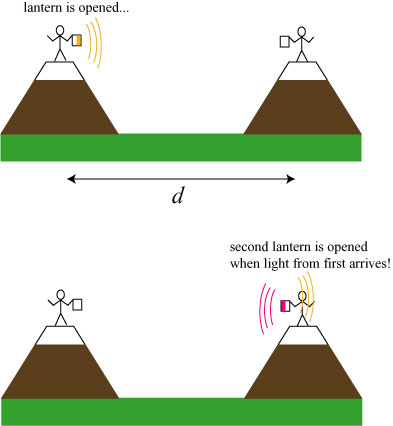
The homogeneous and isotropic requirements are tough. The CMB is homogeneous and isotropic which causes problems. The CMB from regions in the Universe which are in opposite directions are not causally connected and so really have no reason why they should have the same properties. Why?
 |
|  |
|
 |
Why is it perplexing that the CMB homogeneous and isotropic? When I look in one direction on the sky and observe the CMB, I see light that has traveled over the lifetime of the Universe to reach me. When I look behind me, in the opposite direction, I see light that has also traveled over the lifetime of the Universe to reach me. That is, the part of the Universe in front of me and the part behind me are so far apart that they have never been in thermal contact (and, in fact, in principle do not even know of the other's existence). Given this, how can they have the same temperature?