Distance Scale:

The most important value for an astronomical object is its distance from the Earth. Since cosmology deals with objects larger and brighter than our Sun or solar system, it is impossible to have the correct frame of reference with respect to their size and luminosity as there is nothing to compare extragalactic objects with.

Before the 1920's, it was thought that galaxies were in fact objects within our own Galaxy, possibly regions forming individual stars. They were given the name ``nebula'', which we now use to denote regions of gas and dust within galaxies.

At the turn of the century Cepheid variable stars, a special class of pulsating stars that exhibit a particular period-luminosity relation, were discovered. In other words, it was found that their intrinsic brightness was proportional to their period of variation and, hence, could be used for measuring the distances to nearby galaxies.

In the late 1920's, Hubble discovered similar Cepheid stars in neighboring galaxies as was found in our own Galaxy. Since they followed the same period-luminosity relation, and they were very faint, then this implied that the neighboring galaxies were very far away. This proved that spiral `nebula' were, in fact, external to our own Galaxy and sudden the Universe was vast in space and time.

Although Hubble showed that spiral nebula were external to our Galaxy, his estimate of their distances was off by a factor of 6. This was due to the fact that the calibration to Cepheids was poor at the time, combined with the primitive telescopes Hubble used.

Modern efforts to obtain an estimate of Hubble's constant, the expansion rate of the Universe, find it necessary to determine the distance and the velocities of a large sample of galaxies. The hardest step in this process is the construct of the distance scale for galaxies, a method of determining the true distance to a particular galaxy using some property or characteristic that is visible over a range of galaxies types and distance.

The determination of the distance scale begins with the construction of a ladder of primary, secondary and tertiary calibrators in the search for a standard candle.

Primary Calibrators:

The construction of the distance scale ladder is a process of building of a chain of objects with well determined distances. The bottom of this chain is the determination of the scale of objects in the Solar System. This is done through radar ranging, where a radio pulse is reflected off of the various planets in the Solar System.

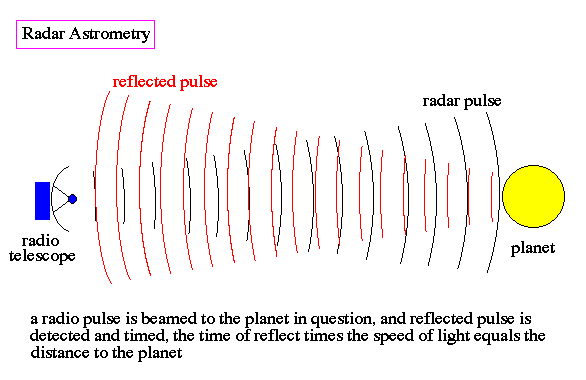
The most important value from solar system radar ranging is the exact distance of the Earth from the Sun, determined by triangular measurement of the Earth and terrestrial worlds. This allows an accurate value for what is called the Astronomical Unit (A.U.), i.e. the mean Earth-Sun distance. The A.U. is the ``yardstick'' for measuring the distance to nearby stars by parallax.

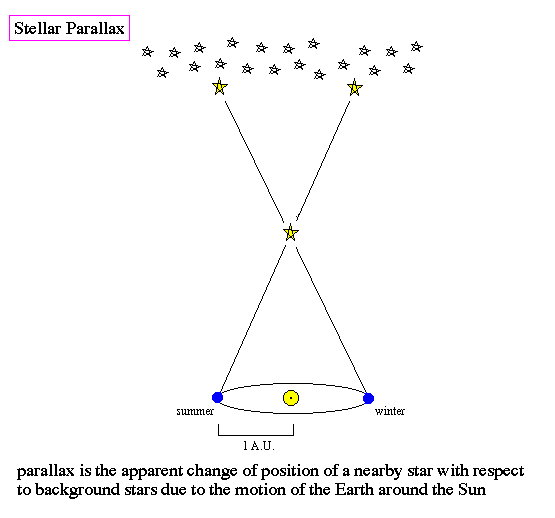
The parallax system is only good for stars within 300 light-years of the Earth due to limitations of measuring small changes in stellar position. Fortunately, there are hundreds of stars within this volume of space, which become the calibrators for secondary distance indicators.

Secondary Calibrators:

Secondary calibrators of the distance scale depend on statistical measures of stellar properties, such as the mean brightness of a class of stars. It has been known since the 1800's that stars follow a particular color-luminosity relation known as the Hertzsprung-Russell Diagram.

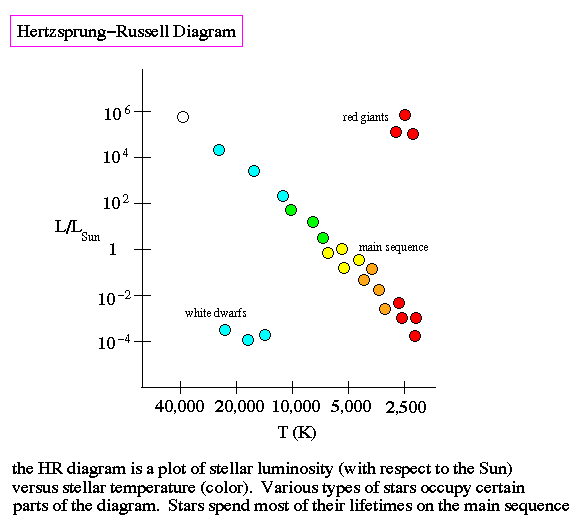
The existence of the main sequence for stars, a relationship between luminosity and color due to the stable, hydrogen-burning part of a star's life, allows for the use of spectroscopic parallax. A stars temperature is determined by its spectrum (some elements become ions at certain temperatures). With a known temperature, then an absolute luminosity can be read off the HR diagram.

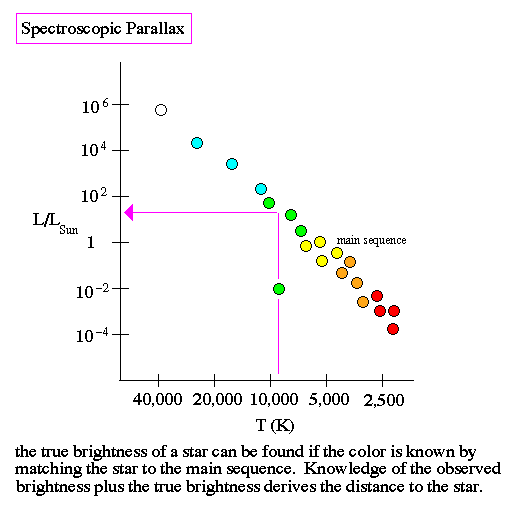
The distance to a star is simply the ratio of its apparent brightness and its true brightness (imagine car headlights at a distance). The method allows us to measure the distances to thousands of local stars and, in particular, to nearby star clusters which harbor variable stars.

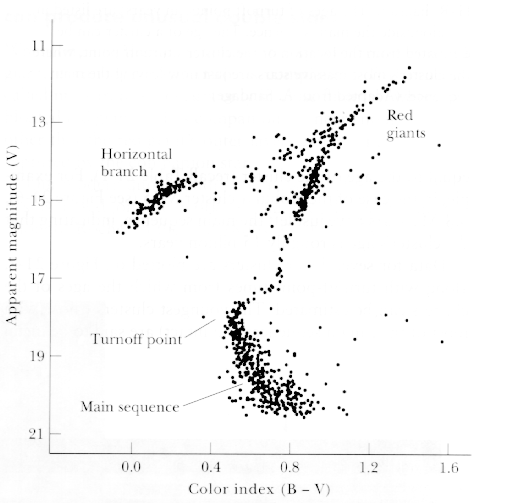
Above is an example of the color (i.e. temperature) versus luminosity of a star cluster. The distance to the cluster can be extracted by comparing the brightness of the main sequence to nearby stars.

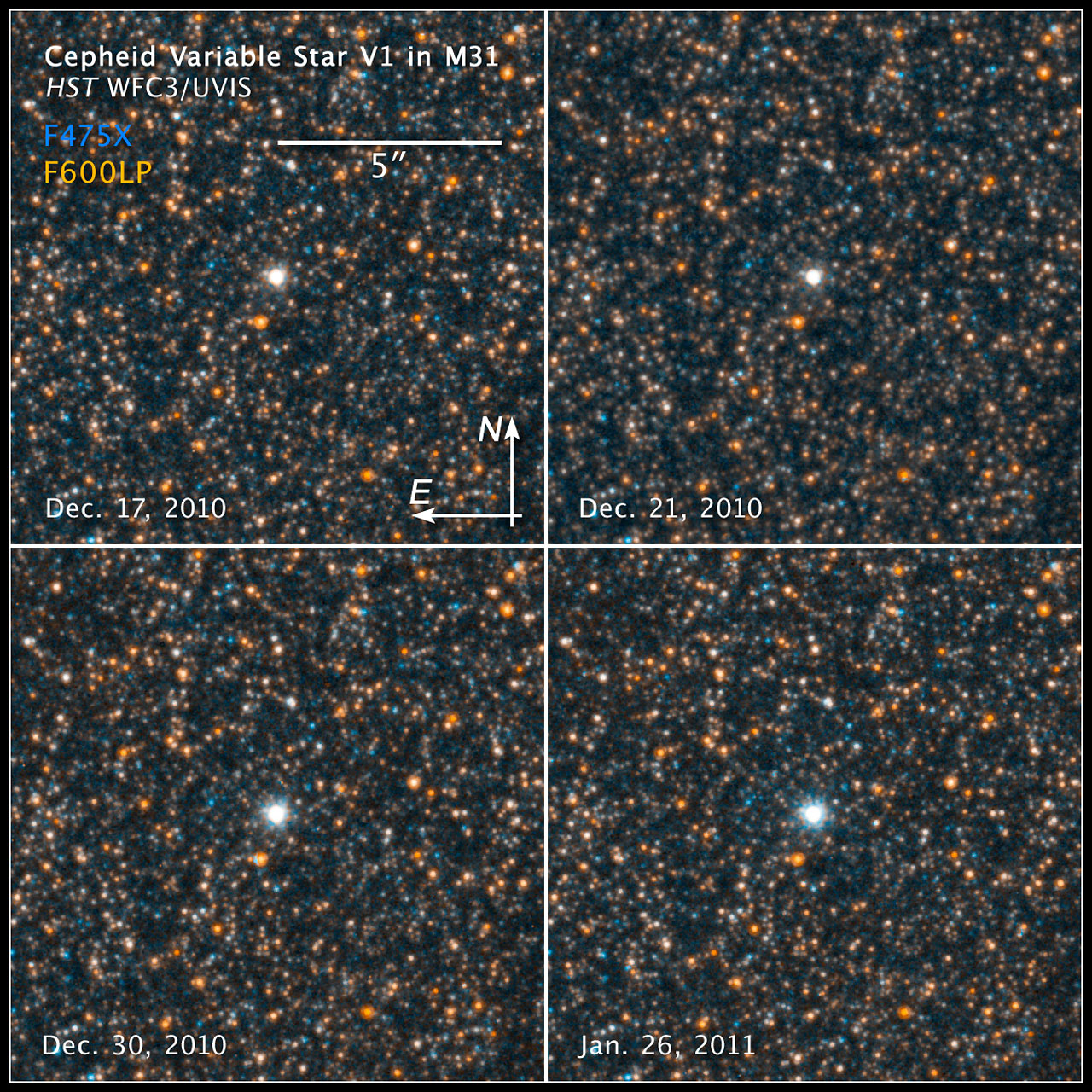
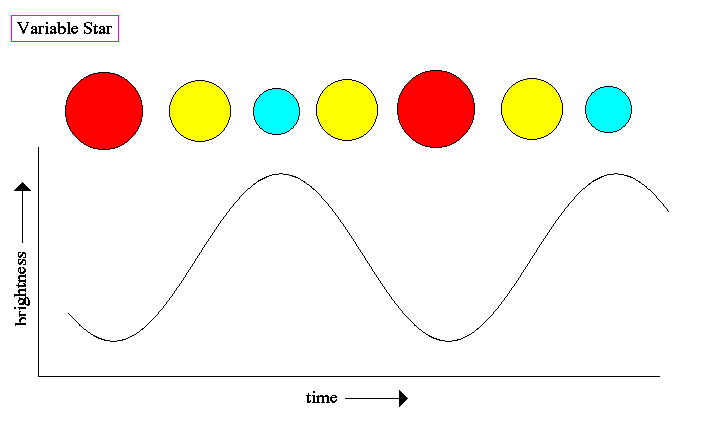
A variable star is a star where the brightness of the star changes over time (usually a small amount). This is traced by a light curve, a plot of brightness and time.

Particular variable stars, such as Cepheids, have a period-luminosity relationship. Meaning that for a particular period of oscillation, they have a unique absolute brightness.

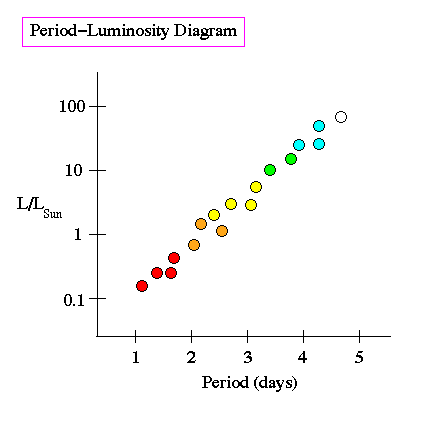
The result is that it is possible to measure the light curve of Cepheids in other galaxies and determine their distances.

Tertiary Calibrators:

The nearby region of the Universe, known as the Local Group and is located at the edge of what is known as the Virgo supercluster of galaxies. The use of Cepheid variables is limited to within the volume of space outlined by the Virgo system. Thus, the distances to nearby galaxies does not measure the true Hubble flow of the expanding Universe, but rather the gravitational infall into Virgo.

In order to determine Hubble's constant, we must measure the velocity of galaxies much farther away then the Local Group or the Virgo supercluster. But, at these distances we cannot see Cepheid stars, so we determine the total luminosity of the galaxy by the Tully-Fisher method, the last leg of the distance scale ladder.

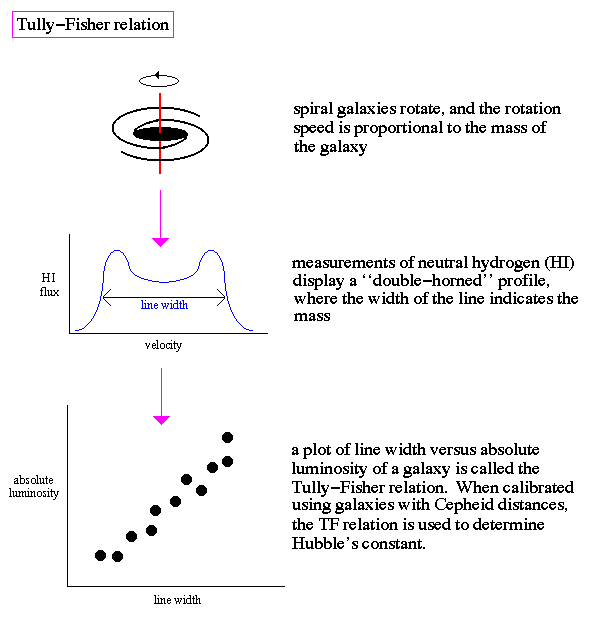
The Tully-Fisher relation is basically a plot of mass versus luminosity of a galaxy. Its not surprising that luminosity and mass are correlated since stars make up basically most of a galaxy's mass and all of the light. Missing mass would be in the form of gas, dust and dark matter.

The key parameter for this last leg of the distance scale are the calibrating galaxies to the Tully-Fisher relation, i.e. the galaxies where we know both the total luminosity from Cepheid distances and the total luminosity from the Tully-Fisher relation.

There is currently a strong debate on the value of the Hubble's constant fueled by new data from HST Cepheid studies of nearby galaxies. The community is divided into two schools of thought; 1) the old school which proposes a value for Hubble's constant around 50 to agree with the ages of the oldest stars in our Galaxy, and 2) a newer, and larger school which finds a higher Hubble's constant of 75. This higher value poses a problem for modern cosmology in that the age of the Universe from Hubble's constant is less than the age of the oldest stars as determined by nuclear physics.

So the dilemma is this, either something is wrong with nuclear physics or something is wrong with our understanding of the geometry of the Universe. One possible solution is the introduction of the cosmological constant, once rejected as unnecessary to cosmology, it has now grown in importance due to the conflict of stellar ages and the age of the Universe.