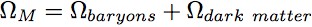
There are two possible futures for our Universe, continual expansion (open and flat), turn-around and collapse (closed). Note that flat is the specific case of expansion to zero velocity.

The key factor that determines which history is correct is the amount of mass/gravity for the Universe as a whole. If there is sufficient mass, then the expansion of the Universe will be slowed to the point of stopping, then retraction to collapse. If there is not a sufficient amount of mass, then the Universe will expand forever without stopping. The flat Universe is one where there is exactly the balance of mass to slow the expansion to zero, but not for collapse.

The parameter that is used to measure the mass of the Universe is the critical density, Ω. The cosmic density parameter, Ω, is usually expressed as the ratio of the mean density observed to that of the density in a flat Universe.

Given all the range of values for the mean density of the Universe, it is strangely close to the density of a flat Universe. And our theories of the early Universe (see inflation) strongly suggest the value of Ω should be exactly equal to one. If so our measurements of the density by galaxy counts or dynamics are grossly in error and remains one of the key problems for modern astrophysics.

Cosmological Models:

In modern cosmology, the different classes of Universes (open, flat or closed) are known as Friedmann universes and described by a simple equation:

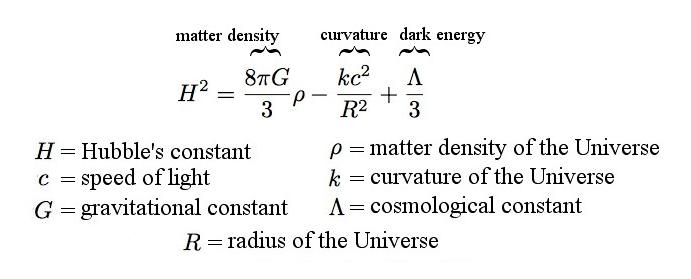
In this equation, `R' represents the scale factor of the Universe (think of it as the radius of the Universe in 4D spacetime), and H is Hubble's constant, how fast the Universe is expanding. Everything in this equation is a constant, i.e. to be determined from observations. These observables can be broken down into three parts gravity (matter density), curvature and pressure or negative energy given by the cosmological constant.

Historically, we assumed that gravity was the only important force in the Universe, and that the cosmological constant was zero. Thus, if we measure the density of matter, then we could extract the curvature of the Universe (and its future history) as a solution to the equation. New data has indicated that a negative pressure, or dark energy, does exist and we no longer assume that the cosmological constant is zero.

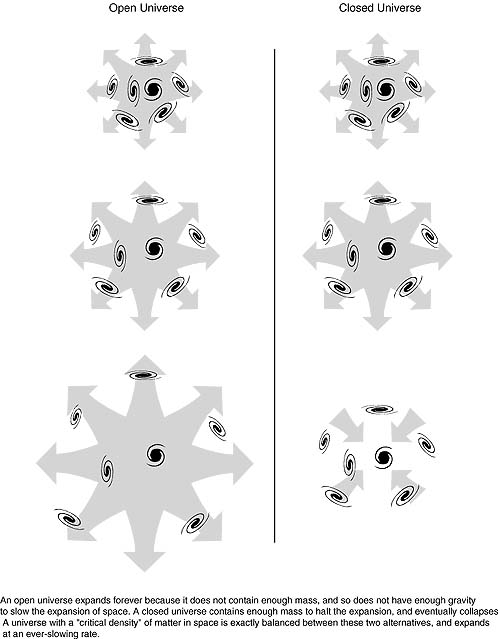
Each of these parameters can close the Universe in terms of turn-around and collapse. Instead of thinking about the various constants in real numbers, we perfer to consider the ratio of the parameter to the value that matches the critical value between open and closed Universes. For example, the density of matter exceeds the critical value, the Universe is closed. We refer to these ratios as Omega (subscript M for matter, Λ for the cosmological constant, k for curvature). For various reasons due to the physics of the Big Bang, the sum of the various Omega must equal one. And for reasons we will see in a later lecture, the curvature Omega is expected to be zero, allowing the rest to be shared between matter and the cosmological constant.

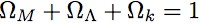
The search for the value of matter density is a much more difficult undertaking. The luminous mass of the Universe is tied up in stars. Stars are what we see when we look at a galaxy and it fairly easy to estimate the amount of mass tied up in stars, gas, planets and assorted rocks. This is contains an estimate of what is called the baryonic mass of the Universe, i.e. all the stuff made of baryons = protons and neutrons. When these numbers are caluclated it is found that Ω for baryons is only 0.02, a very open Universe. However, when we examine motion of objects in the Universe, we quickly realize that most of the mass of the Universe is not seen, i.e. dark matter, which makes this estimate of Ω to be much too low. So we must account for this dark matter in our estimate.