Infinity, in Western culture, means endless or immeasurable. Infinity commonly inspires feelings of awe, futility and fear. The symbol for infinity is called the lemniscate:

And is often seen in art and drawing, for example above the head of the Juggler in the Tarot set.

Greek word for infinite is apeiron = unbounded, for example to the Greeks the original chaos from which the Universe was formed was apeiron, or a crumpled handkerchief was apeiron. Thus, apeiron means not only infinitely large, but infinitely complex.

Plato rejected the concept of infinite following the tenets of Pythagoras that any given aspect of the Universe could be represented by a finite arrangement of natural numbers.

Aristotle recognized that there are many aspects of the Universe that point to the actuality of the apeiron. For example, it seems possible that time goes on forever, and the space is infinitely divisible (any line segment contains an infinite number of points). However, to protect his a priori finite world, Aristotle invented the notion of potentially infinite as opposed to actually infinite.

For example, Aristotle would state that the set of natural numbers is potentially infinite, since there is no largest natural number, but he would deny that the set is actually infinite, since it does not exist as one finished thing.

Infinity and God:

Plotinus was the 1st thinker after Plato to adopt that at least God (or the One) is infinite. He states that `the One has never known measure and stands outside of number, and so is under no limit either in regard to anything external or internal; for any such determination would bring something of the dual into it'. St. Augustine, who adapted Platonic philosophy to the Christian religion, believed not only that God was infinite, but also that God could think infinite thoughts. Later medieval thinkers did not go as far as Augustine and, although granting the unlimitedness of God, were unwilling to grant that any of God's creatures could be infinite.

St. Thomas Aquinas gives a sort of Aristotelian proof that ` although God's power is unlimited, he still cannot make an absolutely unlimited thing, no more than he can make an unmade thing (for this involves contradictories being true together)'. The argument is elegant, but suffers from the flaw of being circular: it is proved that the notion of an unlimited thing is contradictory by slipping in the premise that a `thing' is by its very nature limited.

Infinitesimals:

While the medieval proofs were most self-contradictory, they did discover one paradox involving infinity. Consider two circles, one twice the radius of the inner one (see below). The circumference of the outer circle is twice as big as the inner one, then the outer circle should include a larger infinity of points than the inner circle. But, by drawing a radii we can see that each point P and Q on the inner circle correspond to exactly one point Q' and P' on the outer circle. Thus, we seem to have two infinites that are simultaneously different and equal.

In the early 1600s Galileo offered a curious solution to this problem. Galileo proposed that the smaller circle could be turned into the larger circle by adding an infinite number of infinitely small gaps. This introduces the concept of indivisibles, with infinites transcends our finite understanding, the latter on account of their magnitudes, the former because of their smallness.

Galileo resolved some of his difficulties by asserting that problems arise only, `when we attempt, with our finite minds, to discuss the infinite, assigning to it those properties which we give to the finite and limited; but this I think is wrong, for we cannot speak of infinite quantities as being the one greater or less than or equal to another'. This assertion is supported by Galileo's paradox.

Galileo's Paradox:

Consider the sequence of numbers n (natural numbers) and n² (their perfect squares) shown below:

1  2  3  4  5  6  7  8 ...
1  4  9 16 25 36 49 64 ...

A paradoxical situation arises because, on the one hand, it seems evident that most natural numbers are not perfect squares, so that the set of perfect squares is smaller than the set of all natural numbers.

1  2  3  4  5  6  7  8  9 10 11 12 13 14 15 16 17 18 ...
1        4              9                   16       ...

For certainly there are many more natural numbers in the gaps between their matching squares, and these gaps grow larger with increasing numbers. But, on the other hand, since every natural number is the square root of exactly one perfect square, it would seem that there are just as many perfect squares as natural numbers. In other words, there is a one-to-one correspondence for every number in both sequences.

For Galileo, this meant that the totality of all numbers is infinite and that attributes such as `equal', `greater' or `less' are not applicable to infinite, but only to finite quantities. Candor will show, below, that the sequence of numbers n and n² are the same size, even though they have an infinite number of members, because you can make a one-to-one correspondence between each element in the sets. In fact, you can show that the natural numbers are also the same size as the set of all rational numbers.

Just because infinite sets do not behave like finite sets, this does not mean that infinity is an inconsistent notion. Rather, it means that infinite numbers obey a different set of arithmetic from finite numbers. This different kind of arithmetic takes its initial form as calculus in the late 17th century. In order to understand motion, the space position of an object is a function of continuously changing time. But the variable, time, grows continuously (e.g. from zero to ten seconds) but is apeiron in the sense that it takes on arbitrary values (1, 2.5, 6, etc) and also takes on infinitely many values in the interval.

Calculus solves this problem through the use of infinitesimals. To calculate the velocity of an object at moment t, one measures the speed over an infinitely small time interval dt. The quantity dt is an infinitesimal and obeys very strange rules. For example, if dt is added to a regular number, then it can be ignored and treated like zero. On the other hand, dt is different enough from zero that it can be used in the denominator of a fraction. So is dt zero or not? Adding many infinitesimals together just gives another infinitesimal. But adding infinitely many of them gives an ordinary number.

Cantor and Transfinites:

Georg Cantor, in the late 1800's, finally created a theory of the actual infinite which was consistent. His point of entry to the infinite was a mathematical problem to determine the uniqueness of the representation of a function as a trigometric series. To see one such construction, consider the Koch curve shown below.

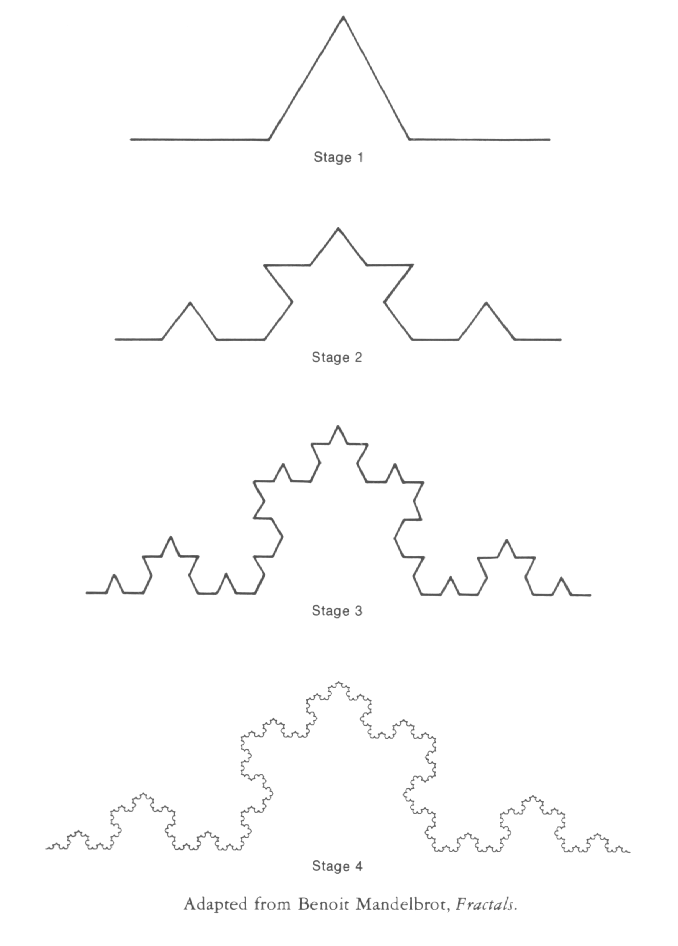
The Koch curve is found as the limit of an infinite sequence of approximations. The first approximation is a straight line segment (stage 0). The middle third of this segment is then replaced by two pieces, each as long as the middle third, which are joined like two sides of an equilateral triangle (stage 1). At each succeeding stage, each line segment has its middle third replaced by a spike resembling an equilateral triangle. We can regard the limit of this infinite process as being a curve that actual exists, if not in physical space, then at least as a mathematical object.

Cantor used construction like this to show that the set of points on a real line constitutes a higher infinity than the set of all natural numbers. One can see this intuitively when we consider the sequence of rational numbers between 0 and 1 (for example, 1/2, 1/3, 1/4, 1/101, 1/1,000,001). There are an infinite number of them, but always a gap between each value. For the real numbers on a line segment from 0 to 1 there is a continuum with no gaps, i.e. a larger infinity than rational numbers.

In other words, he showed that there were degrees of infinity. This fact runs counter to the naive concept of infinity: that there is only one infinity and this infinity is unattainable and not quite real. Cantor keeps this idea in this theory and calls it the Absolute Infinity, but he allows for many intermediate levels between the finite and the Absolute Infinity. We call these stages transfinite numbers, numbers that are infinite, but none the less conceivable.

There are three ways in which our physical world appears unbounded and, perhaps, infinite: temporal, spatial and endlessly subdivided (microscopic).

Time is often expressed in terms of human generations, although certainly time existed before humankind and will exist even if we become extinct. If we escape extinction, then we have to admit that the number of generations of man is infinite. Aristotle would argue that the number of generations is potentially infinite only in the sense of being inexhaustible. At any particular time there are only a finite number of generations. For an actual infinite, then all the future would have to exist as a stable, definite thing and all the future generations existing `at once'.

While this goes against common sense, it is in fact true. Einstein's theory of special relativity demonstrates that space and time are interlocked in something we call spacetime. It is spacetime that is fundamental and the passage of time is an illusion (although a fixed one). Past, present and future all exist together in spacetime.

Supertasks:

A supertask is a task that consists in infinitely many component steps, but which in some sense is completed in a finite amount of time. Supertasks were studied by the pre-Socratics and continue to be objects of interest to modern philosophers, logicians and physicists.

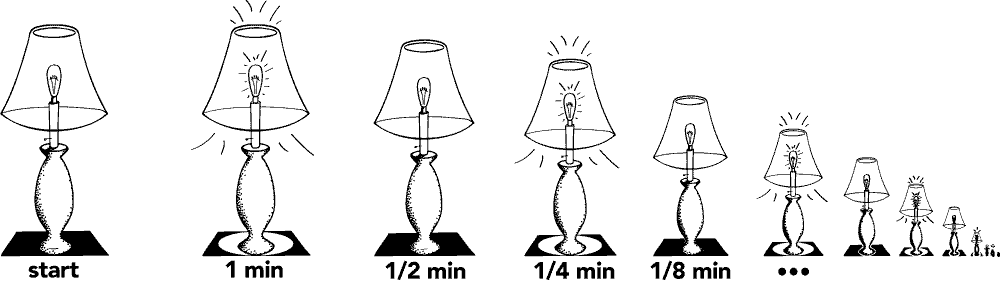
Supertasks are often described by sequences that do not converge. J. F. Thomson (1954) introduced one such example now known as Thomson's Lamp, which he thought illustrated a sense in which supertasks truly are paradoxical.

Suppose we switch off a lamp. After 1 minute we switch it on. After 1/2 a minute more we switch it off again, 1/4 on, 1/8 off, and so on. Summing each of these times gives rise to an infinite geometric series that converges to 2 minutes, after which time the entire supertask has been completed. But when 2 minutes is up, is the lamp on or off?

Supertasks in relativistic spacetime allows for infinite computation. For example, two spaceships take different paths to a star. One ship goes in a straight line (in spacetime) with minimal time. The other accelerates continuously such that their clock slows and slows. The accelerating spaceship is given a computational supertask, for example, they might check all possible counterexamples to Goldbachs conjecture (Goldbachs conjecture is the statement that every even integer n which is greater than 2 can be expressed as the sum of two primes. It is presently unknown whether the conjecture is true). If they find a counter example a signal is sent to the other spaceship. If no signal is detected, then in a finite time the first spaceship has the solution to an infinite task.

Of course, the construction of a hyperspace is a supertask, or the solution to the unexpected hanging.

Hyperspace:

Space is normally considered unbounded, meaning that if you travel outward with a spaceship you never encounter a barrier. However, things can be unbounded and finite.

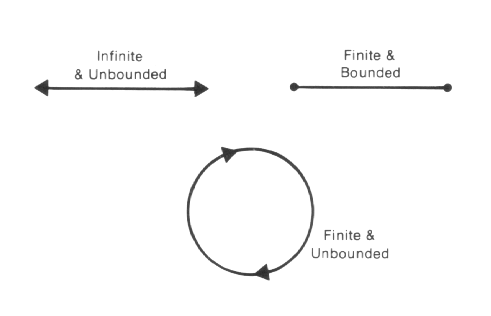
Here is an example of an infinite, yet bounded, space.

A circle is a one dimensional example. A 2D example is the surface of a sphere, such as the Earth's surface. You can travel forever on the Earth in what seems like a straight line, even though you only cover a finite distance. The reason the surface of a sphere is finite and unbounded is because it is bent in three dimensional space. In the same way, it is possible to imagine the three dimensional space of our Universe bent in four dimensional space, into what is called a hypersphere.

Hyperspherical surfaces have their origin even back to 12th century literature as a line of thought in Dante's Paradisio. Aristotle believed that the Universe was a series of nine spheres centered around the Earth. The last of these crystalline spheres was called the Primum Mobile and lay beyond the sphere upon which were fastened all of the other stars (other than the Sun which was attached to the fourth sphere). In the Paradisio, Dante is led out through space by Beatrice. He passes through each of the nine spheres of the world: Moon, Mercury, Venus, Sun, Mars, Jupiter, Saturn, Fixed Stars, Primum Mobile (note that on seven planets were known to the ancient astronomers, those visible to the naked eye). Beyond these nine spheres lie nine spheres of angels, corresponding to the nine spheres of the world. Beyond the nine spheres of angels lies a point called Empyrean, which is the adobe of God.

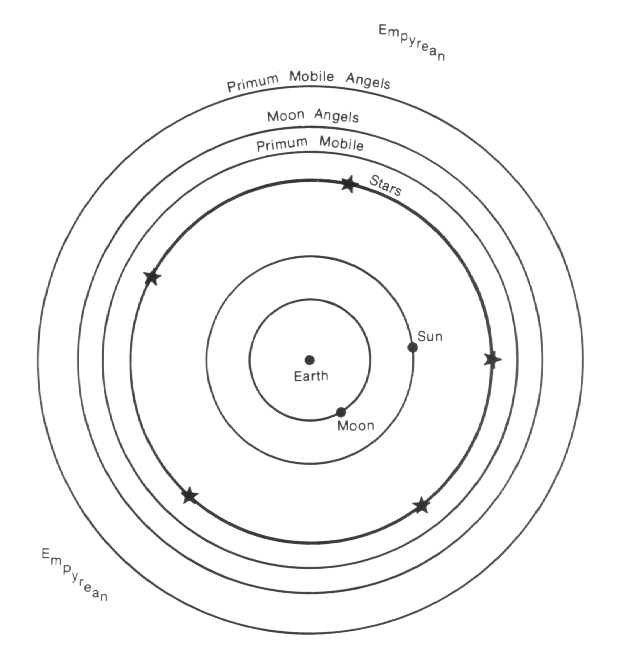
The puzzling thing about Dante's cosmology (see figure above) is that Empyrean appears not as a point, but rather as all space beyond the last sphere. This can be remedied if we take space to be hyperspherical. If we curve the 2D drawing into a 3D sphere, then the Primum Mobile becomes the equator and the spheres of angels decrease in circumference until the Empyrean point is reached.

Most examples of infinity refer to large things. But there also exist the infinities of the small or the problem of infinitely divisible. Its undeniable that a continuous region of mathematical space has an infinite number of mathematical points. But, is this also true of physical space, in other words, is matter infinitely divisible.

A commitment to avoiding the formless made it natural for the Greek atomists, such as Democritus, to adopt a theory of matter under which the seemingly irregular bodies of the world are, in fact, collections of indivisible, perfectly formed atoms.

Therefore, all the diverse substances of the Universe were regarded as being a mixture of the four elemental atoms: Earth, Air, Fire and Water. Gold, for example, was a very dense form of Water. Copper was gold with some Earth mixed in. Alchemists of the Middle Ages and early chemists adopted a similar system, only the four elemental atoms was expanded to include the whole periodic table. The fundamental unit became the molecule.

Still the search for the smallest unit of matter continues into this century with the discovery of elementary particles, electrons, proton, neutrons, that make up the atom. Then, further to the leptons and quarks that make up subatomic particles.

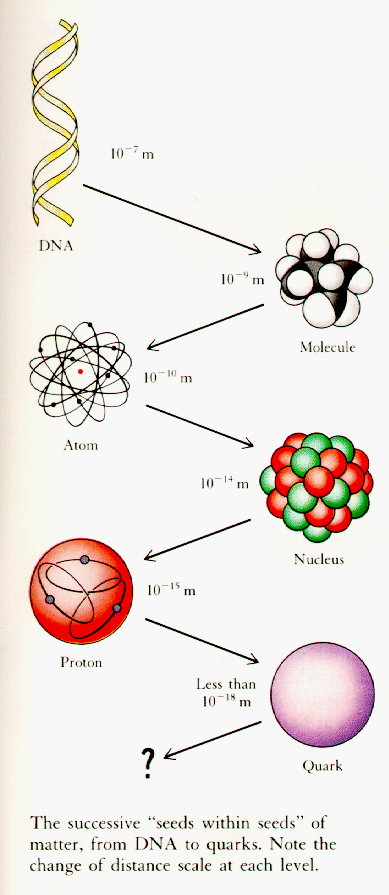
Thus, there appears to be no limit to the indivisibility of matter. However, there are objections to this sort of physical infinity. An Aristotelian argument is that quarks are only potentially (as opposed to actually) there. Since noone will be able to infinitely divide a material object, there are not really infinite number of particles in the object right now.

Another objection is that as we exam the subatomic world, the nature of matter changes. By splitting particles indefinitely we arrive at the conclusion that there is only form, and no content. The particle nature disappears and is replaced by a field-like nature. This becomes a dynamic description of the world. Imagine a the surface of a brook. There are circular ripples, eddies, whirlpools, bubbles and whatnot. The microscopic world view treats particles as objects such as those, which exist as features of the flow.

Horizons:

The particle horizon (also called the comoving horizon, or the cosmic light horizon) is the maximum distance from which particles could have traveled to the observer in the age of the universe. It represents the boundary between the observable and the unobservable regions of the universe, so its distance at the present epoch defines the size of the observable universe. Due to the expansion of the universe it is not simply the age of the universe times the speed of light, as in the Hubble horizon, but rather the speed of light multiplied by the conformal time. Note that the conformal time is not the age of the universe. Rather, the conformal time is the amount of time it would take a photon to travel from where we are located to the furthest observable distance provided the universe ceased expanding. The particle horizon recedes constantly as time passes and the conformal time grows. As such, the observed size of the universe always increases. The conformal time of the Universe is about 46.9 billion years.

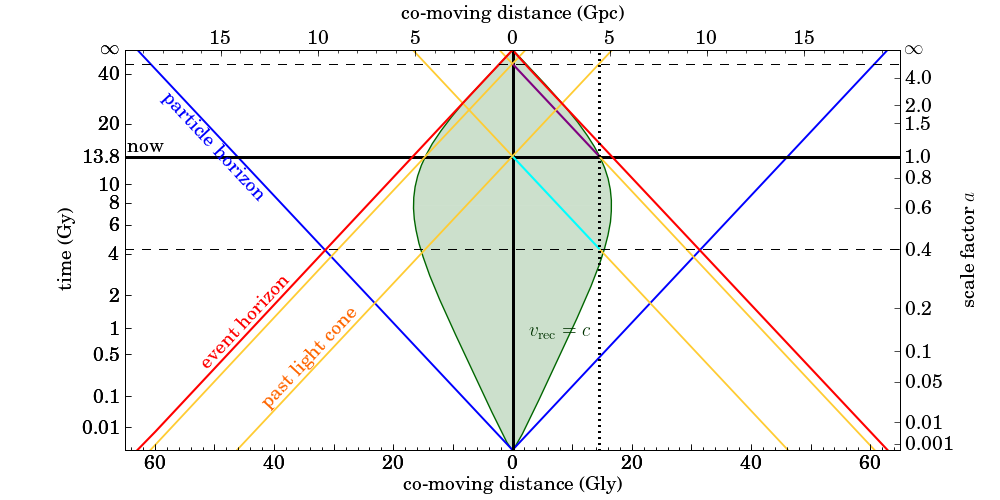
The future horizon (shown in green) for an accelerating Universe gets smaller in the future. There are events which will be unobservable as t goes to infinity as signals from future events become redshifted to arbitrarily long wavelengths in the exponentially expanding de Sitter space. This sets a limit on the farthest distance that we can possibly see as measured in units of proper distance today. Or, more precisely, there are events that are spatially separated for a certain frame of reference happening simultaneously with the event occurring right now for which no signal will ever reach us, even though we can observe events that occurred at the same location in space that happened in the distant past. While we will continue to receive signals from this location in space, even if we wait an infinite amount of time, a signal that left from that location today will never reach us. Additionally, the signals coming from that location will have less and less energy and be less and less frequent until the location, for all practical purposes, becomes unobservable. In a universe that is dominated by dark energy which is undergoing an exponential expansion of the scale factor, all objects that are gravitationally unbound with respect to the Milky Way will become unobservable, in a futuristic version of Kapteyn's universe.