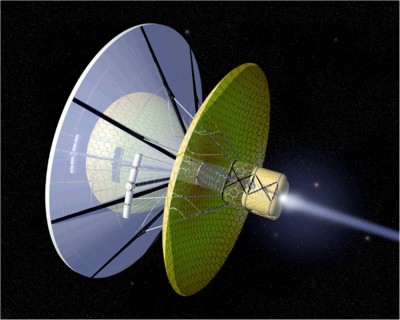

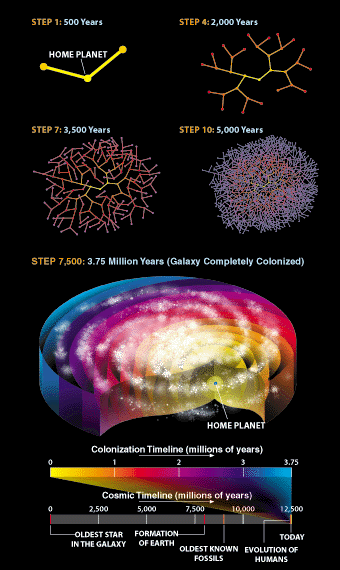
So what Fermi immediately realized was that the aliens have had more than enough time to pepper the Galaxy with their presence. But looking around, he didn't see any clear indication that they're out and about. This prompted Fermi to ask what was (to him) an obvious question: "where is everybody?"
Also, if one considers the amount of time the Galaxy has been around (over 10 billion years) and the speed of technological advancement in our own culture, then a more relevant point is where are all the super-advanced alien civilizations. Russian astrophysicist Nikolai Kardashev proposed a useful scheme to classify advanced civilizations, he argues that ET would posses one of three levels of technology. A Type I civilization is similar to our own, one that uses the energy resources of a planet. A Type II civilization would use the energy resources of a star, such as a Dyson sphere. A Type III civilization would employ the energy resources of an entire galaxy. A Type III civilization would be easy to detect, even at vast distances.
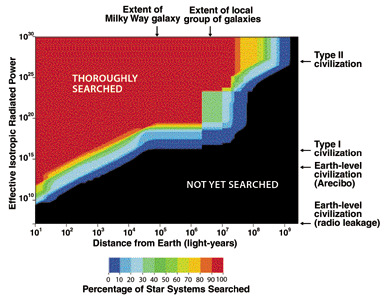
Thus, Fermi's paradox has two components; 1) why haven't the aliens visited us? and 2) why have we not detected their communications/evidence of their energy output? This sounds a bit silly at first. The fact that aliens don't seem to be walking our planet apparently implies that there are no extraterrestrials anywhere among the vast tracts of the Galaxy. Many researchers consider this to be a radical conclusion to draw from such a simple observation. Surely there is a straightforward explanation for what has become known as the Fermi's Paradox. There must be some way to account for our apparent loneliness in a galaxy that we assume is filled with other clever beings.

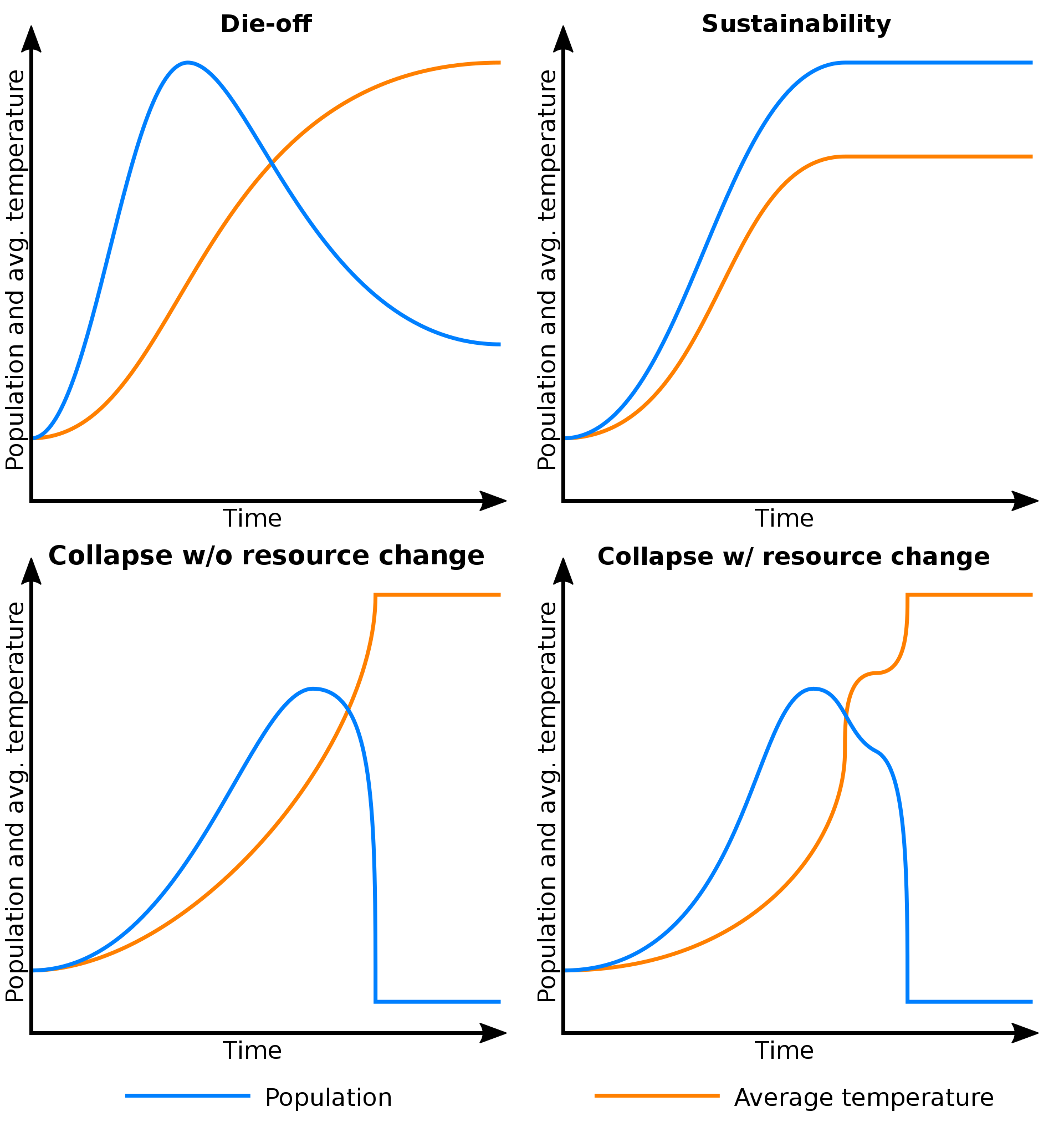
Scenarios in a mathematical model by en:Adam Frank et al., 2018, which describes climate change due to GHG emissions by an advanced energy-intensive civilization.[1] From left to right, top to bottom: Die-off: The world population reaches a peak and subsequently declines slowly until an equilibrium is attained. Population can decline by up to 90% after peaking; according to Frank, such a civilization "might well just descend into chaos." Sustainability: Both the world population and average surface temperature rise and then level off. This scenario shows that civilizations can be stable in the long term. Collapse without resource change: A civilization makes no attempt to switch to less GHG-intensive energy and collapses due to runaway climate change, possibly going extinct. Collapse with resource change: A civilization transitions to less GHG-neutral energy, but collapses anyway due to triggering a tipping point in the climate system.

In general, solutions to Fermi's paradox come down to either 1) life is difficult to start and evolve (either hard for the process or hard to find the right conditions) or 2) advanced civilizations destroy themselves on short timescales. In other words, this is an important problem to solve in the hope that it is 1 and not 2.

Very little experimental evidence for our current view of the structure of the Universe, so we depend on our sense of beauty. Where beauty here does not imply aesthetics, but rather conciseness, economy of concepts, brevity of mathematical expression, breadth of application.
Central to the beauty of our theories of how the Universe works is symmetry as expressed by Neother's theorem, a statement that for every continuous symmetry there exists a conservation law. Invariance of the laws of Nature to spatial translation, temporal translation and rotation means conservation of energy, mass and angular momentum. One symmetry that is *not* conserved is mirror symmetry. CP violation shows us that the Universe is chiral, a fancy word that means parity or handy-ness. So the Universe does distinguish between left and right handed interactions, Nature looks different in a mirror. Finding symmetries in a theory is important. By finding symmetries that a theory does not possess, a broken symmetry, is even more important.
When dealing with particles and their interactions, global symmetry makes no sense (why should the behavior of particles here on Earth have any effect on observations of particles on distant stars). Instead we restore symmetry through the use of a gauge field, a field that carries the information of symmetry around the Universe. For example, by demanding that electromagnetism obey local gauge symmetry we are forced to accept the existence of electromagnetic fields and the massless gauge boson is the photon. Similar requirements on all quantum fields produces quantum electrodynamics (QED). Objects in uniform motion or acceleration must also obey the laws of Nature, thus imposing local symmetry on motion forces a new field, the gravitational field described by general relativity, to appear.
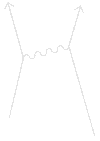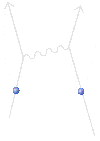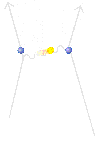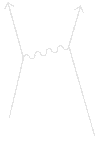
The Standard Model is incomplete as it does not specify the values of fundamental constants nor does it combine with gravity. Two possible avenues for extensions of the Standard Model are grand unified theories (GUTs) and supersymmetry (SUSY). To unify weak and electromagnetism we simply write down a theory with enough gauge symmetry to accommodate the four mediators bosons (photon, W+, W- and Zo). Through the use of the Higgs mechanism (where a general field fills the Universe in which particles can interact to acquire mass), we break the symmetry to get three massive bosons (W+, W-, Zo) and one massless boson (the photon). To unify gluons with the other sub-atomic force carriers we need a new, larger gauge symmetry to bring everyone together. A new symmetry would make the distinction between quarks and leptons go away (at least until the symmetry break, until then we have GUT matter).
One consequence of quark/lepton symmetry is that protons, once thought to be stable, must decay under GUT. However, this is a problem for GUT as current experiments have not been able to detect proton decay and its half-life must be greater than 1032 years. We are also unable to experiment at the GUT level as we would need to force quarks within a radius of 10-31 meters in order to exchange a GUT boson. This would require energies on the order of 1015 GeV which is 1013 times greater than our current technology. But, ultimately, GUT fails due to the gauge hierarchy problem, the fact that the difference between the electroweak and GUT symmetry breaking points implies two difference masses for the Higgs boson (102 GeV vs. 1015 GeV) means the GUT is insufficient.
To have a complete set of all possible spacetime symmetries, one symmetry is missing in the Standard Model. This would be the ability to transform particles into different particles, ones with different spin. This symmetry, called supersymmetry, transforms fermions into bosons and vice versa. Thus, the distinction between particles of matter (fermions) and particles of force (bosons) would blur. Evidence of supersymmetry would show us that Nature has utilized all mathematically consistent spacetime symmetries.
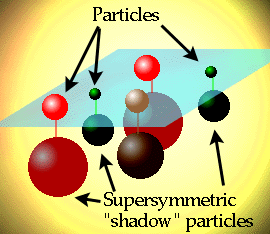
Supersymmetry (SUSY) has the initial advantage of solving the gauge hierarchy problem under SUSY GUT through the introduction of a whole set of supersymmetric partners to each known particle. Requiring a local symmetry for supersymmetry forces two gauge fields, one that communicates information about transformations and a second that communicates information about translation in spacetime using massless spin-2 particles. A massless spin-2 particle is one that carries a long-range force that is only attractive, i.e. gravity and the particle is the graviton. So supersymmetry automatically contains a gravitational field theory. Thus, local supersymmetry is called supergravity since general relativity appears naturally when SUSY is made local. When supergravity breaks, what is left is a low energy supersymmetric grand unified theory (GUT).
The first attempt to explore other dimensions beyond the four of spacetime was Kaluza-Klein theory, a description of spacetime using five dimensions. From a 5D theory, 4D forms of both electromagnetism and general relativity appear naturally. The extra dimension is associated with the sub-atomic world in order to explain `where' this dimension is. The original formulation of the Kaluza-Klein theory had the 5th dimension as circular, so that we need four coordinates to describe a normal event (three spatial and one of time) plus an additional coordinate to specify a position on the circle. So imagine a garden hose, from a distance it looks like a line, but close up we could see an ant moving backwards and forward on the hose plus around the hose. If this additional dimension is smaller than the radius of an atom, then we would not detect or measure its presence. The small size on this extra dimension is explained by inflation, the 4D universe inflated leaving behind the 5th dimension to compact into very small scale size.
Kaluza-Klein theory fails to resolve several problems, especially in the quantum realm, but the idea of using extra dimensions is revisited in the early 1980's. The new twist is instead of considering a single extra dimension, modern theory supposes that at each point in spacetime there is a compact space with many dimensions, a manifold at each point. The minimum number of dimensions that at compact manifold could possess, and still maintain symmetry, is seven, making the minimum of dimensions for spacetime to be 11. Interestingly enough, the maximum number of dimensions one can write down for a consistent supergravity theory is also 11. Unfortunately, 11D supergravity does not quite work. While it may be an effective theory that works at low energies, it cannot be the final theory of everything, mostly because of a problem with infinities. Quantum field theory treats objects like quarks and leptons as point-like. But calculations dealing with gravitons eventually encounter infinities, which is a sign that the theory is flawed. One approach to resolve this dilemma is to abandon point-like particles and consider 1D strings.
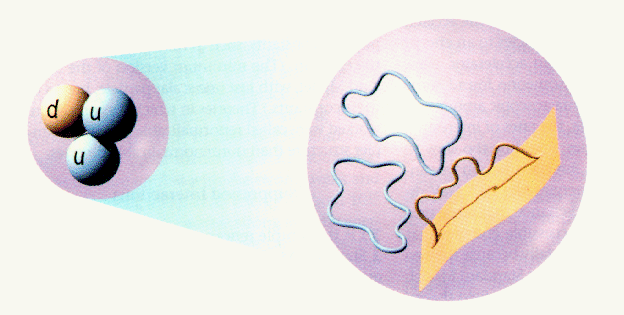
Strings come in two types, open (like a line segment) and closed (like a loop). An open string moving through spacetime traces out a sheet. A closed string will trace out a tube. Combining string theory with supersymmetry produced superstring theory which was a 10D spacetime (one short of supergravity). Superstring must be embedded inside a larger theory, called M-theory. M-theory contains objects called branes which do not correspond to oscillation states of strings. Branes can come in any number of dimensions, a 2D brane would be a sheet or membrane. The energy of a brane is concentrated on its surface, its energy density is its tension. Under this view, branes form the structure of the Universe and all the Standard Model fields are restrict to the brane, but closed strings (like gravity) are free to travel between branes. The collection of branes would form a bulk of many brane walls.
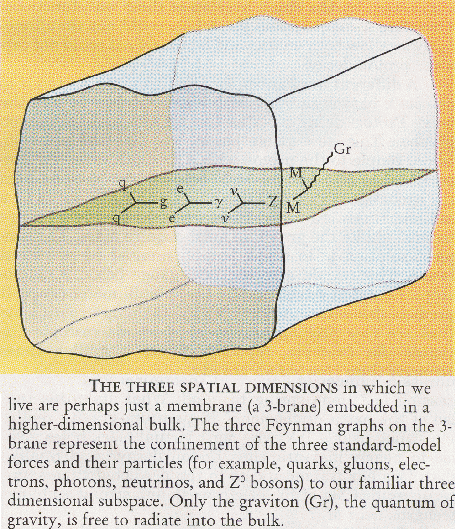
So the final version of the structure of the Universe is a series of branes, each containing the 4D macroscopic world and a compactified 6D manifold that the Standard Model operates in the microscopic world. The collection of branes forms a bulk in 11D spacetime, only gravitons can travel between the branes (explaining why it is so weak as a force as it is `spread' over the bulk). Thus, in a real sense, there are many parallel Universes nearby in the bulk on other branes. When branes collides you can have a realize of energy (i.e. the Big Bang) and inflation to form many multiverses in a brane.

