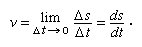
Calculus is the branch of mathematical analysis concerned with the rates of change of continuous functions as their arguments change. Two men are now credited with discovering calculus, Sir Isaac Newton of England and Gottfried Wilhelm Leibniz of Germany. For almost a century, development of the subject was inhibited by a bitter controversy over priority between supporters of Newton and those of Leibniz.

A basic concept of calculus is "limit," an idea applied by the early Greeks in geometry. Archimedes inscribed equilateral polygons in a circle. Upon increasing the number of sides, the areas of the polygons (which he could calculate) approach the area of the circle as a limit. Using this result together with a similar idea involving circumscribed polygons, he was able to find the area of the circle as r², in which r is the radius of the circle and (pi) is a constant that has a value between 3 1/7 and 3 10/71.

The area of an irregularly shaped plate also can be found by subdividing it into rectangles of equal width. If the number of rectangles is made larger and larger, the sum of their areas (found by multiplying base by height) approaches the required area as a limit. The same procedure can be used to find volumes of spheres, cones, and other solid objects. The beauty and importance of calculus is that it provides a systematic way for the exact calculation of many areas, volumes, and other quantities that were beyond the methods of the early Greeks.

Newton's discovery of calculus, legend says, may very well have been inspired by an apple falling from a tree. As an apple falls, it moves faster and faster; that is, it has not only a velocity but an acceleration. Newton expressed this mathematically by supposing that at any stage of its motion the apple drops a small additional distance s (delta s) during a brief additional time interval t (delta t). Then the velocity is very nearly equal to the distance s divided by the time t--i.e., s/t. The exact velocity v would be the limit of s/t as t gets closer and closer to zero or, as we say, approaches zero. That is,

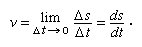
The quantity ds/dt is called the derivative of s with respect to t, or the rate of change of s with respect to t. It is possible to think of ds and dt as numbers whose ratio ds/dt is equal to v; ds is called the differential of s, and dt the differential of t.

Just as velocity is the rate of change, or derivative, of the distance with respect to time, so the acceleration is the rate of change, or derivative, of the velocity with respect to time. Therefore a, the acceleration, would be

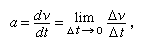
where v is the increase in velocity that occurs during the interval t. Since a is the derivative of v and v is the derivative of s, a is called the second derivative of s:

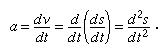
To find derivatives of s with respect to t, the dependence of s on t must be known; in other words, s must be expressed as a function of t. Usually this functional dependence is stated as a formula relating s and t. That part of calculus dealing with derivatives is called differential calculus.

Given s as a function of t, the derivative (that is, v) of s can be found. Conversely, if v is known it is possible to work backward to get s. This process of finding what is called the anti-derivative of v is begun by rewriting the equation v = ds/dt as ds = vdt. The quantity s is here regarded as the anti-differential of ds, denoted by a special symbol called an integral sign:

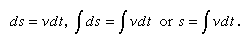
The last equation specifies s the integral of v with respect to t. That part of calculus dealing with integrals is called integral calculus. Applications of integral calculus involve finding the limit of a sum of many small quantities, such as the rectangular slices of an irregular plane figure.

Excerpt from the Encyclopedia Britannica without permission.