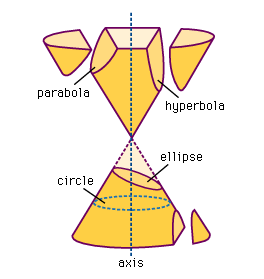
Any curve produced by the intersection of a plane and a right circular cone. Depending on the angle of the plane relative to the cone, the intersection is a circle, an ellipse, a hyperbola, or a parabola.

Conics may be described as plane curves that are the paths (loci) of a point moving so that the ratio of its distance from a fixed point (the focus) to the distance from a fixed line (the directrix) is a constant, called the eccentricity of the curve. If the eccentricity is zero, the curve is a circle; if equal to one, a parabola; if less than one, an ellipse; and if greater than one, a hyperbola.

Excerpt from the Encyclopedia Britannica without permission.