Diffraction in Hexagonal Microcrystals
Dye-doped zeolite microcrystals*
A new class of materials for optical
applications are the
guest-host
systems, in which a species of (sufficiently small) molecules
is encapsulated into the "hollow spaces" of a medium such as
porous silica or a
zeolite, which is therefore called
the "host" matrix. Such hybrid materials exhibit new properties
that neither the host nor the guest molecules can produce
alone. This is because the pores in which the guest molecules
reside serve to impose order and stability that limits their
motion and can even force the incorporated molecules to assume
a particular orientation.
This happens for example in zeolite microcrystals that are
doped with an organic dye as "guest" molecules. The dye is able
to emit light by fluorescence, and because of the imposed
orientation of the organic molecules in their host matrix, the
light is polarized.

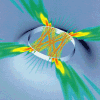
The nanopores of the zeolite form a "molecular sieve" with a
hexagonal arrangement. If one grows crystallites out of this
material, this translates into a hexagonal facet
structure. The light generated by the fluorescent dye molecules
now gets trapped inside this self-assembled hexagonal
microresonator by reflecting off the facets. The polarization
properties of the medium favor propagation in a cross-sectional
plane of the crystal, whose size is below 10 micron
diameter.
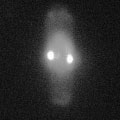
These tiny grains of hybrid material can in
fact be made into extremely small lasers.
Apparently, the light is trapped sufficiently long to provide
the necessary feedback for light amplification. But pores in a
material usually reduce the overall refractive index n, and in
fact the zeolite-dye system has n=1.45 - this makes it easy for
rays in such a resonator to escape to the surrounding air by
the law of refraction.
This raises the question: what do the
modes look like ?
What kind of long-lived ray paths
can one imagine in this cavity ? There can only be lasing from
modes corresponding to rays with angles of incidence in the
"whispering-gallery" region, i.e. travelling close to the
surface. But are there such WG modes ? Look at these periodic
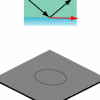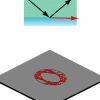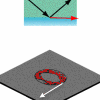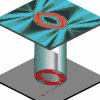
ray paths:
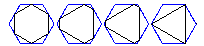
All orbits here have the same angle of incidence, and the
same length. They constitute a family of rays, all of which
can be expected to make a contribution to the resonator modes.
There is nothing a priori that singles out the symmetric
inscribed hexagon (left), and in fact admixtures from all of
these orbits can contribute to any single mode.
What about their escape behavior? Total internal reflection
confines these modes, but the classical Fresnel formulas
break down at the corners. The degenerate periodic orbit
hitting the corners (right) is therefore special because simple
total internal reflection doesn't work there. So the
corners are where the escape should happen in a
preferred way.
That is in fact what is seen in experiment and also what I
find in my wave simulations. Here is an example:
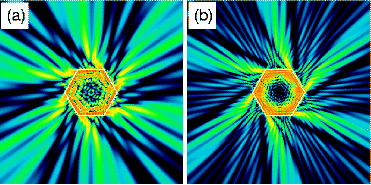
This is for microcryastals with n=1.45 at (a) 4 and (b) 8
micron diameter, wavelength 600…700 nm. To read more
about the "pinwheel-like" asymmetry in the
emission, follow this link.
The experiments were carried out by Uwe Vietze and Franco
Laeri. This group in Darmstadt, in collaboration with Bremen
University and the MPI für Kohleforschung did the
pioneering development leading to zeolite nanocomposites with
lasing action. For the small cavities studied here, the ray
picture doesn't give a correct description of the actual
emission pattern due to strong corner diffraction.
Together, we conducted a comprehensive investigation of this
micro-optical system, published in Applied Physics B, see also my publication
list.
The hexagonal resonator is interesting from a theoretical
point of view because its internal dynamics is
pseudointegrable, a pathological condition. It causes
the rays patterns to look rather neat compared with a chaotic
cavity - but don't let this fool you. Even if we neglect the
copmplication of leakage from the resonator, the wave fields
for this system cannot be written down in any simple way,
except for a subset of solutions whose intensity vanishes on
all the diagonals (and hence in particular on the corners). But
the interesting solutions are precisely those with nonzero
intensity on the diagonals, because the ray picture tells us
that those types of fields favor escape at the corners.
The pathological properties of pseudointegrable systems can
be studied especially well in
polygonal billiards , and in this way the importance of
diffraction
as a correction to geometric optics is revealed.
The theoretical interest in the hexagonal resonator combines
in an ideal way with the great significance of this geometry in
naturally occuring and artificially engineered micro-optical
systems. Perhaps the first hexagonal microstructure that comes
to mind is in fact the snow crystal
(that's what snowflakes are made of) . In tropical cirrus
clouds, such crystals are less than 50 micron small, i.e. they
are micro-optical systems. Their scattering properties are of
great importance in meteorology because of their potential
climatic effects. Of course, people have not been that
interested in the possibility of long-lived resonances in
snowflakes... certainly my kids are more interested in the
lifetime of the snowflake itself.
Photonic states in a hexagon are, however, very important when
it comes to microlasers made of Gallium Nitride - one of the
most promising candidates for the elusive goal of producing
blue light with a semiconductor laser diode. This compound
grows with a hexagonal facet structure as well !
* Remark:
This work was selected for the "Optics in
2000" section of Optics and Photonics News, December
2000.
This page © Copyright Jens Uwe Nöckel,
06/2003
Last modified: Mon Mar 14 20:05:51 PDT 2011