Thinking about the Cosmic Background Radiation
It is not so easy to think about the expanding universe and the cosmic microwave background.
It's a lot easier if you have a deep understanding of
- the Special Theory of Relativity and how time and space are interrelated;
- the General Theory of Relativity and curved space time.
That, however, requires lots of mathematics.
Nevertheless, simple ideas can take us a long way.
In particular, ``local'' thinking is easy. It is the global picture that
is hard.
Here are some local ideas.
- Let the people (if any) on each galaxy measure time t as the time since
the big bang -- according to a ``clock'' on their galaxy.
- Here and now, t is approximately 10 billion years.
- The nearby matter is (on average) moving away from us according to the Hubble law.
- For a person on another galaxy at the same time t, the situation looks the same.
- Nearby matter is (on average) moving away from the other galaxy according to the Hubble law.
- The Hubble constant is the same.
- The person does not see an edge of the universe.
- At earlier times t the expansion should have been somewhat faster, because gravity would have slowed it down.
- That is, the Hubble constant would have been bigger.
- How much bigger depends on how much mass there is.
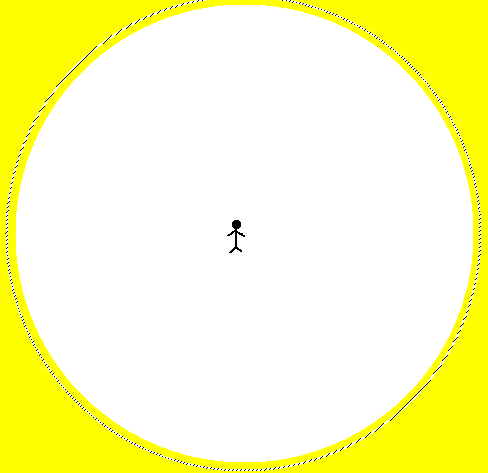
Now think about what we would see a mere 0.5 million years after the big bang.
We had a gas of protons, electrons, and lots of photons.

Here is what you would see.

You can see just a little way because the ionized gas is opaque.
Now think about what we would see a mere 5 million years after the big bang.
We had a gas of atoms and lots of photons.
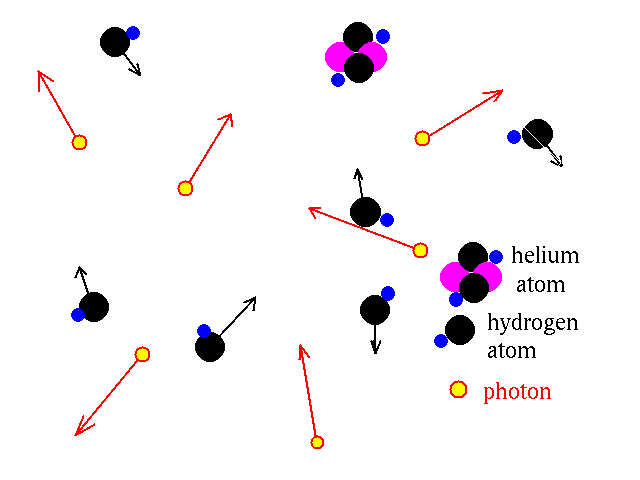
Here is what you would see.
You can see a rather long way.
- But light has a finite speed.
- So the light you see from further away came from events further
in the past.
- Looking 4 light years back, the light came from the hot, opaque gas.
If we look now, we can see back in time many billions of years. That's
not very local.
- We can describe distant objects by their redshift, usually
denoted by z.
1 + z = (wavelength observed)/(original wavelength)
- Then z serves as a kind of cosmic distance measure.
- Bigger z corresponds to earlier times.
- With a little relativity, one finds that z --> infinity
corresponds to t --> 0.
- Looking to z ~ 1000 corresponds to t ~ 1 million years.
- That is the ``decoupling'' time when atoms formed.
- The temperature at that time was about 3000 K.
- The photon spectrum at that time was a blackbody spectrum corresponding
to 3000 K.
- But the light is redshifted by a factor of 1000.
- So now we see blackbody radiation corresponding to a temperature of
about 3 K.
Davison E. Soper, Institute of Theoretical Science,
University of Oregon, Eugene OR 97403 USA
soper@bovine.uoregon.edu




