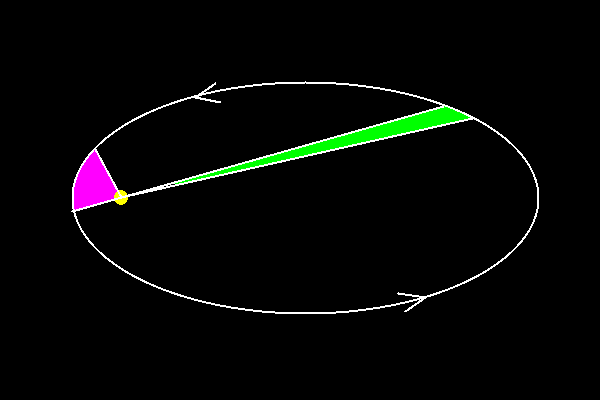
This means that the planet moves faster when it is near the sun, slower when it is far away.
As the planet moves in its orbit, a line from the sun to the planet sweeps out equal areas in equal times.

This means that the planet moves faster when it is near the sun, slower
when it is far away.
The eccentricity of the earth's orbit is 0.017. This means that the distance from the earth to the sun when we are nearest to the sun is ( 1.000 - 0.017) AU = 0.983 AU. Similarly, the distance from the earth to the sun when we are farthest from the sun is ( 1.000 + 0.017) AU = 1.017 AU.
This is not much of a difference, but using Kepler's second law, we can deduce something. Call the time of day when the sun crosses the great circle in the sky that contains the north celestial pole and the zenith ``local noon.'' When the earth is nearest the sun, is the time from one ``local noon'' and the next ``local noon'' bigger or less than 24 hours?