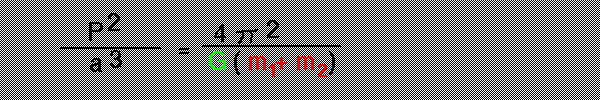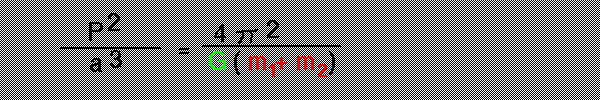Newton's Results for the orbits of the planets
Using his three laws and the law of gravity Newton predicted ...
(well, first he had to invent calculus)
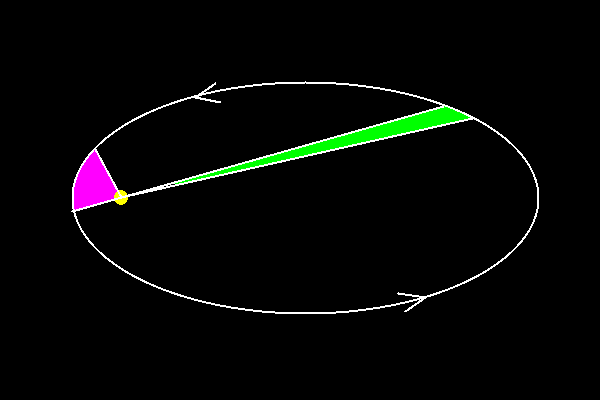
- The planets move in elliptical orbits
with sun at one focus.

- The speed at which a planet moves varies along the orgit so that
a line from the sun to the planet sweeps out equal areas in equal times.
- The periods P of the planet's orbits are related to
the semimajor axes a of their orbits by
In fact, Newton found the constant:
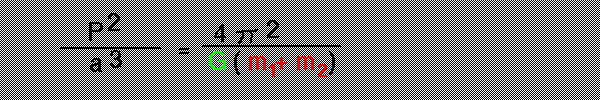
This relation allows one to measure the masses of the Earth,
the Sun, other planets, stars ...
What Newton derived was Kepler's three laws. The laws were already known, of course, but it wasn't known where they came from. Newton showed that the same laws of mechanics that govern the motion of objects on earth also govern
the motion of the planets.
We can use this relation to find the mass of a planet if it has a moon
(or a space probe) orbiting it. If we know P and a we
can use

To get the sum of the masses of the planet ( m1) and the
mass of the moon (m2). Typically, the mass of the moon
is negligably small compared to the mass of the moon. Then we have
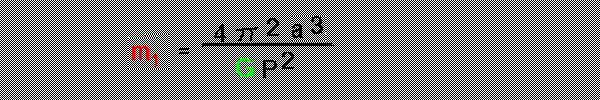
Study hint: These last three formulas are a little complicated. For
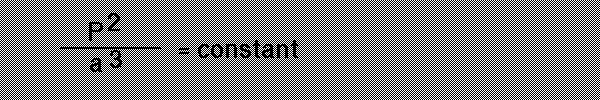
our purposes, it is enough to remember Kepler's Third Law, that
P2 = constant * a3 for all of the planets
in the solar system or (with a different constant) for all of the moons
orbiting Jupiter. Then we need to understand that from the motions
of Jupiter's moons we can find the mass of Jupiter, but I do not
recommend memorizing these three formulas.
ASTR 121 Home
Davison E. Soper, Institute of Theoretical Science,
University of Oregon, Eugene OR 97403 USA
soper@bovine.uoregon.edu