Possibilities shaped by constraints of arithmetic

Gaussian Fireworks

Ellen Eischen

 

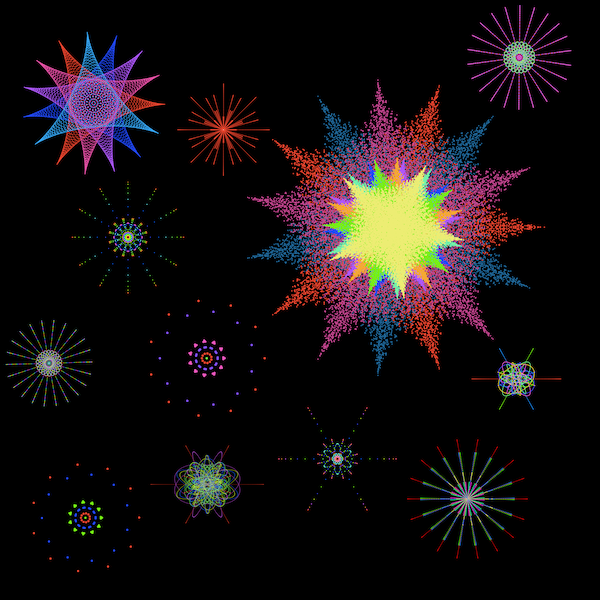
Gaussian Fireworks

When is the last time you were surrounded by fireworks and didn’t notice? Assuming someone ignited them, that probably never happened. The numbers plotted in Gaussian Fireworks were well-known to mathematicians for over two centuries, though, before anyone noticed their firework-like formations.

Advances in computing recently enabled us to plot millions of these numbers, Gaussian periods, and see the variety and beauty in their patterns. This opportunity was not available to Carl Friedrich Gauss when he introduced them at the turn of the nineteenth century. Like a few specks of light provide no clue to the general shape of an entire firework, a plot of just a few Gaussian periods gives no hint of the beauty lurking at a much larger scale. A few years ago, an undergraduate at Pomona College, Bob Lutz, stumbled upon their intricate patterns as he experimented with plotting families of hundreds of thousands of Gaussian periods.

The origins of Gaussian periods lie in the answer to a simple question, which stumped the ancient Greeks and others for 2000 years:

Which shapes can you draw with an unmarked straightedge and compass, if you require all the edges to be the same length and the angles all to be equal to each other?

Using Gaussian periods (which mathematically inclined viewers might be impressed to learn are complex numbers), Gauss and Pierre Wantzel determined when such shapes could be constructed. Gaussian periods have also continued to play important roles in mathematics, especially in number theory. Because the possibility of seeing their large-scale plots is so new, though, many mathematicians continue to be unaware of their striking visual aspects.

Now, you can explore further and create your own plots at:

http://www.elleneischen.com/gaussianperiods.html

Just as chemical properties determine the colors in a firework, mathematical properties of these numbers determine their colors in these pictures. Unlike with real fireworks, you can safely experiment with their infinitely many variations at home.

Related Courses at the University of Oregon

Courses include Galois theory and number theory, such as the University of Oregon’s Math 446, Math 347, and Math 348.

Learn More about this Area…

Books for the public include Richeson’s Tales of Impossibility: The 2000-Year Quest to Solve the Mathematical Problems of Antiquity, Stewart’s Professor Stewart’s Incredible Numbers (the chapter on complex numbers), and Parker’s Things to Make and Do in the Fourth Dimension (Chapter 2).

Research papers include:

  • E. Eischen and S. Garcia, “A gallery of gaussian periods.” Proceedings of Bridges 2020: Mathematics, Art, Music, Architecture, Education, Culture (Phoenix, Arizona) (Carolyn Yackel, Robert Bosch, Eve Torrence, and Kristof Fenyvesi, eds.), Tessellations Publishing, 2020, pp. 243–248.
  • S.R. Garcia, T. Hyde, and B. Lutz. “Gauss’s hidden menagerie: from cyclotomy to supercharacters.” Notices of the American Mathematical Society, vol. 62, no. 8, 2015, pp. 878–888.
  • W. Duke, S.R. Garcia, and B. Lutz. “The graphic nature of Gaussian periods.” Proceedings of the American Mathematical Society, vol. 143, no. 5, 2015, pp. 1849–1863.