



Russell J. Donnelly
541-346-4226 (Tel)
541-346-5861 (Fax)

![]() Progress
in Cryogenic Fluid Mechanics
Progress
in Cryogenic Fluid Mechanics
This
informal report is an attempt to give an overall picture of research in
fluid mechanics using low temperature techniques at the University of
Oregon and Yale University over the past several years. This effort has
been a prime example of the felicitous application of low temperature
techniques to other fields. We first became interested in these possibilities
in the late 1980’s when the (old) idea of a superfluid wind tunnel
was seriously reexamined.
•
Thermal convection
Those devoted to the study of quantum fluids are aware of the unprecedented sorts of fluid dynamics that occur when superfluids are present. What has been slow for many of us to grasp are the other extraordinary fluid mechanical tools low temperature physics provides. A prime example is in the field of thermal convection. A layer of fluid of height L heated from below and cooled from above produces a temperature difference DT and an associated gradient in the density r. For typical fluids with a positive thermal expansion coefficient, the denser fluid lies above the less dense fluid, creating a gravitationally unstable configuration. For small DT, the layer remains at rest. But if DT is large enough, convection will occur. Just above the onset of convection, the flow takes the form of closed circular stream lines. If the flow is visualized from above, the flow pattern consists of bands of up-and-down flows called convection rolls. With further increases in DT, the rolls become unstable. This typically results in unsteady or time dependent flow. With yet further increase in DT, the time-dependence passes through a chaotic regime where the rolls are still identifiable, but where the motion is difficult to predict. For even larger DT, the roll structure is lost, and turbulent flow sets in.
There are several parameters which determine the character of the flow. The key control parameter is the Rayleigh number, Ra, which is a dimensionless measure of DT.
![]()
with
a Isobaric thermal expansion coefficient
k Thermal diffusivity
L Height of the convecting layer
![]() Adverse temperature drop across
the layer
Adverse temperature drop across
the layer
g Acceleration of gravity
The
dynamical equations for Rayleigh-Bénard convection contain only Ra and the Prandtl number
![]() . In addition, the flow depends on the geometry of the container, as expressed
by the aspect ratio G = D/L, where D is the diameter.
Thus, the scaled flow properties should be the same for two systems
having the same set of parameters Ra,
Pr and G
(assuming that the geometry remains unaltered).
The structure of flows as a function of Ra is important for understanding the reasons for a large scale convective
turbulence experiment. The larger
the Ra, the larger is the range
of scales excited in the turbulent flow.
Ra can be made large
by choosing the fluid such that the combination a/kn is
large, by making DT large, or by making L
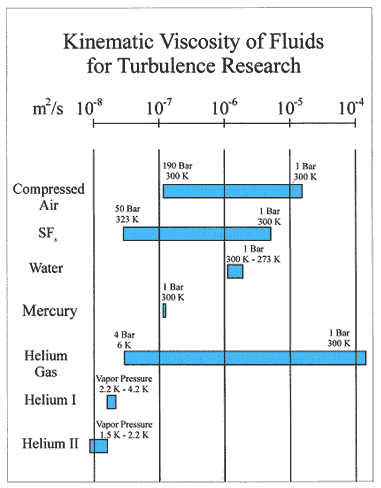
large. To our knowledge, low temperature
helium is the optimum fluid by a substantial margin for making a/kn large. The quantity
a/kn can be a million times
larger for helium gas near the critical point than for water. Various choices for fluids of different kinematic
viscosity are shown in the plot below.
Although, in principle, DT could be made large, this is undesirable because the fluid will
then no longer obey the Boussinesq approximation, rendering the theory
considerably more difficult. For
instance, for large DT, the density could no longer be assumed
to depend linearly on temperature. Because
Ra depends on the third power
of L, extremely large Rayleigh
numbers can be attained by making L
large.
. In addition, the flow depends on the geometry of the container, as expressed
by the aspect ratio G = D/L, where D is the diameter.
Thus, the scaled flow properties should be the same for two systems
having the same set of parameters Ra,
Pr and G
(assuming that the geometry remains unaltered).
The structure of flows as a function of Ra is important for understanding the reasons for a large scale convective
turbulence experiment. The larger
the Ra, the larger is the range
of scales excited in the turbulent flow.
Ra can be made large
by choosing the fluid such that the combination a/kn is
large, by making DT large, or by making L
large. To our knowledge, low temperature
helium is the optimum fluid by a substantial margin for making a/kn large. The quantity
a/kn can be a million times
larger for helium gas near the critical point than for water. Various choices for fluids of different kinematic
viscosity are shown in the plot below.
Although, in principle, DT could be made large, this is undesirable because the fluid will
then no longer obey the Boussinesq approximation, rendering the theory
considerably more difficult. For
instance, for large DT, the density could no longer be assumed
to depend linearly on temperature. Because
Ra depends on the third power
of L, extremely large Rayleigh
numbers can be attained by making L
large.
The heat flux, q, plays an obviously important role in Rayleigh-Bénard convection, since it generates the temperature difference, DT. Note that q = Q/A where A is the cross-sectional area of the apparatus, and Q is the heat current. It is convenient to define a dimensionless measure of the heat transport, the Nusselt number, Nu: Nu = Q/Qc where Qc is the portion of the heat current carried by conduction alone. Note that Nu depends only on the dimensionless parameters of the problem, i.e. Ra, Pr and G.
Brian
Pippard realized before anyone else the utility of critical helium gas, and his
graduate student Threlfall was the first to show the great range of Rayleigh
numbers possible in one apparatus, achieving Rayleigh numbers from 60
to 2x109. Threlfall’s work stimulated a great deal of
theoretical and experimental work at ![]() . After much turmoil, the theorists
came forth with Nu~Ra 2/7 which was somewhat near Wu’s results.
. After much turmoil, the theorists
came forth with Nu~Ra 2/7 which was somewhat near Wu’s results.
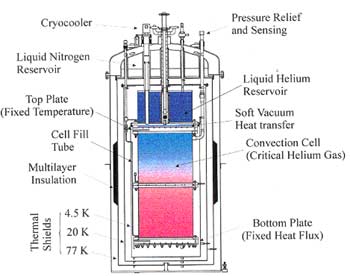
When the SSC was terminated, DOE ran a competition to find uses for resources like liquid helium plants. We competed successfully and were able to draw up conceptual designs for a 10 m high convection tank that would, in principle, achieve Rayleigh numbers of 1022, ie. of interest in astrophysical fluid dynamics. This is still in the future. However with the help of NSF, we proceeded to plan and construct a 1m high Bénard cell , and were able to reach Ra~10^17. The apparatus and results are shown below.
![]()
![]()

![]()
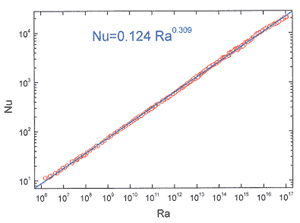
To the best of our knowledge this figure represents the largest
dynamical range observed (11 decades in Rayleigh number) in any single
run in physics on one apparatus.1
Quantum
turbulence: an unexpected surprise
For the past half century there have been hundreds of experiments
reported from the
What has changed all this is the initiation of a new form of turbulent flow created by a grid towed through a sample of stationary helium II. The towing of the grid (typically in a 1x1cm2 channel) provides a wide range of initial vorticities by simply towing at different speeds which can then be examined as the flow decays.3
The major surprise in these experiments was the unexpected classical behavior of the flow 4. Second sound sees only quantized vortex lines and just why they should mimic classical flows is a profound challenge. Evidently the quantized vortex lines tend to mimic the turbulence in the normal fluid. A deep discussion of this has been given by Joe Vinen.5.
![]()
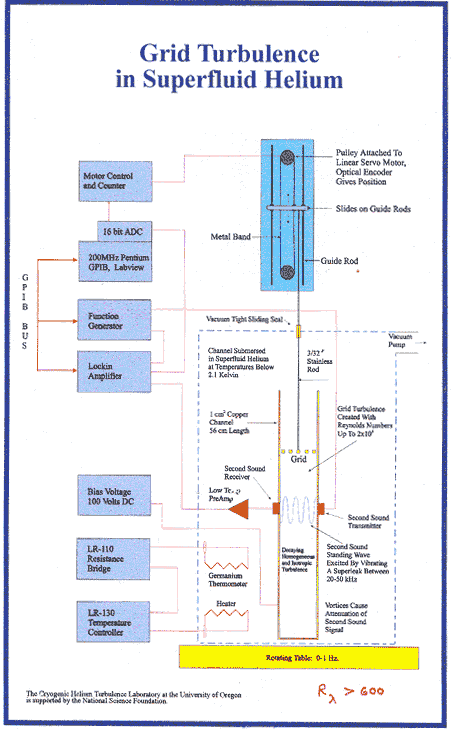
The
decay of turbulent quantized vorticity generated by a moving grid in superfluid
helium is shown below. The close correspondence with the classical decay
rate is both unmistakable and astounding.
![]()
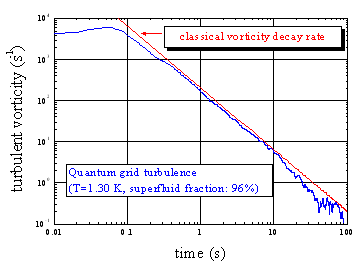
One of the most surprising things to arise in the theory of quantum turbulence is the question of just how turbulence decays below 1 K when no normal fluid is present. Viscosity does the job for ordinary fluids, but superfluid turbulence well below 1 K decays about the same as above 1 K. This is the subject of an extended theoretical, simulation and experimental investigation by Joe Vinen and colleagues in the UK and Japan.
Dissipation
in Turbulent Helium II
The dissipation per unit mass in a classical turbulent flow is of the form
![]()
where
![]() is the kinematic viscosity of the fluid and
is the kinematic viscosity of the fluid and
![]() is the mean square vorticity.
The question then for helium II is: what takes the place of
is the mean square vorticity.
The question then for helium II is: what takes the place of
![]() ? One choice favored for many years
is
? One choice favored for many years
is
![]() , where
, where
![]() is the viscosity and
is the viscosity and
![]() the total density of helium II
although the choice
the total density of helium II
although the choice
![]() seems even more logical. Thanks
to the towed grid experiment’s similarity in decay to classical fluids,
it is possible to deduce the actual value
seems even more logical. Thanks
to the towed grid experiment’s similarity in decay to classical fluids,
it is possible to deduce the actual value
![]() of the effective kinematic viscosity
of the two coupled fluids. The
results are shown below, and it is seen that
of the effective kinematic viscosity
of the two coupled fluids. The
results are shown below, and it is seen that
![]() is a completely new quantity in
quantum turbulence.6
is a completely new quantity in
quantum turbulence.6
Tow
tanks and wind tunnels
It has been known since the closing days of World War II that using low temperatures greatly improves the efficiency and ultimate Reynolds numbers of wind tunnels. Cooling raises the density and lowers the viscosity of air, leading to lower kinematic viscosity and hence higher Reynolds numbers.
Early studies in our group showed that a wind tunnel using liquid helium as a test fluid could be used to test submarine models at full Reynolds number appropriate to a nuclear submarine at full throttle.8. Tow tanks also have remarkable new possibilities 8.
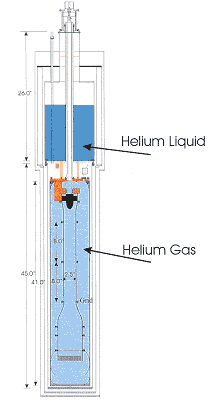
What we have come slowly to realize, however, is the tremendous flexibility in fluid properties offered by critical helium gas: something brought to our attention in the convection experiments discussed above. We have constructed a recirculating critical helium gas tunnel shown below. Our preliminary tests were at 6.5 K with mesh Reynolds numbers around 1500. The grid generated turbulence was probed with the 10 micron diameter hot wire described below. The hot wire obeyed King’s law relating velocity and voltage, and time series exhibited standard statistical features. One of the optimal operating points for high Re flow will be at 4 bar pressure and 6 K. Mesh Reynolds numbers of 30,000 to 100,000 will be available, and the corresponding microscale Reynolds numbers will range from 150 to 280. This first small step takes cryogenic wind tunnels down in temperature by more than a factor of 10 (from 77 K). The tunnel is illustrated in the figure below. The black object is a computer box fan (suggested by Roger Arndt) and the fan is driven by a magnetic coupling from outside.
![]()
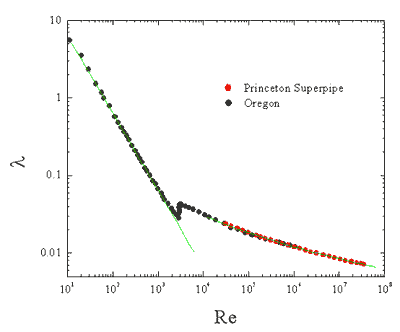
Pipeflow
We have
shown that an unusually small pipe flow apparatus using both liquid helium
and room temperature gases can span an enormous range of Reynolds numbers. This paper 9 describes the construction
and operation of the apparatus in some detail. A wide range of Reynolds numbers is an advantage
in any experiment seeking to establish scaling laws. Using a flow pipe
only 28 cm long and 0.4672 cm in diameter we were able to span a Reynolds
number range from 10 to 106 by taking advantage of the variation
in the kinematic viscosity of the fluids used.
Recently Lex Smit’s group at Princeton
University has used the (28 ton!) Superpipe to reach Reynolds number to
35 million using highly
![]()
Hot wire anemometers
Although
helium I is a Navier-Stokes fluid, the possibility of generating ever
higher Reynolds numbers carries with it ever decreasing Kolmogorov lengths.
For example in a 10 cm pipe characteristic lengths at the highest Reynolds
numbers could be less than 50 Angstroms.
Hot wire anemometers are resistive self-heating devices which balance
heat lost to flow, which depends on the fluid velocity. Calibration provides
the correlation between fluid velocity and electrical power supplied.
Standard hot wires have a diameter of order 5µm and length ~1000µm.
Standard materials (e.g. platinum) used at room temperature are insensitive
at low temperature.
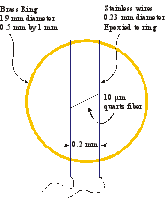
Our cryogenic hot wires anemometers are made on a quartz fiber of diameter
10 microns. They consist of an evaporated Au-Ge film of thickness of order
3000 Å. A small sensitive region in middle of the fiber is defined
by masking the fiber and evaporating a metallic film over it. Masking
is achieved by laying a small diameter fiber perpendicular to the first
fiber. The metal film provides electrical contact to the sensitive region.
The figure below shows how the small sensors are mounted. Sensor support
dimensions follow the “rule of 10”: they are placed ten times
(more or less) their characteristic dimension away from the sensitive
region. Electrical contact to the fiber is made through stainless steel
wires, which are isolated from the brass ring by epoxy. The fiber is fastened
to the wires using an electrically conducting epoxy.
![]()
PIV
One of
the most important goals we had was to find a was to implement Particle
Image Velocimetry (PIV). One reason
is that this widely used technique in fluid mechanics would probably work
in both helium I and helium II. The
simplest experiment we could think of was a variant on the towed grid
apparatus described above. A small
cryostat purchased from Janis Dewars was retrofitted
with a towed grid device as shown below.
The design and implementation of the cryogenics was done at Oregon
and transferred to Yale. There
Christopher White did a most interesting
dissertation on implementing PIV in cooperation from Dr. Adonios
Karpetis. The
details of this work are ably explained in White’s thesis, and demonstrate
beyond doubt that PIV will work in liquid helium.10
![]()

References
(These
are a few key papers. Full bibliographical
references are in our detailed papers.)
1.
"Turbulent Convection at High Rayleigh Numbers", J. J. Niemela,
L. Skrbek, K. R. Sreenivasan and R. J. Donnelly. Nature
404: 837-841 (2000).
2.
"Mutual Friction in a Heat Current in Liquid Helium II, I. Experiments
on Steady Heat Currents", W. F. Vinen. Proc. Roy. Soc. London(114-27)(1957).
3.
"Decay of Vorticity in Homogemeous Turbulence",
M. R. Smith, R. J. Donnelly, N. Goldenfeld and W. F. Vinen. Phys. Rev.
Lett. 71: 2538-2586 (1993).
4.
"Four Regimes of Decaying Turbulence in a Finite Channel", L.
Skrbek, J. J. Niemela and R. J. Donnelly. Phys.
Rev. Lett. 85: 2973-2976 (2000).
5.
"Classical character of turbulence in a quantum fluid", W. F.
Vinen. Physical Review B 61:
1410-1420 (2000).
6.
"Dissipation of Grid Turbulence in Helium II", S. Stalp,
J. J. Niemela, W. F. Vinen and R. J. Donnelly. Physics of Fluids
14: 1377-1379 (2002).
7."The
Flow About a Torsionally Oscillating Sphere", R. Hollerbach, R. J.
Wiener, I. S. Sullivan and R. J. Donnelly. Physics of Fluids 14:
4192-4205 (2002).
8
"Liquid and Gaseous Helium at Test Fluids", R. J. Donnelly in.
High Reynolds Number Flows Using Liquid and Gaseous Helium R. J. Donnelly,
Ed., New York, 1989. Springer-Verlag 284 (1991).
9.
"Pipe Flow Measurements over a Wide Range of Reynolds Numbers Using
Liquid Helium and Various Gases", C. J. Swanson, B. Julian, G. G.
Ihas and R. J. Donnelly. J. Fluid. Mech. 461: 51-60 (2002).
10.
“High Reynolds Number Turbulence in Small Apparatus”. C. M. White . PhD
Thesis, Mechanical Engineering, Yale University: 112pp.(2001)
