Taylor Vortex Flow

The Couette-Taylor problem concerns flow between independently rotating cylinders. It is a widely studied example of a system removed from equilibrium, in which the nonequilibrium condition is achieved by inducing mechanical stresses on the fluid through motion of the cylinder walls. The system, originally designed independently by Couette (1888) and Mallock (1888) for investigation of the viscous behavior of fluids, was the subject of a landmark work by G. 1. Taylor (1923). In his study, Taylor quantitatively predicted and experimentally confirmed the existence of a flow instability when the inner cylinder reaches a critical speed. For low cylinder speeds, the fluid simply moves azimuthally around the cylinders. Taylor observed that when this simple flow becomes unstable, it is replaced by a cellular pattern in which the fluid travels in helical paths around the cylinders in layers of vortices-now known as Taylor vortices (figs. 2,3).

Flow between rotating cylinders is remarkable for the fact that slow increase of the speed of the inner cylinder gives rise to a progression of clearly distinguishable fluid states that have increasing complexity. A series of transitions occurs in steps that may be arrested and studied independently, starting with the simplest, featureless flow and ending with fine-scale turbulence. This is to be contrasted with other fluid experiments, such as flow in boundary layers or between parallel plates, where turbulence arises-beyond a relatively low critical rate of flow- in a downstream spatial development of complexity. In such flows it is considerably more difficult to identify distinct stages of instability and transition.

A vivid illustration of the variety of flow patterns achieved in the Couette-Taylor system is the diagram from Anderek, Liu, and Swinney ( 1986) identifying flow states obtained by systematic variation of inner and outer cylinder speeds. A collection of excellent photographs of the various flow patterns may be found in the paper by Andereck et al. The boundaries separating the flow states depend, in many cases, on the exact protocol used in varying the parameters. This is an important signature of nonlinearity in fluid flow problems: the flow state depends on the history of its preparation. This was clearly established in an important paper by Coles (1965).

The sequence of transitions is also sensitive to the geometry, and in particular to the ratio of inner and outer cylinder radii. Andereck's diagram was produced for a radius ratio of 0.883. Surveys at other radius ratios, accompanied by sketches of the flow patterns in a cross-section of the gap between the cylinders, may be found in Snyder (1970). Such surveys have been done with relatively long cylinders, and the theory for such systems often assumes the length is infinite. In contrast, experiments can be done in short cylinders, where the length of the system becomes an additional parameter: see the reviews by Mullin (1991, 1993).

The Couette-Taylor problem is also characterized by experiments of very high precision. In this way, it is comparable to the problem of a horizontal layer of fluid subject to a destabilizing temperature gradient (the Rayleigh-Bénard problem). In many respects the Couette-Taylor problem is simpler because the flow starts out clearly anisotropic in the three principal directions (radial, azimuthal, and axial). This means there is less ambiguity about the kind of patterns that can form in the early stages of transition. Finally, the Couette-Taylor experiment is usually a closed system, meaning that no fluid flows into or out of it. However, this is not always the case, and experiments with flow in the axial direction have been carried out.

Tagg (1992) created a bibliography of papers in this subject about 1992 and found more than 1500 references! Remarking on the tremendous number of variants on this problem, Tagg called Couette-Taylor flow the “hydrogen atom” of fluid mechanics.

Taylor Couette flow has many variants. The first problem attempted by the Donnelly group was to see if the linear stability theory for the classical Taylor vortex problem could be made to work for the two-fluid theory of helium II. The importance of this problem is that the celebrated Taylor analysis settled the case for the validity of the Navier Stokes equation and the boundary conditions. The first attempt was by Chandrasekhar and Donnelly, but who got it wrong because the form of the equations of motion (called HVBK today) was still up in the air, and they neglected the term in vortex tension.

"The Hydrodynamic Stability of Helium II Between Rotating Cylinders, I", S. Chandrasekhar and R. J. Donnelly. Proc. Roy. Soc. A214: 9-28 (1958)

The first experiments on this subject were done by Donnelly in an early PRL:

"Experiments on the Hydrodynamic Stability of Helium II Between Rotating Cylinders", R. J. Donnelly. Phys. Rev. Lett. 3: 507-508 (1959).

The apparatus (at the University of Chicago) is shown below.

Chandrasekhar also worked on the stability of hydromagnetic flows, in particular Taylor-Couette flow of a conducting fluid in a magnetic field (see Chandra’s remarkable book Hydrodynamic and Hydromagnetic Stability, Oxford, 1961). Our group undertook to verify these calculations using the hydromagnetic Couette viscometer shown below, with magnetic field furnished by an old cyclotron magnet.

The results were published in 1962:

"Experiments on the Stability of Flow Between Rotating Cylinders in the Presence of a Magnetic Field", R. J. Donnelly and M. Ozima. Proc. Roy. Soc. A266: 272-286 (1962).

The experiment in place is shown below. This cyclotron now sits beside the entrance drive to Fermilab.

"Experiments on the Stability of Viscous Flow Between Rotating Cylinders IV. The Ion Technique", R. J. Donnelly. Proc. Roy. Soc. A283: 509-519 (1965).

The principal of obtaining scans of the vortex patterns is shown below:

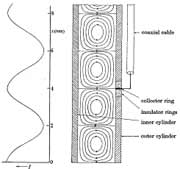
This technique allowed Donnelly and Schwarz to verify the “Landau Law” for the growth of equilibrium amplitude of vortex motion above critical:

and to study in detail the transient response to a sudden transition through the critical speed:



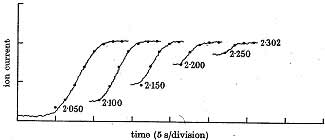
"Experiments on the Stability of Viscous Flow Between Rotating Cylinders VI. Finite Amplitude Experiments", R. J. Donnelly and K. W. Schwarz. Proc. Roy. Soc. A283: 531-556 (1965). The relevant theory is discussed in Chapter 3 of Landau and Lifshitz Fluid Mechanics, Second edition

Acting on a suggestion by Fred Reif and Harry Suhl, we began an investigation into the stability of modulated Taylor Vortex flow. In the days before stepping motors we had to use one Graham variable speed drive to modulate the speed control of another:

"Enhancement of Hydrodynamic Stability by Modulation", R. J. Donnelly, F. Reif and H. Suhl. Phys. Rev. Lett. 9: 363-365 (1962).

This problem lasted for many years with various twists and turns, and even was extended to Rayleigh Bénard convection. A review article summarizes the present situation briefly:

"Externally Modulated Hydrodynamic Systems", R. J. Donnelly in. Nonlinear Evolution of Spatio-temporal Structures in Dissipative Continuous Systems F. Busse, ed., Streitberg (Bayreuth) Germany,1990.

Russell Donnelly and Dave Fultz constructed a large rotating cylinder pair at the University of Chicago. This is shown in the photograph below taken in 1961, with Sir Geoffrey Taylor and Dave Fultz. Many of the visual experiments are described in Chandrasekhar’s 1961 stability book. A photo of Chandra the day the book was received is shown below.