In order to learn anything about the composition, formation and evolution of stars, one must first determine their distances. For example, to determine the absolute luminosity of a star (the amount of energy emitted per second) you measure its apparent brightness (its luminosity from the Earth), then multiple by the distance from the Earth squared.

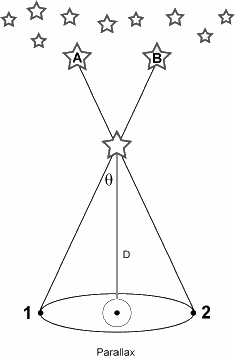
The most direct method to measure the distance to nearby stars is through the use of parallax. The Earth's motion around the Sun every year produces a very small shift in nearby star's position in the sky compared to distant, background stars. This shift is always less than one arcsec for any star, which is very small (where a circle is 360 degrees, one degree is 60 arcminutes, one arcminute is 60 arcsecs).

The parallax of a star is used to calculate its distance. The angle of the parallax, θ, in arcsecs is equal to the distance, D, by the following formula:

here the distance, D, is measured in parsecs, where one parsec equals 3.26 light-years (the distance light travels in one year).

Proper Motion:

Although the stars appear fixed in the sky, they are actually moving through space at very high velocities. Their extremely large distances makes this motion almost undetectable. This motion is called proper motion, and can also be used to judge the distance to stars.

The nearest stars should display the greatest proper motion, and the star with the greatest proper motion is Barnard's star with a change of 10 arcsecs per year. Since only a few stars have proper motions greater than 1 arcsec per year, thus, the shape of the constellations have remained mostly unchanged since the dawn of man. The diagram below shows how the Big Dipper will look after 10,000 years.

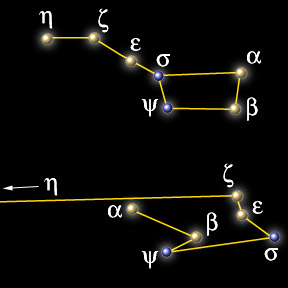
Notice also that the Sun is moving through the Galaxy, so solar motion will give the appearance of stars in front of our motion moving towards an apex (like a car driving through snowflakes). Those behind us will appear to move towards an antapex.

Stellar Magnitudes:

The measure of the brightness of a star is, for historical and physiological reasons, called its apparent magnitude. The human eye detects light in a logarithmic fashion, meaning that changes occur in powers of 10 rather than in a linear manner. So ancient astronomers divided stars into six classes or magnitudes where the brightest are first magnitude, the faintest are sixth magnitude. Later measurements showed that a change in 5 magnitudes is equal to a 100 increase in brightness.

Here is a list of common objects in the sky and their magnitudes. Note that the greater the magnitude (the more positive the number), the fainter the star. Negative numbers are bright stars.

Object           Apparent Mag
-----------------------------
Sun                  -26.5
Full Moon            -12.5
Venus                 -4.0
Jupiter               -3.0
Sirius                -1.4
Polaris                2.0
eye limit              6.0
Pluto                 15.0
limit for telescopes  25.0
----------------------------

We also distinguish between apparent magnitude and absolute magnitude. Apparent magnitude is what we measure in the sky, absolute magnitude is the real luminosity of the star, corrected for distance.

The magnitude system works out such that a change in 1 in magnitude corresponds to a change in 2.512 in brightness. The formula is as follows:

where b₁ and b₂ are the brightnesses of two stars (ergs per sec) and m₁ and m₂ are the magnitudes of the two stars. If you know the brightness of the stars, and want to determine their relative magnitudes, this formula is the inverse of the one above:

If the apparent magnitude of a star is m and its absolute magnitude is M (its real brightness), then the distance to the star, d in parsecs, is given by:

Solar Neighborhood:

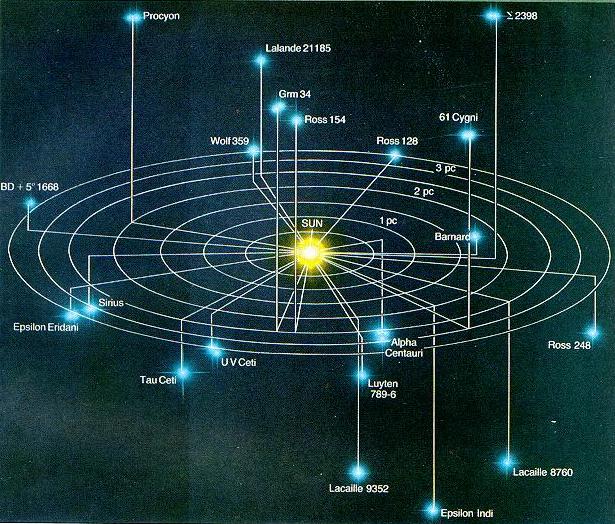
Stars have different absolute luminosities. So the brightest stars in the sky are not necessarily the closest stars. Here is a list of the twenty brightest stars in the sky. And here is a list of the twenty nearest stars.

The nearest stars make-up what is called the solar neighborhood, shown below. Note that the nearest stars are mostly small dim stars. These types of stars are hard to see at great distances. The twenty brightest stars are mostly supergiant stars; which are rare, but very bright.

Stellar Masses:

Since stars are so far away, it is impossible to measure their masses directly. Instead, we look for binary star systems and use Newton's law of gravity to measure their masses.

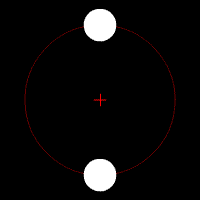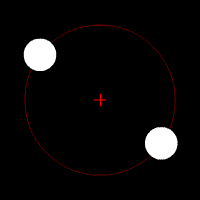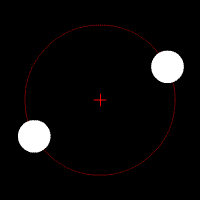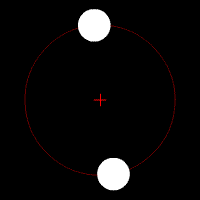
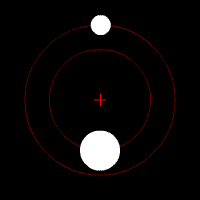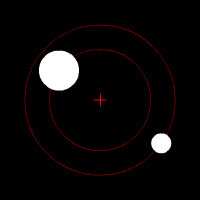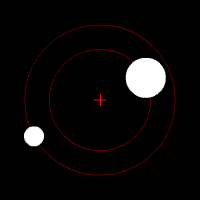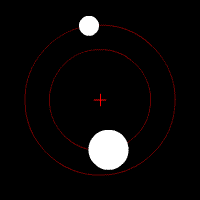
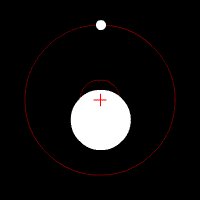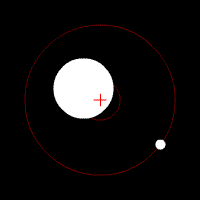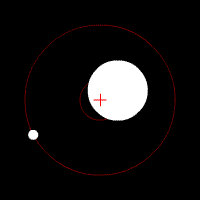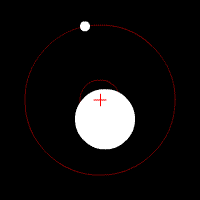
Two stars in a binary system are bound by gravity and revolve around a common center of mass. Kepler's 3rd law of planetary motion can be used to determine the sum of the mass of the binary stars if the distance between each other and their orbital period is known.

Kepler's 3rd law states that the square of a planet's or star's orbital period is proportional to its mean distance from each other such that:

where P is the orbital period in years and r is the distance between each other in Astronomical Units (the distance from the Earth to the Sun). The constant, k, is derived from Newton's law of gravity to be the sum of the masses of the stars, M₁ + M₂, in units of solar masses. So the full equation becomes:

When you plot the mass of a star versus its absolute luminosity, one finds a correlation between the two quantities shown below.

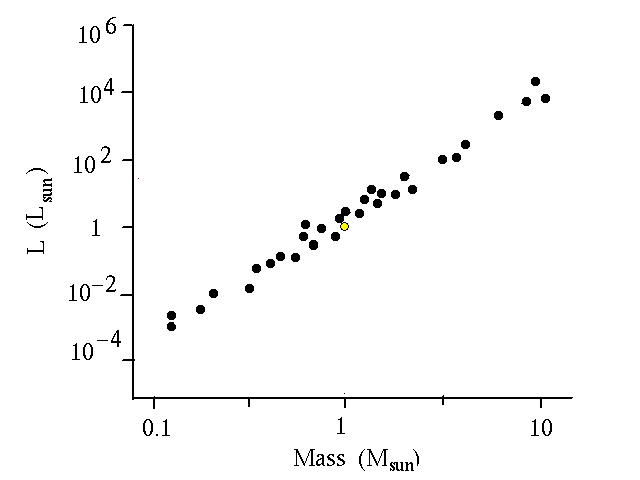
This relationship is called the mass-luminosity relation for stars, and it indicates that the mass of a star controls the rate of energy production, which is thermonuclear fusion in the star's core. The rate of energy generation, in turn, uniquely determines the stars total luminosity. Note that this relation only applies to stars before they evolve into giant stars (those stars which burn hydrogen in their core).

Notice that stars range in mass from about 0.08 to 100 times the mass of the Sun. The lower mass limit is set by the internal pressures and temperatures needed to start thermonuclear fusion (protostars too low in mass never beginning fusion and do not become stars). The upper limit is set by the fact that stars of mass higher than 100 solar masses become unstable and explode. Notice also that these range of masses corresponds to a luminosity range from 0.0001 to 10⁵ solar luminosities.