Essentials of Physics- PHYS 101
Lecture 4
![]()
Please get an acrylic half-dome for solar observations. Solar observations are to be recorded for 2 different, sunny days. Try to complete the first day's observations as soon as possible. Separate your observations by at least two weeks, if not more. Use the same dome for both sets of observations. Mark the domes with different colored pens to denote each day.
Observations, including the completed instruction sheet and dome, are due at the beginning of Lab 6, on May 6th or 7th.
![]()
![]()
We can test this using our old friends the cart, track, motion detector and, introducing the fabulous hanging mass
![]()
1) You can always multiply by 1
Example: 1.6 km = 1 mile. 1.6 km / 1 mile = 1. To convert miles to km, I multiply by 1:
2) Multiply both sides of an equation by the same thing (number):
Say we know that SF = m a
Say we also know a and SF. How do I solve for m?
Multiply both sides of equation, above by the same thing (1/a):
![]() More on the relationship between a, m, and F
More on the relationship between a, m, and F
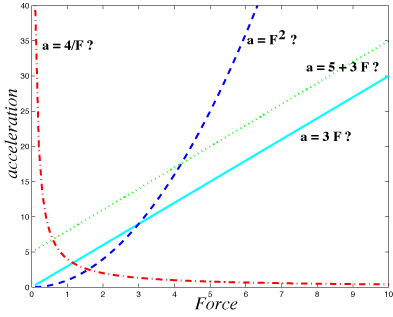
Last week we observed that the acceleration of a cart doubled when the force pulling it was doubled. More generally we found that acceleration is proportional to the net force.
a µ F
What about mass?
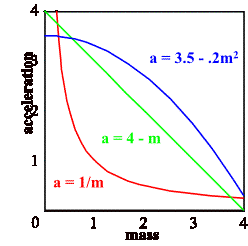 |
We know mass gives an object inertia which resists a change in motion. Another way to say this is more mass makes an object harder to accelerate.
What is the exact relationship between acceleration and mass?
In lab you kept the pulling force constant but changed the mass of the car. What you found (hopefully) was that if the mass of the car doubled the acceleration was reduced by half.
a µ 1/m
'acceleration is inversely proportional to mass'
put all this together to get--
Put this together with a µ F and what do we get?
a = Fnet/m more commonly written as: Fnet = ma !
![]()
| Let's observe the effect of "gravity" on a softball with the following experimental setup: | 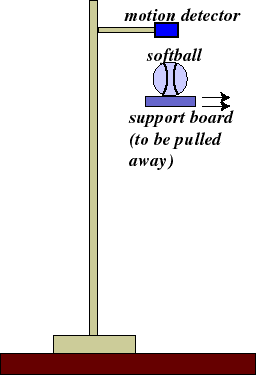 |
Question: What happens when the board is pulled out from under the softball?
Answer: Duh, let me guess.....
Question: So, HOW is the ball moving towards the ground?
Time for an
Question: If the ball is accelerating, what is causing the acceleration?
Answer: The "force of gravity," the attraction between the Earth and the softball, is causing the ball to accelerate downwards.
Question: Is the "force of gravity" the same for lighter or heavier (less or more massive) objects of a similar shape?
| Try this experiment: | 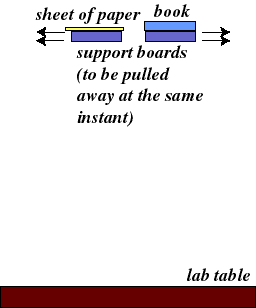 |
Answer: OK, so they both hit at the same time (after suitable modification). That means that they both accelerated in an identical manner, speeding up towards the table at the same rate.
![]()