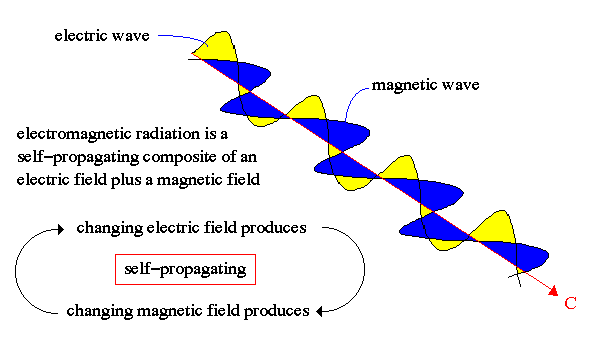
Electromagnetic Radiation (a.k.a. Light):
Roemer (1680's) was the first to measure the speed of light using Jupiter's moons -> c=299,790 km/sec or about 185,000 mi/sec
Maxwell (1850's) showed that light is energy carried in the form of opposite but supporting electric and magnetic fields in the shape of waves, i.e. self-propagating electromagnetic waves.


Inverse Square Law:
The brightness of an object varies inversely as the square of the distance. This means that objects farther away are dimmer.
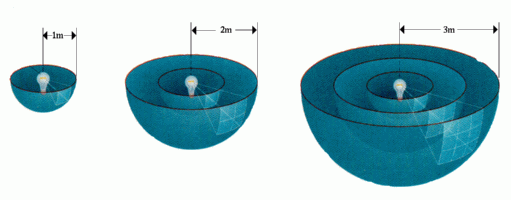
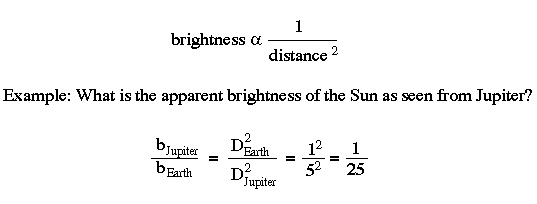
But notice that the dimming does not progress in a linear fashion (i.e. 1, 2, 3, 4 ...) but rather in an inverse square (i.e. 1/2, 1/4, 1/8, 1/16 ...).
Doppler effect:
The Doppler effect occurs when an object that is emitting light is in motion with respect to the observer. If the object is moving towards the observer the light is ``compressed'', meaning that the wavelength of the light becomes smaller. Smaller wavelength means bluer light, so we say the object is blueshifted. If the object is moving away from the observer the light is ``expanded'', the wavelength is increased or redshifted.
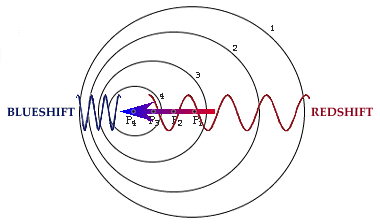
Notice that the speed of light does not change, only the wavelength. It is a basic premise of the theory of relativity that the velocity of light never changes regardless of the motion of the observer.
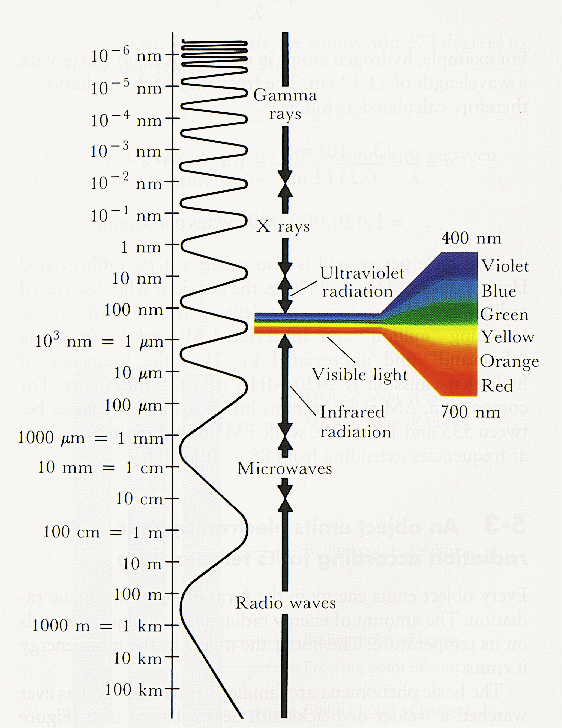
Electromagnetic radiation is expressed in either wavelength, λ in centimeters, or frequency, ν in Hertz (sec-1). The relationship between wavelength and frequency is
where c is the speed of light, 3x1010 cm/sec.
The Doppler effect relates a change in wavelength, Δλ, to the original wavelength, λ, and the velocity of the source, v, such that
For example, the change in wavelength for a 50 cm radio wave from a car moving at 3000 cm/sec (about 45 mph) is
Δλ = 5x10-6 cm
a very small change.
Planck's curve:
One of the primary results from the field of spectroscopy was the discovery of how the spectrum of an object changes with temperature. In particular, was the formulation of the two laws of radiation:

Planck's constant:
In the early 1900's, German physicist E. Planck noticed fatal flaw in our physics by demonstrating that the electron in orbit around the nucleus accelerates. Acceleration means a changing electric field (the electron has charge), when means photons should be emitted. But, then the electron would lose energy and fall into the nucleus. Therefore, atoms shouldn't exist!
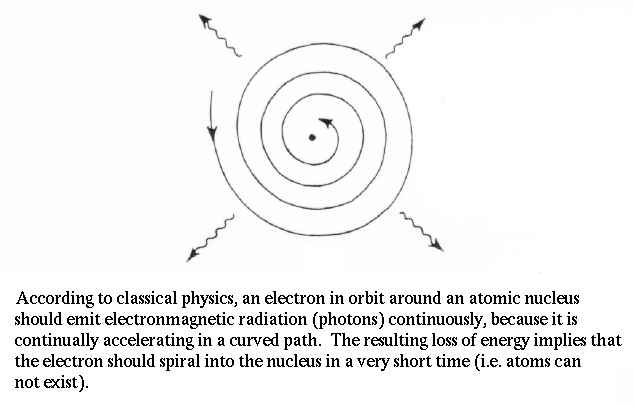
To resolve this problem, Planck made a wild assumption that energy, at the sub-atomic level, can only be transfered in small units, called quanta. Due to his insight, we call this unit Planck's constant (h). The word quantum derives from quantity and refers to a small packet of action or process, the smallest unit of either that can be associated with a single event in the microscopic world.

Changes of energy, such as the transition of an electron from one orbit to another around the nucleus of an atom, is done in discrete quanta. Quanta are not divisible and the term quantum leap refers to the abrupt movement from one discrete energy level to another, with no smooth transition. There is no ``inbetween''.
The quantization, or ``jumpiness'' of action as depicted in quantum physics differs sharply from classical physics which represented motion as smooth, continuous change. Quantization limits the energy to be transfered to photons and resolves the UV catastrophe problem.
Wave-Particle Dualism:
The wave-like nature of light explains most of its properties:
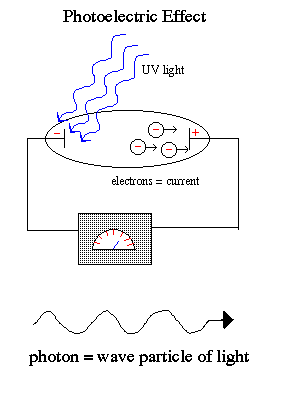
This dualism to the nature of light is best demonstrated by the photoelectric effect, where a weak UV light produces a current flow (releases electrons) but a strong red light does not release electrons no matter how intense the red light.

Einstein explained the photoelectric effect by assuming that light exists in a particle-like state, packets of energy (quanta) called photons. There is no current flow for red light because the packets of energy carried by each individual red photons are too weak to knock the electrons off the atoms no matter how many red photons you beamed onto the cathode. But the individual UV photons were each strong enough to release the electron and cause a current flow.
It is one of the strange, but fundamental, concepts in modern physics that light has both a wave and particle state (but not at the same time), called wave-particle dualism.
de Broglie Matter Waves:
Perhaps one of the key questions when Einstein offered his photon description of light is, does an electron have wave-like properties? The response to this question arrived from the Ph.D. thesis of Louis de Broglie in 1923. de Broglie argued that since light can display wave and particle properties, then matter can also be a particle and a wave too.

One way of thinking of a matter wave (or a photon) is to think of a wave packet. Normal waves look with this:
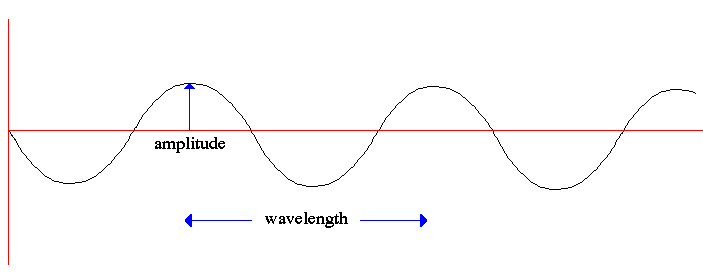
having no beginning and no end. A composition of several waves of different wavelength can produce a wave packet that looks like this:
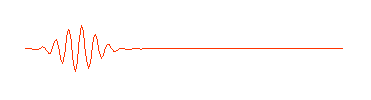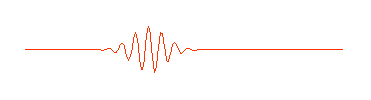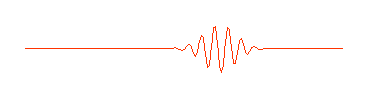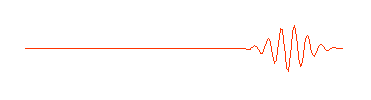
So a photon, or a free moving electron, can be thought of as a wave packet, having both wave-like properties and also the single position and size we associate with a particle. There are some slight problems, such as the wave packet doesn't really stop at a finite distance from its peak, it also goes on for every and every. Does this mean an electron exists at all places in its trajectory?
de Broglie also produced a simple formula that the wavelength of a matter particle is related to the momentum of the particle. So energy is also connected to the wave property of matter.
While de Broglie waves were difficult to accept after centuries of thinking of particles are solid things with definite size and positions, electron waves were confirmed in the laboratory by running electron beams through slits and demonstrating that interference patterns formed.
How does the de Broglie idea fit into the macroscopic world? The length of the wave diminishes in proportion to the momentum of the object. So the greater the mass of the object involved, the shorter the waves. The wavelength of a person, for example, is only one millionth of a centimeter, much to short to be measured. This is why people don't `tunnel' through chairs when they sit down.
Uncertainty Principle:
Classical physics was on loose footing with problems of wave/particle duality, but was caught completely off-guard with the discovery of the uncertainty principle.

The uncertainty principle, developed by W. Heisenberg, is a statement of the effects of wave-particle duality on the properties of subatomic objects. Consider the concept of momentum in the wave-like microscopic world. The momentum of wave is given by its wavelength. A wave packet like a photon or electron is a composite of many waves. Therefore, it must be made of many momentums. But how can an object have many momentums?
Of course, once a measurement of the particle is made, a single momentum is observed. But, like fuzzy position, momentum before the observation is intrinsically uncertain. This is what is know as the uncertainty principle, that certain quantities, such as position, energy and time, are unknown, except by probabilities. In its purest form, the uncertainty principle states that accurate knowledge of complementarity pairs is impossible. For example, you can measure the location of an electron, but not its momentum (energy) at the same time.

Mathematically we describe the uncertainty principle as the following, where `x' is position and `p' is momentum:
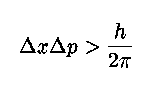
This is perhaps the most famous equation next to E=mc2 in physics. It basically says that the combination of the error in position times the error in momentum must always be greater than Planck's constant. So, you can measure the position of an electron to some accuracy, but then its momentum will be inside a very large range of values. Likewise, you can measure the momentum precisely, but then its position is unknown.
Also notice that the uncertainty principle is unimportant to macroscopic objects since Planck's constant, h, is so small (10-34). For example, the uncertainty in position of a thrown baseball is 10-30 millimeters.
The depth of the uncertainty principle is realized when we ask the question; is our knowledge of reality unlimited? The answer is no, because the uncertainty principle states that there is a built-in uncertainty, indeterminacy, unpredictability to Nature.
Quantum Wave Function:
The wave nature of the microscopic world makes the concept of `position' difficult for subatomic particles. Even a wave packet has some `fuzziness' associated with it. An electron in orbit has no position to speak of, other than it is somewhere in its orbit.
To deal with this problem, quantum physics developed the tool of the quantum wave function as a mathematical description of the superpositions associated with a quantum entity at any particular moment.

The key point to the wave function is that the position of a particle is only expressed as a likelihood or probability until a measurement is made. For example, striking an electron with a photon results in a position measurement and we say that the wave function has `collapsed' (i.e. the wave nature of the electron converted to a particle nature).
|
Superposition:
|
Readings:
Antimatter |
The fact that quantum systems, such as electrons and protons, have indeterminate aspects means they exist as possibilities rather than actualities. This gives them the property of being things that might be or might happen, rather than things that are. This is in sharp contrast to Newtonian physics where things are or are not, there is no uncertainty except those imposed by poor data or limitations of the data gathering equipment.
The superposition of possible positions for an electron can be demonstrated by the observed phenomenon called quantum tunneling.
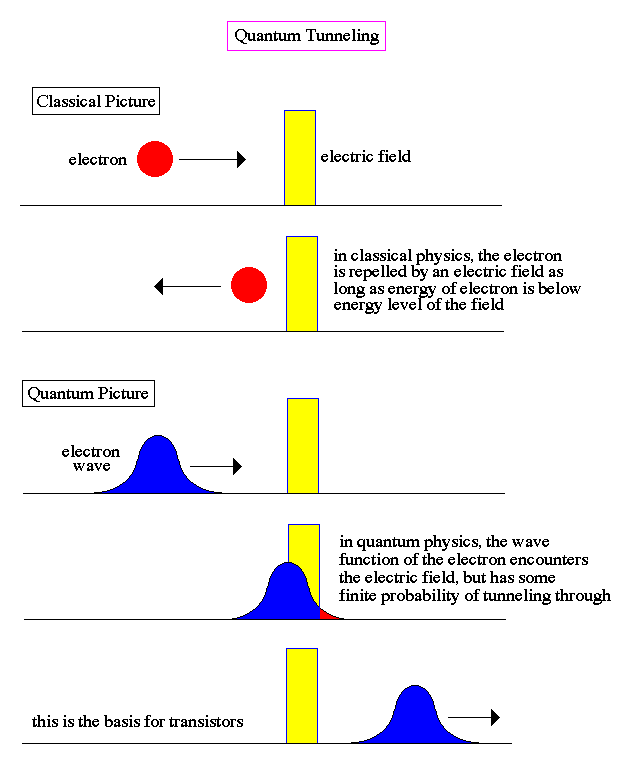
Notice that the only explanation for quantum tunneling is if the position of the electron is truly spread out, not just hidden or unmeasured. It raw uncertainty allows for the wave function to penetrate the barrier. This is genuine indeterminism, not simply an unknown quantity until someone measures it.
It is important to note that the superposition of possibilities only occurs before the entity is observed. Once an observation is made (a position is measured, a mass is determined, a velocity is detected) then the superposition converts to an actual. Or, in quantum language, we say the wave function has collapsed.
The collapse of the wave function by observation is a transition from the many to the one, from possibility to actuality. The identity and existence of a quantum entities are bound up with its overall environment (this is called contextualism). Like homonyms, words that depend on the context in which they are used, quantum reality shifts its nature according to its surroundings.
Bohr Atom:
Perhaps the foremost scientists of the 20th century was Niels Bohr, the first to apply Planck's quantum idea to problems in atomic physics. In the early 1900's, Bohr proposed a quantum mechanical description of the atom to replace the early model of Rutherford.

The Bohr model basically assigned discrete orbits for the electron, multiples of Planck's constant, rather than allowing a continuum of energies as allowed by classical physics.
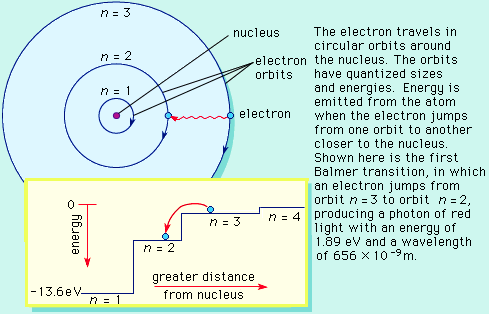
The power in the Bohr model was its ability to predict the spectra of light emitted by atoms. In particular, its ability to explain the spectral lines of atoms as the absorption and emission of photons by the electrons in quantized orbits.

In principle, all of atomic and molecular physics, including the structure of atoms and their dynamics, the periodic table of elements and their chemical behavior, as well as the spectroscopic, electrical, and other physical properties of atoms and molecules, can be accounted for by quantum mechanics => fundamental science.
Quantum Mechanics:
The field of quantum mechanics concerns the description of phenomenon on small scales where classical physics breaks down. The biggest difference between the classical and microscopic realm, is that the quantum world can be not be perceived directly, but rather through the use of instruments. And a key assumption to quantum physics is that quantum mechanical principles must reduce to Newtonian principles at the macroscopic level (there is a continuity between quantum and Newtonian mechanics).
Quantum mechanics uses the philosophical problem of wave/particle duality to provide an elegant explanation to quantized orbits around the atom. Consider what a wave looks like around an orbit, as shown below.

Only certain wavelengths of an electron matter wave will `fit' into an orbit. If the wavelength is longer or shorter, then the ends do not connect. Thus, de Broglie matter waves explain the Bohr atom such that on certain orbits can exist to match the natural wavelength of the electron. If an electron is in some sense a wave, then in order to fit into an orbit around a nucleus, the size of the orbit must correspond to a whole number of wavelengths.
Notice also that this means the electron does not exist at one single spot in its orbit, it has a wave nature and exists at all places in the allowed orbit (the uncertainity principle). Thus, a physicist speaks of allowed orbits and allowed transitions to produce particular photons (that make up the fingerprint pattern of spectral lines).
Quantum mechanics was capable of bringing order to the uncertainty of the microscopic world by treatment of the wave function with new mathematics. Key to this idea was the fact that relative probabilities of different possible states are still determined by laws. Thus, there is a difference between the role of chance in quantum mechanics and the unrestricted chaos of a lawless Universe.
The quantum description of reality is objective (weak form) in the sense that everyone armed with a quantum physics education can do the same experiments and come to the same conclusions. Strong objectivity, as in classical physics, requires that the picture of the world yielded by the sum total of all experimental results to be not just a picture or model, but identical with the objective world, something that exists outside of us and prior to any measurement we might have of it. Quantum physics does not have this characteristic due to its built-in indeterminacy.
For centuries, scientists have gotten used to the idea that something like strong objectivity is the foundation of knowledge. So much so that we have come to believe that it is an essential part of the scientific method and that without this most solid kind of objectivity science would be pointless and arbitrary. However, quantum physics denies that there is any such thing as a true and unambiguous reality at the bottom of everything. Reality is what you measure it to be, and no more. No matter how uncomfortable science is with this viewpoint, quantum physics is extremely accurate and is the foundation of modern physics (perhaps then an objective view of reality is not essential to the conduct of physics). And concepts, such as cause and effect, survive only as a consequence of the collective behavior of large quantum systems.
Antimatter:
A combination of quantum mechanics and relativity allows us to examine subatomic processes in a new light. Symmetry is very important to physical theories. For example, conservation of momemtum is required for symmetry in time. Thus, the existence of a type of `opposite' matter was hypothesized soon after the development of quantum physics. `Opposite' matter is called antimatter. Particles of antimatter has the same mass and characteristics of regular matter, but opposite in charge. When matter and antimatter come in contact they are both instantaneously converted into pure energy, in the form of photons.
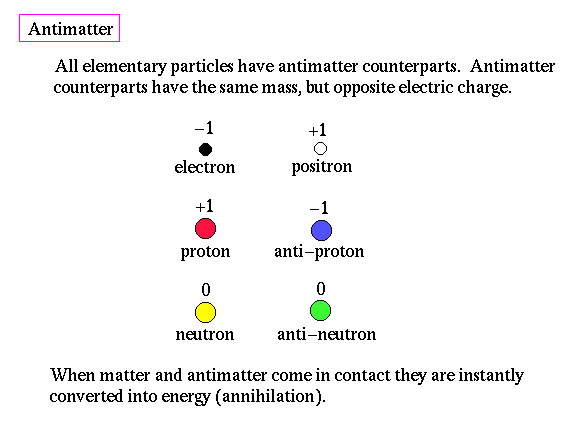
Antimatter is produced all the time by the collision of high energy photons, a process called pair production, where an electron and its antimatter twin (the positron) are created from energy (E=mc2).
Fission/Fusion:
One of the surprising results of quantum physics is that if a physical event is not specifically forbidden by a quantum rule, than it can and will happen. While this may strange, it is a direct result of the uncertainty principle. Things that are strict laws in the macroscopic world, such as the conversation of mass and energy, can be broken in the quantum world with the caveat that they can only broken for very small intervals of time (less than a Planck time). The violation of conservation laws led to the one of the greatest breakthroughs of the early 20th century, the understanding of radioactivity decay (fission) and the source of the power in stars (fusion).
Nuclear fission is the breakdown of large atomic nuclei into smaller elements. This can happen spontaneously (radioactive decay) or induced by the collision with a free neutron. Spontaneously fission is due to the fact that the wave function of a large nuclei is 'fuzzier' than the wave function of a small particle like the alpha particle. The uncertainty principle states that, sometimes, an alpha particle (2 protons and 2 neutrons) can tunnel outside the nucleus and escape.
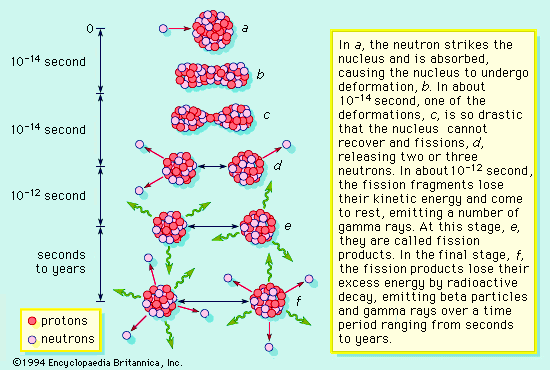
Induced fission occurs when a free neutron strikes a nucleus and deforms it. Under classical physics, the nucleus would just reform. However, under quantum physics there is a finite probability that the deformed nucleus will tunnel into two new nuclei and release some neutrons in the process, to produce a chain reaction.
Fusion is the production of heavier elements by the fusing of lighter elements. The process requires high temperatures in order to produce sufficiently high velocities for the two light elements to overcome each others electrostatic barriers.
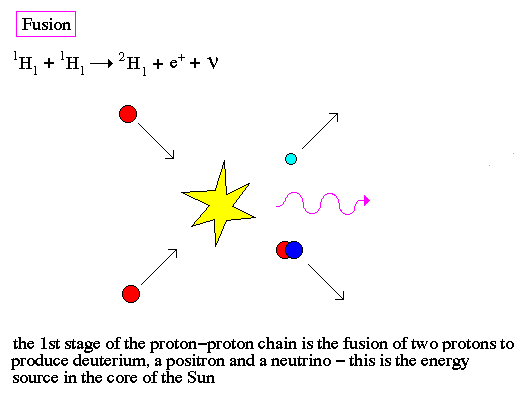
Even for the high temperatures in the center of a star, fusion requires the quantum tunneling of a neutron or proton to overcome the repulsive electrostatic forces of an atomic nuclei. Notice that both fission and fusion release energy by converting some of the nuclear mass into gamma-rays, this is the famous formulation by Einstein that E=mc2.
Although it deals with probabilities and uncertainties, the quantum mechanics has been spectacularly successful in explaining otherwise inaccessible atomic phenomena and in meeting every experimental test. Its predictions are the most precise and the best checked of any in physics; some of them have been tested and found accurate to better than one part per billion.
Holism:
This is the holistic nature of the quantum world, with the behavior of individual particles being shaped into a pattern by something that cannot be explained in terms of the Newtonian reductionist paradigm. Newtonian physics is reductionistic, quantum physics is holistic.
Where a reductionist believes that any whole can be broken down or analyzed into its separate parts and the relationships between them, the holist maintains that the whole is primary and often greater than the sum of its parts. Nothing can be wholly reduced to the sum of its parts.

The atom theory of the Greeks viewed the Universe as consists of indestructible atoms. Change is a rearrangement of these atoms. An earlier holism of Parmenides argued that at some primary level the world is a changeless unity, indivisible and wholly continuous.
The highest development of quantum theory returns to the philosophy of Parmenides by describing all of existence as an excitation of the underlying quantum vacuum, like ripples on a universal pond. The substratum of all is the quantum vacuum, similar to Buddhist idea of permanent identity.
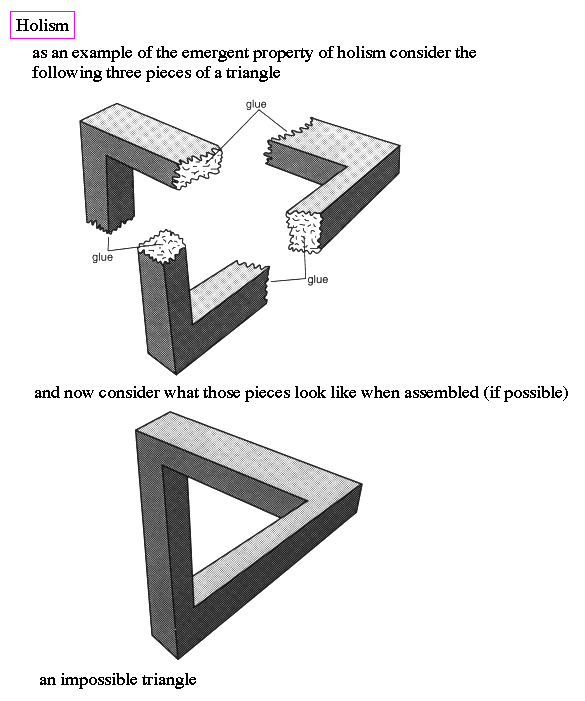
Quantum reality is a bizarre world of both/and, whereas macroscopic world is ruled by either/or. The most outstanding problem in modern physics is to explain how the both/and is converted to either/or during the act of observation.
Note that since there are most probable positions and energy associated with the wave function, then there is some reductionism available for the observer. The truth is somewhere between Newton and Parmenides.

|
|

|