Peter B Gilkey
email: gilkey@uoregon.edu
Research Articles
[1] Curvature and the eigenvalues of the Laplacian for elliptic complexes,
Advances in Math, V 10 # 3 (1973), 344-382.
[2] Curvature and the eigenvalues of the Dolbeault complex for Kaehler
manifolds, Advances in Math, V 11 # 3 (1973), 311-325
[3] Local invariants of a Riemannian metric for two dimensional manifolds,
Indiana Univ Math J, V 23 # 9 (1974), 855-882.
[4] Spectral geometry and the Kaehler condition for complex manifolds,
Invent. Math, V 26 (1974), 231-258. A Correction was subsequently posted
in V 29 (1975) 81-82 to correct an error found by Prof. T. Tsujishita
[5] Spectral geometry of real and complex manifolds, Proc.Symp. in Pure
Math, V 27 (1975), 265-280.
[6] Spectral geometry and manifolds of constant holomorphic sectional curvature,
Proc. Symp. in Pure Math, V 27 (1975), 281-285 (joint work with Jon Sacks).
[7] The boundary integrand in the formula for the signature and Euler characteristic
of a Riemannian manifold with boundary, Advances in Math, V 15 # 3 (1975),
334-360.
[8] The spectral geometry of a Riemannian manifold, J.Diff.Geo. V 10 #
4 (1975), 601-618.
[9] The index theorem and the heat equation, Publish or Perish Press, Boston
Mass (1974), 125pp.
[10] Local invariants of an embedded Riemannian manifold, Annals of Math.
V 102 (1975), 187-203.
[11] Local invariants of a pseudo - Riemannian manifold, Math. Scand. 36
(1975), 109-130.
[12] Curvature and the eigenvalues of the Dolbeault complex for Hermitian
manifolds, Advances in Math. V 21 # 1 (1976), 61-77.
[13] The spectral geometry of symmetric spaces, Trans.AMS V 225 (1977),
341-353.
[14] Heat equation, proportionality principle, and the volume of fundamental
domains, Differential Geometry and Relativity, ed Cahen and Flato, D.Reidel
Publ.Co. 1976, 43-54. (Joint work with R.Cahn and J.Wolf).
[15] Local invariants of real and complex Riemannian manifolds, Proc.Symp.
in Pure Math. V 30 (1977), 107-110.
[16] Smooth invariants of a Riemannian manifold, Advances in Math. V 28
# 1 (1978), 1-10.
[17] Lefschetz fixed point formulas and the heat equation, Partial Differential
Equations and Geometry (Proc.Park City Conf). Ed C.Byrnes. Marcel Dekker
(1979), 91-147.
[18] Recursion relations and the asymptotic behavior of the eigenvalues
of the Laplacian. Compositio Mathematica, V 38 # 2 (1979), 201-240.
[19] Topological invariants and absence of an axial anomaly for a Euclidean
Taub-NUT metric, Physical Review D, V 17 # 2 (1978), 423-427. (joint with
T.Eguchi and A.Hanson).
[20] Curvature and the heat equation for the DeRham complex. Geometry and
Analysis (Papers dedicated to the memory of V.K.Patodi). Indian Academy
of Sciences (1979), 47-80.
[21] The residue of the local eta function at the origin, Math.Annalen,
V 240 (1979), 183-189.
[22] The residue of the global eta function at the origin, Advances in
Math., V 40 3 (1981), 290-307.
[23] The spectral geometry of the higher order Laplacian, Duke Math.J.
V 47 3 (1980), 511-528. (Correction in V 48 4 p 887).
[24] Gravitation, gauge theories and differential geometry, Review Section
of Physics Letters, North Holland Publishing Company (Amsterdam), V 66
6 (1980), 212-393. (Joint work with T.Eguchi and A.Hanson).
[25] The eta invariant for a class of elliptic boundary value problems.
Comm on Pure and Appl Math V 36 (1983), 85-131. (joint work with Lance
Smith).
[26] The twisted index theorem for manifolds with boundary. J.Diff.Geo.
18 (1983), 393-444. (Joint work with Lance Smith)
[27] Invariance theory, the heat equation, and the Atiyah-Singer index
theorem , Publish or Perish Press, 1985, 349pp. Available on the web: Published
on the EMIS server
http://www.emis.de/monographs/gilkey/index.html
ISBN 0-914098-20-9. See also PDF
file provided by Mirko Janc
[28] The secondary characteristic classes of locally flat bundles on lens
spaces in dimension 3, Differential Geometric Control Theory, ed Brockett,
Millman, Sussman (Birkhauser 1983), 237-258.
[29] Vector bundles with harmonic curvatures over spheres, Differential
Geometry, ed Brooks, Gray, Reinhart (Birkhauser 1983), 89-102.
[30] Invariants of the heat equation . Pacific Journal of Mathematics V
117 (1985), 233-254 (joint with H.Fegan).
[31] The eta invariant and the K-theory of odd dimensional spherical space
forms. Invent.Math. 76 (1984), 421-453.
[32] The eta invariant of even dimensional Pinc manifolds, Advances in
Math. V 58 3 (1985), 243-284.
[33] The eta invariant and secondary characteristic classes of locally
flat bundles, Algebraic and Differential Topology- Global Differential
Geometry , band 70 (ed G.Rassias), Teubner texte zur Mathematik (Leipzig
1984), 49-87.
[34] On sphericalspace forms with meta-cyclic fundamental group which are
isospectral but not equivariant cobordant Compositio Mathematica Vol 56
(1985), 171-201.
[35] K-theory for spherical space forms (joint work with Max Karoubi),
Topology and its applications 25 (1987), 179-184.
[36] The eta invariant, Pinc bordism, and equivariant Spinc bordism for
cyclic 2-groups (Joint work with A. Bahri), Pacific Journal, V128 (1987),
1-24.
[37] Pinc bordism and equivariant Spinc bordism for cyclic 2-groups (Joint
with A. Bahri), Proceedings of AMS , Vol 99 (1987), 380-382.
[38] The moments of certain complex potentials (joint with H. Fegan), Proceedings
of the AMS, Vol 96 (1986), 525-527.
[39] The eta invariant and non-singular bilinear products on Rn. Canad.
Math. Bull. Vol 30 (2), 1987 p147-154.
[40] The eta invariant and real K-theory of lens spaces. Mathematische
Zeitschrift, vol 194 (1987), 309-320.
[41] Some representations of exceptional Lie algebras, Geometriae Dedicata
25 (1988), 407-416 (with G.Seitz)
[42] An analytic computation of the additive structure of $MU*(BZ4)$, "Geometry
and Topology", edited by G.M.Rassias, 1989 World Scientific Press, 152-158.
[43] The eta invariant and the equivariant unitary bordism of spherical
space form groups. Compositio Math 65 (1988), 33-50.
[44] Leading terms in the asymptotics of the heat equation, Contemporary
Mathematics 73 (1988), 79-85
[45] The geometrical index theorem for Clifford modules "Topics in Mathematical
Analysis", edited by T.M.Rassias, 1989 World Scientific Press, 315-327.
[46] The eta invariant and equivariant Spinc bordism for spherical space
form groups. Can. J. Math Vol XL 2 1988, 392-428.
[47] The complex bordism of groups with periodic cohomology (with A. Bahri,
M. Bendersky, and D. Davis) Trans. AMS V316 (1989), 673-687.
[48] K-theory and bordism of spherical space form groups, Simon Stevin
64 (1990), 179-186. (no 2 1990).
[49] A Higher order invariant of differential manifolds. Transactions of
the American Mathematical Society V 315 1 (1989), 373-388. (Joint with
G. Fredricks and P. Parker).
[50] The relationship between complex bordism and K-theory for groups with
periodic cohomology. Contempory Mathematics, V96 (1989), 19-31. (Joint
with A. Bahri and M. Bendersky).
[51] Spinors on spherical space forms, Proceedings of the 6th international
Colloquium on Differential Geometry (ed Luis A Cordero) Cursos e Congressos
da Universidade de Santiago De Compostela 61 (1988), 109-124.
[52] Spectral Geometry of Riemannian Manifolds, Contemporary Mathematics
V 101 (1989) (Recent Developments in Geometry, Ed. S.Y. Cheng, H. Choi,
and Robert E. Greene), 147-154.
[53] The eta invariant of even order operators, Differential Geometry (ed
F.J.Carreras, O. Medrano, A.M. Naveira), Lecture notes in Mathematics 1410,
Springer-Verlag 1989, 202-211.
[54] The Geometry of Spherical Space Form Groups, Series in Pure Mathematics
Volume 7, World Scientific (1989), 361 pages.
[55] Leading Terms in The heat Invariants, joint with T. Branson and B.
Orsted, Proceedings of the AMS, 108(2) (1990), 437-450.
[56] The Asymptotics of the Laplacian on a manifold with boundary, joint
with T. Branson, Comm. in PDE 15(2) (1990), 245-272.
[57] Leading terms in the heat invariants for the Laplacians of the DeRham,
signature, and spin complexes, joint with T. Branson and B. Orsted, Math.
Scand. 66 (1990), 307-319.
[58] The higher order differential invariant $\alpha$, Proceedings of the
6th international Colloquium on Differential Geometry (ed Luis A Cordero)
Cursos e Congressos da Universidade de Santiago De Compostela 61 (1988),
99-107. (With G. Fredricks and P. Parker)
[59] Variational properties of heat equation invariants for operators of
Dirac Type. Seminari di Geometria Giornate di Topologia e geometria delle
varieta Bologna (1992), 17-38.
[60] The spectral geometry of the Laplacian and the conformal Laplacian
for manifolds with boundary. (With Novica Blazic and Neda Bokan). Proceedings
of the Conference on Global Analysis and Global Differential Geometry ed.
B. Wegner, Lecture Notes (Springer Verlag) 1481 (1991), 5-17.
[61] Heat equation asymptotics of non minimal operators on differential
forms J. Math. Phys. V32, 2089-2091 (August, 1991), (With T. Branson and
S. Fulling).
[62] Functoriality and heat equation asymptotics. Differential geometry
and it applications, ed. J. Szenthe and L. Tamasry, V56 Colloquia Mathematica
Societatis Janos Bolyai, North Holand (1992), 285-315.
[63] Spectral Geometry, in Workshop in the geometry and topology of
gauge fields edited by A. Rigas, Mathematica Contemporanea (Brazilian
Mathematical Society) V2 (1992), 73-112.
[64] On the index of geometrical operators on Riemannian manifolds with
boundary. Advances in Mathematics V102 (1993), 129-183.
[65] Spectral geometry of the form valued Laplacian for manifolds with
boundary. (joint with Bokan and Blazic), Indian J. pure appl. Math 23 (1992),
103-120.
[66] An inhomogeneous elliptic complex (with N. Bokan and R. Zivaljevic).
Journal D'Analyse Mathematique, V61 (1993), 367-393.
[67] Residues of the eta function for an operator of Dirac Type, joint
with Tom Branson, Journal of Functional Analysis, V 108 1 (1992), 47-87.
[68] Manifolds whose odd higher order curvature operators have constant
eigenvalues at the basepoint. Journal of Geometrical Analysis, V 2 2 (1992),
151-156.
[69] Residues of the eta function for an operator of Dirac type with local
boundary conditions, Joint with T. Branson, Differential Geometry and its
applications 2 (1992), 249-267.
[70] Asymptotics of Laplacians defined by symmetric connections (joint
with N. Bokan) Results in Math V 20 (1991), 589-599.
[71] Heat equation asymptotics of the generalized Ahlfors Laplacian on
a manifold with boundary. Operator calculus and spectral theory, edited
by Demuth, Gramsch, and Schulze, V57 Birkh\"auser (1992), 1-13. (joint
with Branson, \O rsted, and Pierzchalski.)
[72] Manifolds whose curvature operator has constant eigenvalues at the
basepoint. Journal of Geometrical Analysis V 4 (1994), 155-158.
[73] Heat equation asymptotics of the form valued Laplacian for manifolds
with boundary (with Bokan and Blazic), in Proceedings of the 3rd congress
of geometry Thessaloniki 1991, edited by N. K. Stephanidis, Aristotle
University of Thessaloniki (1992), 84-95.
[74] Heat content asymptotics for operators of Laplace type with Neumann
boundary conditions. Joint with S. Desjardins. Math. Z. 215 (251-268 (1994).
[75] The functional determinant of a four dimensional boundary value problem.
Joint with Tom Branson. TAMS 344 (1994), 479-531.
[76] Heat content asymptotics of a Riemannian manifold with boundary. Joint
with M. van den Berg. J. Functional Anal V120 (1994), 48-71.
[77] Functorality and heat content asymptotics for operators of Laplace
type. Joint with van den Berg and Desjardins. Topological methods in nonlinear
anlaysis V2 (1993), 147-162. Introduction in GIF
format
[78] Heat equation asymptotics of elliptic operators with non scalar leading
symbol. Joint with Branson, and Pierzchalski. Math. Nachrichten 166 (1994)
207-215. Introduction in GIF format
[79] Heat content asymptotics of non minimal operators. Topological methods
in non-linear analysis. Vol 3 1994 69-80. Introduction
in GIF format
[80] Isoparametric geodesic spheres and a conjecture of Osserman concerning
the Jacobi operator. Joint with Swann and Vanhecke. Quart J Math. Oxford
46 (1995), 299-320. Introduction in Gif format
[81] Invariants of locally conformally flat manifolds, joint with Tom Branson
and Juha Pohjanpelto. Transactions of the AMS 347 (1995) 939-954.
Abstract
in Gif format
[82] Second edition of book Invariance Theory, the heat equation, and
the Atiyah-Singer index theorem CRC Press ISBN 0-8493-7874-4 (1994)
516pp. Bibliography by H. Schroeder (Dortmund).
Dr. Andreas Schaefer has most kindly provided a list of some misprints. One is
grateful to him for these: Corrections
[83] The eta invariant and metrics of positive scalar curvature. With B.
Botvinnik. Math. Ann. 302 (1995), 507-517. Introduction
in Gif format
[84] Heat invariants for odd dimensional hemispheres. With with M. van
den Berg. Procedings of the Royal Society of Edinburgh 126A (1996), 187-193.
Introduction
in
Gif format
[85] Applications of spectral geometry to affine and projective geometry.
Joint with N. Bokan and U. Simon. Beitraege zur Algebra und Geometrie vol
35 (1994) 2 p 283-314. Introduction in GIF format
[86] The eta invariant, equivariant spin bordism, and metrics of positive
scalar curvature. Joint with B. Botvinnik. Operator Theory: Advances
and Applications, vol 78 (Birkhauser) p 141-152. Abstract
in
GIF format
[87] When the leading terms in the heat equation asymptotics are coercive.
Joint with N. Blazic, N. Bokan, and T. Branson. Houston Math Journal V21
(1995), 75-82. Abstract in GIF format
[88] Metrics of positive scalar curvature on spherical space forms. With
B. Botvinnik. Canadian Math Journal v48 (1996), 64-80. Introduction
in GIF format
[89] Second order local affine invariants. Joint with Bokan and Simon.
Shirokov volume, Izvestija Vusov Math (Kazan) 5 (396),11-15 (1995) IN RUSSIAN.
Abstract
in GIF format
[90] The eta invariant and the equivariant spin bordism of spherical space
form 2 groups. New developments in Differential geometry, ed L.
Tamassy and J. Szenthe (1996), v350 Mathematics and its applications, Kluwer
Academic Publishers ISBN 0-7923-3822-7, p213-223. with B. Botvinnik.
introduction
in GIF format
[91] Geometry of manifolds and heat content asymptotics for operators of
Laplace type. (joint with Bokan and Blazic). Topology of submanifolds VII
(ed Dillen, Magid, Simon, Woestijne, Verstraelen). World Scientific (ISBN
981-02-2195-9 1995), p 84-87. Click for further information
[92] Eigenvalues of the Laplacian for sphere bundles. Topological methods
in non-linear analysis 5 (1995), 341-344. Click for further
information
[93] Riemannian submersions which preserve the eigenforms of the Laplacian.
With J. H. Park. Illinois J Math 40 (1996), 194-201. Click for further
information
[94] An analytic computation of $ko_{4\nu-1}(BQ_8)$. Joint with Botvinnik.
TMNA Vol 6 (1995), 127-135. Click for further information
[95] Relating the second and fourth order terms in the asymptotic expansion
of the heat equation. Joint with Bokan and Simon. Journal of Geometry,
Vol 58 pp 61-65 1997. Click for further information
[96] Eigenvalues of the Laplacian and Riemannian submersions. With J. H.
Park. Yokohama Math. Journal 43 (1995), 7-11. Click for further information
[97] The functional determinant in the standard conformal class on four
dimensional balls and spherical shells.Joint with T. Branson. Proceedings
of the 24th National Conference of Geometry and Topology, ed. A. C. Albu
and M. Craioveanu, Mirton Publishing Company Timisoara (1996), 59-80. Click
for further information
[98] The asymptotics of the Laplacian on a manifold with boundary II.Joint
with T. Branson and D. Vassilevich. Bollettino dell'Unione Matematica Italiana
(7) 11-B (1997) Suppl. fasc. 2 39-67. hep-th/9504029
[99] A note on Osserman lorentzian manifolds, Bulletin of the London Math
Society vol 29 (1997), no. 2, 227-230. Joint with N. Blazic and N. Bokan.
Click for further information
[100] The Bochner Laplacian, Riemannian submersions, heat content asymptotics
and heat equation asymptotics. Joint with J. H. Park. Czech Math J. 49,
1999, 233-240 Click for further information
[101] The Gromov-Lawson-Rosenberg Conjecture: when do manifolds admit metrics
of positive scalar curvature. Geometry from the Pacific Rim: Proceedings
of the Pacific Rim Geometry Conference held in Singapore Dec. 1994. Publisher:
Walter de Gruyter ISBN 3-11-014792-0 (1997) p149-159. Click for further
information
[102] The spectral geometry of the Hopf fibration, joint with J. V. Leahy
and J. H. Park. Journal Physics A 29 (1996), 5645-5656. Click for
further
information
[103] The local index formula for a Hermitian manifold, joint with S. Nikcevic,
and J. Pohjanpelto, Pacific Journal V180 (1997), 51-56. Click for further
information
[104] A note on the eigenfunctions of the Laplacian for a twisted holomorphic
product. Communications of Korean Math. Soc. \#12 (1997) 325-332. Joint
with J. H. Park Click for further information
[105] The Gromov Lawson Rosenberg conjecture for groups with periodic cohomology.
Joint with Botvinnik and Stolz. J. Differential Geometry Vol 46 (1997),
374-405.
[106] The Gromov-Lawson-Rosenberg conjecture: the twisted case. Joint with
B. Botvinnik. Houston Math J v23 (1997), 143-160.
[107] Geometry of differential operators on Weyl manifolds. Joint with
Bokan and Simon. Proc R. Soc. London A (1997) vol 453 pages 2527-2536
[108] Invariant metrics of positive Ricci curvature on principal bundles,
with JeongHyeong Park and Wilderich PAMS Vol 126 1845-1850 (1998)
[109] Heat equation with inhomogeneous Dirichlet conditions. Joint with
van den Berg. Comm. Analysis and Geometry 7 ( 1999) 279-294.
[110] Eigenvalues of the form valued Laplacian for Riemannian submersions.
Joint with Leahy and Park. PAMS Vol 126 1845-1850 (1998)
[111] A comparison estimate for the heat equation with an application to
the heat content of the s-adic von Koch snowflake. Joint with van den Berg.
Bull London Math Soc 30 (1998), 404-412
[112] Vacuum expectation value asymptotics for second order differential
operators on manifolds with boundary, with T. Branson and Dmitri Vassilevich.
Journal of Mathematical Physics, Vol 39 (1998) pp. 1040-1049. hep-th/9702178
Erratum-ibid. 41 (2000) 3301
[113] Eigenforms of the spin Laplacian and projectable spinors for principal
bundles. With Leahy and Park. J. Nucl. Phys. B. 514 [PM] (1998) 740-752.
[114] Generalized Osserman Manifolds. Abhandlungen aus dem Mathematischen
Seminar der Universit\"at Hamburg, 68 (1998), 125-127
[115] Pontrjagin forms, Chern Simons classes, Codazzi transformations,
and affine hypersurfaces. Joint with Blazic and Bokan. Journal of Geometry
and Physics Vol 27 (1998), 333-349.
[116] Logarithmic terms in asymptotic expansions of heat operator traces,
with Gerd Grubb. Comm on PDE Vol 23 (1998) p 777-792.
[117] Pseudo Riemannian manifolds whose generalized Jacobi operator has
constant characteristic polynomial. Joint with Stanilov and Videv. J. Geom
62 (1998) 144-153.
[118] The eta invariant and the connective $K$ theory of cyclic 2 groups.
Joint with Barrera-Yanez. Annals of Global Analysis and Geometry Vol 17
(1999), 289-299.
[119] A remark on the heat equation with inhomogeneous Neumann boundary
conditions Joint with M. van den Berg. J. Potential Analysis Vol 14, 269-274
(2001)
[120] Spectral invariants of affine hypersurfaces. Joint Bokan and Simon.
Publications de l'Institut Mathematique Publications de l'Institut Mathematique
Vol 64 (1998), 133-145.
[121] Riemannian manifolds whose skew-symmetric curvature operator has
constant eigenvalues II. Differential geometry and applications (ed Kolar,
Kowalski, Krupka, and Slovak) Publ Massaryk University Brno Czech Republic
1999 ISBN 80-210-2097-0, 73-87.
[122] The eta invariant of pin manifolds with cyclic fundamental groups.
Periodica Math. Hungarica. Vol 36 (1998) 139-170.
[123] The eigenforms of the complex Laplacian for a holomorphic Hermitian
submersion, Nagoya Math. J. 156, 1999, 1-23 joint with Leahy and Park.
[124] The heat content asymptotics for variable geometries. J. Phys. A:
Math. Gen. 32 (1999) 2825-2834.
[125] Riemannian manifolds whose skew-symmetric curvature tensor has constant
eigenvalues (with J Leahy and H Sadofsky).
[126] The heat content asymptotics of a time dependent process. joint with
van den Berg.
[127] Spectral Geometry, Riemannian Submersions, and the Gromov-Lawson
Conjecture. With JV Leahy and JH Park. ISBN 0-8493-8277-7 Publ 1999 CRC
Press 279 pp
[128] Heat asymptotics with spectral boundary conditions. Joint with Dowker
and Kirsten. Eds: B. Booss-Bavnbek, K. Wojciechowski, Geometric Aspects
of Partial Differential Equations. Proceedings of a Minisymposium on Spectral
Invariants, Heat Equation Approach, September 18-19, 1998, Roskilde, Denmark.
Contemporary Mathematics Vol 242, Amer. Math. Soc., Providence, R.I., 1999.
pp 107-124. Archive hep-th/0004020
[129] Spinors, Spectral Geometry, and RIemannian Submersions, with J Leahy
and JH Park. Published on the EMIS server at:
http://cdns.emis.de/monographs/GLP/index.html.
(Originally published as Lecture Notes Series \#40, Research Institute
of Mathematics, Global Analysis Research Center, Seoul National University
1998, 143 pages. Abstract available from Seoul National University:
http://www.garc.snu.ac.kr/print/lecture/40.html)
[130] Heat kernel asymptotics with mixed boundary conditions. Branson,
Gilkey, Kirsten, and Vassilevich. Nuclear Physics B 563 (1999), 603-626.
hep-th/9906144
[131] Spinors, self-duality, and IP algebraic curvature tensors of rank
4. Joint with Semmelman. ESI preprint 616.Proceedings of the Symposium
in Contemporary Mathematics in honor of 125 years of faculty of Math at
Univ Belgrade, N. Bokan Editor ISBN 86-7589-014-1, pp 1-12 (2000)
[132] Relating algebraic properties of the curvature tensor to geometry.
Novi Sad J Math V29 (1999), 109-119.
[133] Heat content asymptotics of time dependent processes with source
terms and with inhomogeneous boundary conditions. Joint with JH Park. Mod.
Phys. Lett. A. Vol 15 \#18 (2000) pp 1165-1179.
[134] Bundles over projective spaces and algebraic curvature tensors. Journal
of Geometry 71 (2001), 54-67.
[135] Algebraic curvature tensors which are $p$ Osserman. Differential
Geometry and its Applications, 14 (2001) 297-311.
[136] Geometric consequences of some algebraic properties of the curvature
tensor. Bull. Math. Soc. Sc. Math. Roumanie Tome 45 (93) (2000), 255-265.
Joint with R. Ivanova
[137] Algebraic curvature tensors whose skew-symmetric curvature operator
has constant rank 2. Periodica Mathematica Hungarica, Vol 44 (2002), 7-26.
Joint with T. Zhang
math.DG/0205080
[138] On properties of the asymptotic expansion of heat trace for the N/D
problem (with Dowker and Kirsten). hep-th/0010199
INT J MATH 12 (2001), 505-517
[139] Heat Trace Asymptotics of a time dependent process", joint with Klaus
Kirsten and JeongHyeong Park. J. Phys. A:Math. Gen Vol 34 (2001) 1153-1168.
hep-th/0010136
[140] Heat trace asymptotics with transmittal boundary conditions and quantum
brane-world scenario. Nuclear Physics B., 601 (2001), 125-148
hep-th/0101105
Joint with Kirsten and Vassilevich.
[141] The geometry of the skew-symmetric curvature operator in the complex
setting. Joint with R. Ivanova. Global Differential Geometry: The Mathematical
Legacy of Alfred Gray Ed. M Fernández and J. Wolf, Contemporary
Mathematics vol 288 - ISBN 0-8218-2750-2 (2001), 325-333 http://arXiv.org/PS_cache/math/pdf/0205/0205081.pdf
[142] The Jordan normal form of Osserman algebraic curvature tensors, with
R. Ivanova. Resul. Math. 40 (2001) 192-204. Paper in DVI
format.
[143] Algebraic curvature tensors for indefinite metrics whose skew-symmetric
curvature tensor has constant Jordan normal form. Joint with T. Zhang.
Houston Math J 11 (Chern Volume) 28 (2002), 311-328. math.DG/0205079
[144] Geometric Properties of Natural Operators Defined by the Riemann
Curvature Tensor. Available from World Scientific ISBN 981-02-4752-4
[145] Curvature tensors whose Jacobi or Szabo operator is nilpotent on
null vectors. With I. Stavrov. Bulletin of the London Math Soc 34 (2002),
650-658. math.DG/0205074
[146] The Jordan normal form of higher order Osserman algebraic curvature
tensors. Joint with R. Ivanova. Comment. Math. Univ. Carolinae 43 (2) (2002)
231-242 Comment. Math. Univ. Carolinae.math.DG/0205073
[147] Spacelike Jordan Osserman algebraic curvature tensors in the higher
signature setting. Joint with R. Ivanova. Proceedings Valencia Conference.
World Scientific. ISBN 981-02-4906 (2002) 179-186, math.DG/0205072
[148] Heat content asymptotics for oblique boundary conditions. Joint with
K. Kirsten and JH Park. Letters in Mathematical Physics 59 (2002) 269-276.
http://www.mis.mpg.de/preprints/2002/prepr2002\_48.html
[149] Heat asymptotics with spectral boundary conditions II. With Kirsten.
Proceedings of the Royal Society of Edinburgh A 133 (2003) 333-361 math-ph/0007015
[150] Higher order Jordan Osserman, Pseudo-Riemannian manifolds, joint
with Ivanova and Zhang, Class and Quantum Gravity, 19 (2002), 4543-4551.
math.DG/0205269
[151] Heat content asymptotics for spectral boundary conditions, joint
with Kirsten and Park, Trends in Mathematics (Kaist) 5 (2002), 49-58.
math-ph/0209012
[152] Szabo Osserman IP Pseudo-Riemannian manifolds. Joint with Ivanova
and Zhang. Publicationes Mathematicae Publ. Math. Debrecen 62 (2003), 387-401.
math.DG/0205085
[153] The eta invariant and the real connective $K$-theory of the classifying
space for quaternion groups, joint with Barrera-Yanez, Annals Global Analysis,
vol 23 (2003), 173-188 math.DG/0205084
[154] Duality symmetry of the p-form effective action and super trace
of the twisted de Rham complex, with K. Kirsten, D. Vassilevich, and A.
Zelnikov, Nuclear Physics B. Nuclear Physics B 648 (2003), 542-556. hep-th/0209125
[155] Heat trace asymptotics defined by transfer boundary conditions, Joint
with Kirsten and Vassilevich. Letters in Mathematical Physics vol 63 (2003),
29-37. hep-th/0208130
[156] Heat content asymptotics with transmittal and transmission boundary
conditions. Joint with Kirsten. J. London Math Soc. (2) 68 (2003), 431-443
math-ph/0206022
[157] Nilpotent Szabo, Osserman and Ivanov-Petrova pseudo-Riemannian manifolds,
joint with B. Fiedler. Contemporary Mathematics 337 (Recent Advances in
Riemannian and Lorentzian Geometries), 2003, pp 53-64. math.DG/0211080.
[158] Supertrace divergence terms for the Witten Laplacian, with joint
with Kirsten and Vassilevich. Potential Analysis 20 (2004) 223-235. math-ph/0212023
[159] Jordan Szabo algebraic covariant derivative curvature tensors, joint
with Raina Ivanova and Iva Stavrov. Contemporary Mathematics 337 (Recent
Advances in Riemannian and Lorentzian Geometries), 2003, pp 65-76.
math.DG/0211089.
[160] Divergence terms in the supertrace heat asymptotics for the de Rham
complex on a manifold with boundary, joint with Kirsten and Vassilevich.
Journal of Geometry and Physics, Volume 49, Issues 3-4, March 2004, Pages
249-271.
math-ph/0211020
[161] Strong ellipticity and spectral properties of chiral bag boundary
conditions. Joint with C.G. Beneventano, K. Kirsten, and E.M. Santangelo.
J. Phys. A 2 36 No 45 (14 November 2003) 11533-11543 hep-th/0306156
[162] Asymptotic Formulae Spectral Geometry, to be published by CRC Press
26 Dec 2003
[163] Heat content, torsional rigidity and generalized Hardy inequalities
for complete Riemannian manifolds, with M. van den Berg. Bulletin of the
London Mathematical Society 36 (2004), 577-586math.DG/0302255
[164] Manifolds which are Ivanov-Petrova or k-Stanilov. Joint with S. Nikcevic
and V. Videv. Journal of Geometry. Volume 80 (2004), 82-94. math.DG/0310118
[165] Curvature homogeneous spacelike Jordan Osserman pseudo-Riemannian
manifolds, with S. Nikcevic. Class. Quantum Grav 21 (2004), 497-507.
math.DG/0310024
[166] Nilpotent Spacelike Jorden Osserman pseudo-Riemannian manifolds,
joint with S. Nikcevic. ``Proceedings of the Winter School `Geometry and
Physics', Srni (2003)''. Rendiconti del circolo mathematico di Palermo
Serie II Suppl. 72 (2004) 99-105. math.DG/0302044
[167] Conformally Osserman manifolds and conformally complex space forms,
joint with N. Blazic, International Journal of Geometric Methods in Modern
Physics (2004), 97-106; math.DG/0311263
[168] Curvature homogeneous pseudo-Riemannian manifolds which are not locally
homogeneous. Joint with C. Dunn. Complex, contact and symmetric
manifolds, 145-152, Progr. Math., 234, Birkhauser Boston, Boston,
MA, 2005. math.DG/0306072.
[169] Heat content asymptotics for operators of Laplace type with spectral
boundary conditions with K. Kirsten, and JH. Park. Letters in Mathematical
Physics 68 (2) (2004) 67-76 math-ph/0308021
[170] The structure of algebraic covariant derivative curvature tensors,
joint with J. Diaz-Ramos, B. Fiedler, and E. Garcia-Rio; Int. J. Geom.
Methods Mod. Phys. 1 (2004), no. 6, 711-720 math.DG/0404060
[171] Complete curvature homogeneous pseudo-Riemannian manifolds, joint
with S. Nikcevic. Classical and Quantum Gravity, volume 21, issue 15, pages
3755 - 3770.
math.DG/0402282
[172] Eigenforms of the Laplacian for Riemannian V-submersions. Tohoku
Math J 57 (2005), 505-519. Joint with J. H. Park. math.DG/0310439
[173] Curvature homogeneous signature $(2,2)$ manifolds. "Differential
Geometry and its Aplications, Proc. Conf. Prague, August 30-September 3,
2004 Charles University, Prague (Czech Republic), editors Bures, Kowalski,
Krupka, Slovak, MATFYZPRESS (2005), ISBN 80-86732-63-0, 29-44. Web
download See also math.DG/0408316
[174] Complete $k$-curvature homogeneous pseudo-Riemannian manifolds, with
S. Nikcevic. Annals of Global Analysis and Geometry. Vol 27 (2005), 87-100.
math.DG/0405024. There
was a difficulty viewing this paper electronically on Springer - it has
been fixed. Here is a PDF file
.
[175] Conformally Osserman manifolds and self-duality in Riemannian geometry.
Joint with N. Blazic. "Differential Geometry and its Aplications, Proc.
Conf. Prague, August 30-September 3, 2004 Charles University, Prague (Czech
Republic), editors Bures, Kowalski, Krupka, Slovak, MATFYZPRESS (2005),
ISBN 80-86732-63-0, 15-18. Web
download see also math.DG/0504498
[176] Complete $k$-curvature homogeneous pseudo-Riemannian manifolds $0$-modeled
on an indecomposible symmetric space, joint with S. Nikcevic. Topics in
Almost Hermitian Geometry and the Related Fields, World Scientific (2005),
87-104. math.DG/0504050
[177] Heat kernel coefficients for chiral bag boundary conditions, joint
with K. Kirsten and G. Esposito. J. Phys. A: Math. Gen. 38 No 10 (11 March
2005) 2259-2276.
math.AP/0510156
[178] Generalized plane wave manifolds, Kragujevac J. Math. 28 (2005),
113-138, joint with S. Nikcevic. math.DG/0505253
[179] The spectral geometry of the Weyl conformal tensor. Joint with N.
Blaic, S. Nik\cevic, and U. Simon. Banach Center Publ. 69 (2005), 195-203
math.DG/0310226
[180] Affine curvature homogeneous 3-dimensional Lorentz Manifolds. Joint
with S. Nikcevic. International Journal of Geometric Methods in Modern
Physics Vol. 2, No. 5 (2005) 737-749; math.DG/0504163
[181] Eta invariant with spectral boundary conditions, joint with K. Kirsten
and J.H. Park. J. Phys. A: Math. Gen. 38 8103-8122 (2005); math-ph/0406028
[182] Isometry groups of $k$-curvature homogeneous pseudo-Riemannian manifolds,
joint S. Nikcevic, Rendiconti del Circolo Matematico di Palermo,
Serie II, Suppl. 79 (2006), 99-110.
math.DG/0505598
[183] Heat Content Asymptotics for Riemannian manifolds with Zaremba boundary
conditions, with M. van den Berg, K. Kirsten, and V. A. Kozlov, Potential
Analysis 26 (2007), 225-254; see also math-ph/0506076
[184] Stability theorems for chiral bag boundary conditions. With K. Kirsten.
math.AP/0510152
Letters in mathematical physics V73 147 - 163 (2005).
[185] Jacobi-Tsankov manifolds which are not $2$-step nilpotent, Proceedings
of the conference Contemporary Geometry and Related Topics (2007),
63-80. ISBN 86-7589-059-1, joint with M. Brozos-Vazquez and S. Nikcevic.
math.DG/0609565
[186] Pseudo-Riemannian manifolds with Commuting Jacobi Operators, with
M. Brozos-Vazquez.
Rendiconti Del Circolo Matematico di Polermo
Vol 55 (2006), 163-174.
math.DG/0608707
[187] Manifolds with commuting Jacobi operators, joint with M. Brozos-Vazquez.
Journal Geometry Volume 86 (2006), 21-30;
math.DG/0507554
[188] The global geometry of Riemannian manifolds with commuting curvature
operators, joint with M. Brozos-Vazquez. Journal Fixed Point Theory (2007),
87-96; Max Planck Institute Preprint
2006-88.
[189] Relating the curvature tensor and the complex Jacobi operator of an
almost Hermitian manifold. Joint with Brozos-Vazquez and Garcia-Rio.
Advances in Geometry. Volume 8, Issue 3, Pages 353-365. See alsoMPI preprint #135-2006
190] Algebraic theory of affine curvature tensors, joint with N. Blazic,
S. Nikcevic, and U. Simon. Archivum Mathematicum, Masaryk University (Brno,
Czech Republic) ISSN 0044-8753, tomus 42 (2006), supplement:
Proceedings of the 26th Winter SchoolGeometry and Physics 2006 (SRNI),
147-168.
math.DG/0609759
[191] Complex Osserman Algebraic Curvature Tensors and Clifford Families,
joint with M. Brozos-Vazquez. Houston
Journal of Mathematics, Vol 34 (2008), 677-702.
math.DG/0608749.
[192] Completeness, Ricci blowup, the Osserman and the conformal Osserman
condition for Walker signature (2,2) manifolds. "Proceedings
of the XV International Workshop on Geometry and Physics" (Puerto de la Cruz, Tenerife, Canary Islands, Spain
September 11-16 2006). Publ. de la RSME 10 (2007), 57-66.
Joint with M. Brozos-Vazquez,
E. Garcia-Rio, and R. Vazquez-Lorenzo. math.DG/0611279 and MPI preprint.
[193] Projectively Osserman manifolds. Joint with Brozos-Vazquez, S. Nikcevic,
and U. Simon. Publicationes
Mathematicae Debrecen72 (2008), 359-370;
http://arxiv.org/abs/0708.1210.
[194] The spectral geometry of the canonical Riemannian submersion of a
compact Lie Group, J.
Geometry and Physics 57 (2007), 2065-2076. Joint with Dunn and Park.
MPI preprint
[195] The Geometry of Curvature Homogeneous Pseudo-Riemannian Manifolds.
Joint with Brozos-Vazquez, Imperial
College Press ISBN 978-1-86094-785-8
see also (978-1-86094-785-8)
[196] Pseudo-Riemannian Jacobi-Videv Manifolds. Joint with Stana Nikcevic,
IJGMMP 4 (2007), 727-738.
http://arxiv.org/abs/arXiv:0708.1096
[197] Expected volume of intersection of Wiener sausages and heat kernel
norms on compact Riemannian manifolds with boundary. Joint with van den
Berg. Potential Analysis 27 (2007), 301-312. http://arxiv.org/abs/math/0609603
[198] Examples of signature $(2,2)$ manifolds with commuting curvature
operators, J. Phys. A: Math. Theor. 40 (2007) 13149-13159. Joint with M.
Brozos-Vazquez, E. Garc{\i}a-R{\i}o, and R. Vazquez-Lorenzo.
MPI
Preprint 73/2007: http://www.mis.mpg.de/preprints/2007/preprint2007\_73.pdf
[199] The classification of simple Jacobi-Ricci commuting algebraic curvature
tensors, joint with S. Nikcevic,
Note di Matematica, supplemento n.1 to vol. 28, 2008 (University of Lecce)
341-348. arXiv:0710.2080
[200] Spectral geometry, homogeneous spaces, and differential forms with finite Fourier series, joint with
C. Dunn and J-H. Park.
MPI preprint:
http://www.mis.mpg.de/preprints/2007/prepr2007\_72.html. J. Phys. A: Math. Theor. 41 No 13 (4 April
2008) 135204.
[201] Heat Content, Heat Trace, and Isospectrality, Accepted by the
Proceedings of the sixth workshop on Lie Theory and Geometry (ed. C. Gordon, J.
Tirao, J. Vargas, and J. Wolf).
Contemporary Math 491 (2009), 115-124.
http://arxiv.org/abs/0802.2948.
[202] Heat Content Asymptotics with singular initial temperature distributions. Joint with
M. van den Berg and R. Seeley. J. Funct. Anal. 254 (2008), 3093-3122.
http://arxiv.org/abs/arXiv:0803.0675
[203] Geometrical representations of equiaffine curvature operators, joint
with S. Nikcevic, Results in Mathematics 52 (2008), 281-287
math.DG/0802.0265
[204] Curvature structure of Self-dual 4-manifolds, joint with N. Blazic, S. Nikcevic, I.
Stavrov. IJGMMP vol
5 issue 7, 1191-1204.
http://arxiv.org/abs/0808.2799.
[205] Geometric realizations of generalized algebraic curvature
operators. Joint with S. Nikcevic and D. Westerman. J. Math. Phys. 50,
013515 (2009). http://arxiv.org/abs/0811.3180
[206] Riemannian geometric realizations for Ricci tensors
of generalized algebraic curvature operators. Joint with S. Nikcevic and D.
Westerman. Conference Proceedings of the VIII International
Colloquium on Differential Geometry (World Sci. Publ. Co.) (2009)
175-184 ISBN 10 981-4261-16-5
http://arxiv.org/abs/0811.3841
[207] Videv models and manifolds, joint with V. Videv.
J. Geom. 92 (2009), 60-68.
arxiv.org/abs/math.DG/0605464.
[208] The geometry of modified Riemannian extensions.
E. Calvino-Louzao, E. Garcia-Rio, P. Gilkey, and R. Vazquez-Lorenzo.
Proc. R. Soc. A. (2009) 465, 2023-2040. doi:10.1098/rspa.2009.0046
arxiv.org/abs/arXiv:0901.1633
[209] Geometric realizations of curvature models by manifolds
with constant scalar curvature. By
M. Brozos-Vazquez, P. Gilkey, H. Kang, S. Nikcevic, G. Weingart.
Differential Geometry and its Applications
27 (2009), 696-701.
doi:10.1016/j.difgeo.2009.05.002
http://arxiv.org/abs/arXiv:0811.1651.
[210] Heat trace asymptotics with singular weight functions, joint with
M. van den Berg, P. Gilkey, and R. Seeley,
http://arxiv.org/abs/0806.1706.
Communications in Analysis and Geometry, Vol 17 (2009), 529-563.
[211] Geometric Realizations of para-Hermitian curvature models,
M. Brozos-Vazquez, P. Gilkey, S.
Nikcevic, and R. V{a}zquez-Lorenzo,
Results in Mathematics 56
(2009), 319-333.
http://arxiv.org/abs/arXiv:0902.1697.
[212]
The Geometry of Walker Manifolds
Miguel Brozos-Vazquez, Eduardo Garcia-Rio, Peter Gilkey, Stana Nikcevic, Ramon
Vazquez-Lorenzo Synthesis Lectures on Mathematics and Statistics, Vol. 2, No.
1, Morgan and Claypool, Pages 1-179 (2009). ISBN 9781598298192
Now a Springer Link Book https://link.springer.com/book/10.1007/978-3-031-02397-2
[213]
Geometric Realizations of Hermitian curvature models joint with M.
Brozos-Vazquez, H. Kang, and S. Nikcevic.
J. Math Soc. Japan, Vol 62 (2010), 851-866.
arXiv:0812.2743.
[214] Geometric theory of equiaffine curvature tensors,
joint with S. Nikcevic, and U. Simon. Results in Math., p275-317 vol 56 (2009). arxiv.org/abs/0903.5269
[215] Four-dimensional Osserman metrics of neutral signature. Joint with E.
Garc\'{\i}a-R\'{\i}o, and M. E. Vazquez-Abal, R. Vazquez-Lorenzo.
http://arxiv.org/abs/0804.0436v1
Pacific Journal of Math 244 (2010), 21-36.
[216] The eta invariant and
equivariant bordism of flat manifolds with cyclic holonomy group of odd
prime order, joint with R.J. Miatello, R.A. Podesta.
http://arxiv.org/abs/0910.0635
AGAG, Volume 37, Number 3 (2010), pages 275-306.
DOI
10.1007/s10455-009-9185-5
[217]
Curvature properties of Weyl geometries, joint with S. Nikcevic and U. Simon,
Results in Math. 59 (2011) 523-544.
arXiv:1008.4278.
[218] Geometric realizations of Kaehler and of para-Kaehler curvature
models, joint with M. Brozos-V\'azquez and E. Merino,
Int. J. Geom. Methods Mod. Phys 7 (2010), 505-515. (DOI:
10.1142/S0219887810004403)
arXiv:0909.3473.
[219]
Geometric realizations, curvature decompositions, and Weyl manifolds,
Joint with Stana Nikcevic, and Udo Simon, J. Geometry Physics. 61 (2011),
270-275
arXiv: 1002.5027v1
[220]
Geometric realizations of affine Kaehler curvature models,
joint with M. Brozos-Vazquez and S. Nikcevic, Results. Math. 59 (2011),
507-521, arXiv:1007.2489.
[221] Heat trace asymptotics with singular weight functions II, joint with
M. van den Berg and K. Kirsten. J. Geo. Anal J Geom Anal (2011) 21, 870-901
DOI 10.1007/s12220-010-9170-z. Posted as
arXiv:1006.5644.
[222] Geometric Realizability of Covariant Derivative Kaehler Tensors for
almost Pseudo-Hermitian and almost Para-Hermitian Manifolds. Joint with
Miguel Brozos-V\'azquez, Eduardo Garc\'ia-R\'io, Luis Hervella. Accepted by Annali di Matematica.
Arxiv:
http://arxiv.org/abs/1012.4964.
[223]
Compact Osserman manifolds with neutral metric, joint with
M. Brozos-Vazquez, E. Garcia-Rio, and R. Vazquez-Lorenzo. Results
in Mathematics 59 (2011), 495-506.
arXiv: 1004.1145.
[224]
Higher-dimensional Osserman metrics with non-nilpotent Jacobi operators, joint
with E. Calvino-Louzao, E. Garcia-Rio, and R. Vazquez-Lorenzo.
Geom. Dedicata 156 (2012) 151--163
arXiv:1007.2569.
[225]
Complex Osserman Kaehler Manifolds. Joint with M. Brozos-Vazquez, appeared
Forum Mathematicum
http://dx.doi.org/10.1515/FORM.2011.119
Arxiv: arXiv: 1003.5379.
[226]
Acercamiento a la finitud por lo infinito: Lenguaje, TeorÂa de Modelos,
Recursion y Complejidad. En (Eds) Caparros, N., Cruz Roche, R.:
Viaje a la complejidad. Vol 4. Biblioteca Nueva, Madrid, 352-369 (2013). ISBN: 978-84-9940-999-3.
Joint with A. Karousou and S. Lopez Ornat
[227] Hardy inequality and heat semigroup estimates for Riemannian manifolds
with singular data. Joint with M. van den Berg, K. Kirsten, and A. Grigor'yan.
Communications in PDE, {\bf 37} (2012), 885-900.
10.1080/03605302.2011.596879.
arXiv: 1011.1726.
[228]
Growth of heat trace and heat content asymptotic coefficients, with
M. van den Berg and K. Kirsten. J. Funct. Anal. 261 (2011), 2293-2322.
http://arxiv.org/abs/1105.1762
[229] The structure of the space of affine K\"ahler curvature tensors as a
complex module, with Brozos-Vazquez and Nikcevic.
IJGMMP 8 (2011), 1849-1868
See also
http://arxiv.org/abs/arXiv:1105.0185.
[230] Universal curvature identities, with J. H. Park and K. Sekigawa.
http://arxiv.org/abs/1104.1883. J. Diff. Geo. Appl. Volume 29, Issue 6, December 2011, Pages 770-777.
[231]
Kaehler and para-Kaehler curvature Weyl manifolds, joint with S. Nikcevic.
http://arxiv.org/abs/1011.4844
Publ. Math. Debrecen 80 (2012), 369--384.
DOI: 10.5486/PMD.2012.5052.
[232] Universal curvature identities II,
with J.H. Park and K. Sekigawa
http://arxiv.org/abs/1111.1380,
J. Geom and Physics 62 (2012), 814--825.
[233] Geometric realizations of curvature, joint with M. Brozos-Vazquez
and S. Nikcevic, Imperial College Press (2012).
Known misprints
[234] Heat trace asymptotics and the Gauss-Bonnet Theorem for general
connections, with C. G. Beneventano, K. Kirsten, and E. M. Santangelo.
J. Phys. A: Math. Theor. 45 (2012) 374010.
http://arxiv.org/abs/1201.6301
see also On line publication
J. Phys. A: Math. Theor. 45 (2012) 374010.
[235] (para)-K\"ahler Weyl structures - with S. Nik\v cevi\'c.
`Recent trends in Lorentzian Geometry", ed. M. Sanchez, M. Ortega, A. Romero.
ISBN 978-1-4614-4896-9 Springer Verlag (2013). P335--353.
http://arxiv.org/abs/1204.0724
Springer LInk
[236] Heat content asymptotics with singular data, joint with M. van den Berg,
http://arxiv.org/abs/1201.4350
Published online.
J. Phys. A: Math. Theor. 45 (2012) 374027.
[237] Growth of heat trace coefficients for locally
symmetric spaces, with R. J. Miatello.
http://arxiv.org/abs/1109.6228 .
J. Math. Phys. 53, 103506 (2012); doi: 10.1063/1.4751279
[238] Kaehler-Weyl manifolds of dimension 4, with S. Nikcevic,
to appear Rendiconti del Seminario Matematico.
http://arxiv.org/abs/1109.4532 .
[239] Universal curvature identities III, joint with J.H. Park
and K. Sekigawa. IJGMMP (v10 # 6 July 2013) DOI 10.1142/S0219887813500254.
http://arxiv.org/abs/1209.5481
http://dx.doi.org/10.1142/S0219887813500254
[240] Transplanting geometric structures,
Joint with Y. Euh, J.H. Park, and K. Sekigawa.
Differential Geometry and its Applications 31 (2013) 374--387.
http://arxiv.org/abs/1301.3394
[241] Homogeneity of Lorentzian manifolds with recurrent curvature.
Joint with E. Garcia-Rio and S. Nik\v cevi\'c.
Math. Nachr. 287, No. 1, 32--47 (2014)
DOI 10.1002/mana.201200302 See also
http://arxiv.org/abs/1210.7764
[242] Homogeneous 4-dimensional K\"ahler--Weyl Structure.
Joint with M. Brozos-V\'{a}zquez, E. Garc\'{\i}a-R\'{\i}o, and R. V\'{a}zquez-Lorenzo.
http://arxiv.org/abs/1211.4453
DOI: 10.1007_s00025-013-0319-5
Results in Mathematics: Volume 64, Issue 3 (2013), Page 357--369
[243] Applications of Affine and Weyl Geometry, joint with
Eduardo Garcia-Rio, Stana Nikcevic, and Ramon Vazquez-Lorenzo. In production with Morgan & Claypool.
( Preface + Table Contents.
DOI 10.2200/S00502ED1V01Y201305MAS013. TO order
www.morganclaypool.com
Now a Springer book https://link.springer.com/book/10.1007/978-3-031-02405-4
[244] Affine projective Osserman structures, joint with S. Nikcevic
http://arxiv.org/abs/1304.7482
Class. Quantum Grav. 30 (2013) 155015
http://stacks.iop.org/0264-9381/30/155015
[245] 4-dimensional (para)-K\"ahler--Weyl structures. Joint with
S. Nik\v cevi\'c. Publications de l'Institut
Mathematique (related to XVII Geometrical Seminar)
tome 94 (108) (2013), 91--98. DOI: 10.2298/PIM1308091G
http://publications.mi.sanu.ac.rs and
http://arxiv.org/abs/1210.6769
[246] Neumann heat content asymptotics with singular initial temperature and
singular specific heat, joint with M. van den Berg and H. Kang.
Festschrift Volume for Mme Choquet-Bruhat of the Journal of Fixed Point Theory and Applications. Volume 14, Issue 1 (2014), Page 267--298.
See also
http://link.springer.com/article/10.1007/s11784-013-0147-y
The normative version is
http://arxiv.org/abs/1212.1400
[247] Heat flow out of a compact manifold, joint with M. van den Berg.
J. Geom. Anal. DOI 10.1007/s12220-014-9485-2
http://arxiv.org/abs/1306.6257
[248] Heat content with singular initial temperature and singular specific
heat. Joint with M. van den Berg.
Potential Anal (2015) 42:1--38 DOI 10.1007/s11118-014-9422-9.
http://arxiv.org/abs/1401.6373
[249] Universal curvature identities and Euler Lagrange Formulas
for Kaehler manifolds.
Joint with J. H. Park and K. Sekigawa. J. Math. Soc. Japan
Volume 68, Number 2 (2016), 459--487.
http://arxiv.org/abs/1311.2622
[250] Projective affine Ossermann curvature models. Joint with B. Lim. To appear J. Fixed Point Theory Appl.
http://arxiv.org/abs/1403.1900
Journal of Fixed Point Theory and Applications: Volume 16, Issue 1 (2015), Page 243-258
[251] Analytic continuation, the Chern-Gauss-Bonnet theorem,
and the Euler-Lagrange equations in Lovelock theory
for indefinite signature metrics. Joint with J.H.Park. To appear J. Geom. Physics
Preprint
[252] Non-closed curves in $\mathbb{R}^n$ with finite total
first curvature arising from the solutions of an ODE. Joint with
P. Gilkey, C. Y. Kim, H. Matsuda, J. H. Park, and S. Yorozu,
http://http://pages.uoregon.edu/gilkey/S03-FinitenessTotalCurv-v8e.pdf
To appear Hokkaido Mathematical Journal, Vol.46, No.1, February 2017.
[253] Homothety Curvature Homogeneity and Homothety Homogeneity, joint with
E. Garcia-Rio and S. Nikcevic,
Ann. Glob. Anal. Geom. (2015) Vol 48, 149--170.
DOI 10.1007/s10455-015-9462-4
http://arxiv.org/abs/1403.6620
[254] Real analytic complete non-compact surfaces in Euclidean space with
finite total curvature arising as solutions to ODEs.
Joint with C.Y. Kim, and J. H. Park. To appear Tohoku Math J.
http://arxiv.org/abs/1411.0487
[255]
`
Aspects of Differential Geometry I, with J. H. Park and R. Vazquez-Lorenzo,
Lecture #15 (Synthesis lectures on mathematics and statistics),
Morgan and Claypool (ISBN ��������������������������9781627056625 paperback; 9781627056632 ebook).
(2015).
See Errata
PDF files or hard copy books downloaded or printed prior to 3 September may
well have a processing error in them that affected many pages. Check on page 9. If it looks like
 your copy should be discarded. On the other hand, If it looks like
your copy should be discarded. On the other hand, If it looks like
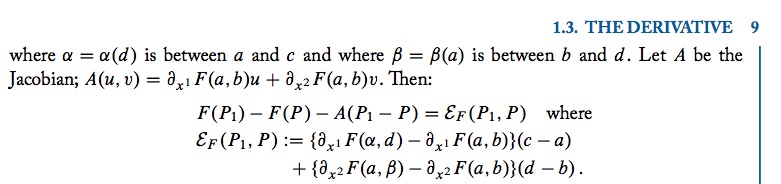 then it is fine.
then it is fine.
Now a Springer book https://link.springer.com/book/10.1007/978-3-031-02407-8
[256]
Aspects of Differential Geometry II, with J. H. Park and R. Vazquez-Lorenzo,
Lecture \#16 (Synthesis lectures on mathematics and statistics),
Morgan and Claypool (ISBN �������������9781627057837 paperback; 9781620757844 ebook).
See Errata
Now a Springer book https://link.springer.com/book/10.1007/978-3-031-02408-5
PDF files or hard copy books downloaded or printed prior to 3 September may
well have a processing error in them that affected many pages. Check on page 9. If it looks like
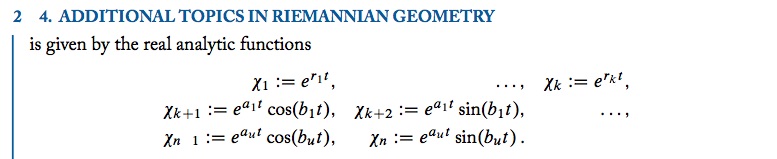 pages 2, 37, 66, and 82 are in error (the correct pages can be downloaded from
here). On the other hand, If it looks like
pages 2, 37, 66, and 82 are in error (the correct pages can be downloaded from
here). On the other hand, If it looks like
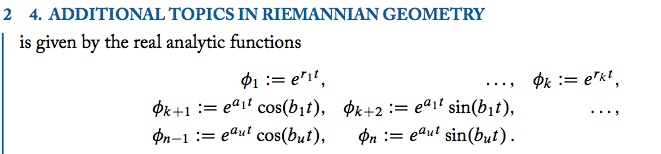 then it is fine.
then it is fine.
[257] Applications of model theory to the study of "soft" universals in language, joint
with A. Karousou and S. Lopez-Ornat. Math. Meth. Appl. Sci. 2017, 40 6096--6102 (early view
DOI: 10.1002/mma.3778)
[258] Anti-invariant Riemannian Submersions, joint with M. Itoh, J. H. Park.
To appear Tawanese J. Math. DOI: 10.11650/tjm.xx.20xx.6898
http://arxiv.org/abs/1509.04842
[259] Homogeneous affine surfaces: Moduli spaces,
joint with M. Brozos-Vazquez and E. Garcia-Rio.
J. Math. Anal. Appl. 444 (2016) 1155--1184.
http://arxiv.org/abs/1604.06610
[260] The structure of the Ricci tensor on locally homogeneous Lorentzian
gradient Ricci solitons, joint with M. Brozos-Vazquez, E. Garcia-Rio, and S. Gavino-Fernandez
Proceedings A Royal Society of Edinburgh 148A, 461--482 (2018).
http://arxiv.org/abs/1403.4400
DOI:10.1017/S0308210517000464
[261] The moduli space of Type~A surfaces with torsion and non-singular
symmetric Ricci tensor. http://arxiv.org/abs/1605.06698
J. Geometry and Physics, vol 110 (2016), 69--77.
[262] Homogeneous affine surfaces: affine Killing vector fields and Gradient Ricci solitons,
joint with M. Brozos-Vazquez and E. Garcia-Rio.
http://arxiv.org/abs/1512.05515 To appear
J. Math. Soc. Japan 70 (2018), 25--70.
[263] Moduli spaces of Type B surfaces with torsion.
http://arxiv.org/abs/1608.06069
To appear J. Geometry
DOI 10.1007/s00022-016-0364-9.
[264] Geodesic completeness for Type~$\mathcal{A}$ surfaces
joint with D. D'Ascanio, and P. Pisani.
https://arxiv.org/abs/1611.00941
J. Diff. Geo. Appl. 54 (2017), 31--43.
(http://dx.doi.org/10.1016/j.difgeo.2016.12.008).
[265] Moduli spaces of oriented Type A manifolds of dimension at least 3
joint with J.H. Park. J. Korean Math. Soc. 54 (2017), No. 6, pp. 1759Ã1786
https://doi.org/10.4134/JKMS.j160647
http://arxiv.org/abs/1607.01563.
[266] Aspects of Differential Geometry III, with E. Calvino-Louzao, E. Garcia-Rio,
J. H. Park and R. Vazquez-Lorenzo,
Lecture \#18 (Synthesis lectures on mathematics and statistics),
Morgan and Claypool (ISBN �������������9781627056861 paperback; 9781627058827 ebook).
Now a Springer book https://link.springer.com/book/10.1007/978-3-031-02410-8
[267] The affine quasi-Einstein Equation for homogeneous surfaces
with Miguel Brozos-V\'azquez, Eduardo Garc\'ia-R\'io, and
Xabier Valle-Regueiro
http://arxiv.org/abs/1707.06304
On line Manuscripta Mathematica
doi="10.1007/s00229-017-0987-7",
https://doi.org/10.1007/s00229-017-0987-7
[268] Half conformally flat generalized quasi-Einstein manifolds, joint with
Miguel Brozos-V\'azquez, Eduardo Garc\'ia-RÂo,and Xabier Valle-Regueiro, https://arxiv.org/abs/1702.06714.
International Journal of Mathematics Vol. 29, No. 1 (2018) 1850002 (25 pages) DOI: 10.1142/S0129167X18500027. The version on the arXiv should be regarded as normative and
should be consulted in preference to the journal version.
[269]The geometry of locally symmetric affine surfaces, joint with D. D'Ascanio and P. Pisani,
http://arxiv.org/abs/1706.04958. Appeared
Vietnam J. Math (Zeidler memorial volume) Volume 47 (2019), 5--21. https://doi.org/10.1007/s10013-018-0280-4.
The version on the arXiv should be regarded as normative rather than the journal version.
[270] A natural linear equation in affine geometry: the affine quasi-Einstein
equation, joint with
Miguel Brozos V'azquez, Eduardo Garc'ia R'io, and
Xabier Valle Regueiro
http://arxiv.org/abs/1705.08352.
dx.doi.org/10.1090/proc/14090
Proc. AMS Vol 146 (8), 3485--3497.
[271] The Geometry of Spherical Space Form Groups 2nd Edition, ISBN: 978-981-3220-78-2 (hard cover),
978-981-3220-80-5 (e-book), World Scientific Press (Singapore) April 2018 (Series in Pure Mathematics: Volume 28).
http://www.worldscientific.com/worldscibooks/10.1142/10467
[272] Affine surfaces which are Kaehler, Para-Kaehler, or nilpotent Kaehler,
joint with E. Calvino-Louzao, E. Garcia-Rio, I. Gutierrez-Rodriguez, and R. Vazquez-Lorenzo.
https://arxiv.org/abs/1801.08306.
Appeared Results in mathematics
Results Math (2018) 73:135
[273] Applications of PDEs to the study of affine surface geometry,
joint with X. Valle-Regueiro
http://arxiv.org/abs/1806.06789
Matematicki Vesnik
Vol 71, 1Ã2 (2019), 45--62 March 2019.
[274] Constructing Bach Flat Manifolds of signature $(2,2)$ using the modified Riemannian extension,
joint with Calvi\~no-Louzao], E. Calvi\~no-Louzao, E. Garc\'{i}a-R\'{i}o,
I. Guti\'errez-Rodr\'iguez, and
R. V\'{a}zquez-Lorenzo. http://arxiv.org/abs/1807.09218
J. Math. Phys. 60, 013511 (2019); https://doi.org/10.1063/1.5080319
[275] Affine Killing complete and geodesically complete homogeneous affine
surfaces, joint with J.H.Park and X. Valle-Regueiro,
http://arxiv.org/abs/1811.05197.
Accepted Journal of Mathematical Analysis and Applications https://doi.org/10.1016/j.jmaa.2019.01.038.
[276] Aspects of Differential Geometry IV (2019), with
E. Calvi\~no-Louzao, E. Garc\'\i{}a-R\'\i{}o,
J. H. Park, and R. V\'{a}zquez-Lorenzo,
Synthesis lectures on mathematics and statistics volume 11 (167 pages).
Morgan and Claypool ISBN: 9781681735634 (paperback), ISBN: 9781681735641(ebook) ISBN: 9781681735658 (hard cover).
Now a Springer book
https://link.springer.com/book/10.1007/978-3-031-02416-0
[277] Affine Killing vector fields on homogeneous surfaces with torsion,
joint with Daniela D'Ascanio and Pablo Pisani, Class. Quantum Gravity
https://arxiv.org/abs/1906.01694
[278] Solutions to the affine quasi-Einstein equation for homogeneous surfaces,
joint with M. Brozos-Vazquez, E. Garcia-Rio, and X. Valle-Regueiro, Adv. Geom. 2020; 20 (3):413--432.
https://arxiv.org/abs/1802.07953.
[279] Geodesic completeness and the quasi-Einstein
equation for locally homogeneous affine surfaces, joint with X. Valle-Regueiro.
http://arxiv.org/abs/1908.04148
Recent advances in Pure and Applied Mathematics Volume 4, Springer 2020, 175--184.
[280] Heat flow from polygons,
joint with Michiel van den Berg and Katie Gittins.
http://arxiv.org/abs/1902.02606. Potential Analysis (2020) 53:1054-1062..
http://link.springer.com/article/10.1007/s11118-019-09797-5
[281] Spaces of locally homogeneous affine surfaces,
joint with M. Brozos-V\'{a}zquez \, E. Garc\'{i}a-R\'{i}o.
https://arxiv.org/abs/1903.11841
Revista de la Real Academia de Ciencias Exactas, Fsicas y Naturales. Serie A. Matematicas
https://doi.org/10.1007/s13398-019-00763-9
RACSAM 114:39 (2020).
[282]``Symmetric affine surfaces with torsion", joint with D. D'Anscio and P. Pisani
J. Geometry and Physics
doi.org/10.1016/j.geomphys.2019.103536.
See also http://arxiv.org/abs/1910.10028
[283] On distinguished local coordinates for locally homogeneous affine surfaces
joint with M. Brozos-Vazquez and E. Garcia-Rio,
http://arxiv.org/abs/1901.03523.
Monatshefte fur Mathematik
https://doi.org/10.1007/s00605-020-01382-y Vol 192 (65--74) (2020).
[284] An almost Zoll affine surface, Proceedings of Abel Symposium 16 (2019),
Springer Verlag https://link.springer.com/book/10.1007/978-3-030-81296-6
2022.
https://arxiv.org/abs/2006.09998
[285] The local index density of the perturbed de~Rham complex, joint with J. Alvarez-Lopez
http://arxiv.org/abs/2004.02243. To appear Czech Math J
[286] Harmonic spaces and density functions, joint with J. H. Park.
Results Math (2020) 75:121 doi.org/10.1007/s00025-020-01248-7
,http://arxiv.org/abs/2001.06715.
[287] Derived Heat Trace Asymptotics for the de Rham and Dolbeault complexes, joint with J. Alvarez Lopez.
http://arxiv.org/abs/2010.01146 To appear
J. Pure and Applied Functional Analysis vol 8 (2023), 49--66.
[288]
Aspects of Differential Geometry V, with
E. Calvi\~no-Louzao, E. Garc\'\i{}a-R\'\i{}o,
J. H. Park, and R. V\'{a}zquez-Lorenzo.
Now a Springer book https://link.springer.com/book/10.1007/978-3-031-02432-0
ISBN: 9781636391106. Morgan \& Claypool (2021).
[289] Harmonic radial vector fields on harmonic spaces, joint with JH Park
http://arxiv.org/abs/2009.02879
To appear J. Math. Analysis and Applications.
[290] The Witten deformation of the Dolbeault complex, Joint with J. Alvarez Lopez
http://arxiv.org/abs/2007.15889.
J. Geometry 112:25 (2021) https://doi.org/10.1007/s00022-021-00589-0
[291] Centrally Harmonic spaces, Joint with J. H. Park,
Periodica Mathematica Hungarica (publ. online 9 March 2022)
https://doi.org/10.1007/s10998-022-00456-8
[292] Maximal domains of radial harmonic functions, joint with J. H. Park
https://arxiv.org/abs/2205.13829
Mediterranean Journal of Mathematics.
https://doi.org/10.1007/s00009-023-02309-1 (2023) 20:126
[293] Volume density asymptotics of central harmonic spaces, joint with J. H. Park
http://arxiv.org/abs/2206.04892
Bull. Korean Math. Soc. 60 (2023) 1539--1553.
Web page updated 25 November 2023 by
Peter
B Gilkey
 your copy should be discarded. On the other hand, If it looks like
your copy should be discarded. On the other hand, If it looks like
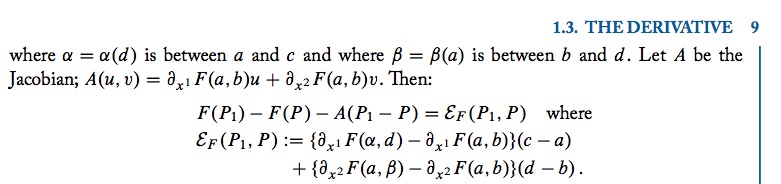 then it is fine.
then it is fine. pages 2, 37, 66, and 82 are in error (the correct pages can be downloaded from
pages 2, 37, 66, and 82 are in error (the correct pages can be downloaded from
 then it is fine.
then it is fine.